Do the criteria of success for a system of science themselves belong in the science category? Must you have a scientific judgment before you can judge a system of science?

No. Same with ethics. — Pfhorrest

You are right about science, of course, but wrong about ethics. Whether ethical principles are right or wrong is an ethical question - a redundant one, of course, which was my point. You can evaluate ethical systems by other criteria, but the most important criterion for ethical principles is their moral truth - and that judgement cannot be subordinated to philosophy or science or anything that is not ethics.

Bringing people out of existence through horrible disease is not part of the antinatalist agenda, sorry. Certainly being brought into existence exposes people all around the world to this though. — schopenhauer1

Which is why you should welcome the culling that I propose. I promise, it will be quick - not like dying from pneumonia. Or just kill yourself already and stop spamming the forum.

Say something or be silent. — schopenhauer1

I say we should start the virus containment program by culling antinatalists. OK, it's not the most effective means to combat the epidemic, but it's something. And best of all, it will be easily the most welcome virus-containment measure of all. Since antinatalists' maxim is that living perpetuates suffering, they will all be in favor of their own extinction - not to mention everyone else around them. It's pure win!

He is suggesting that the majority of Atheists are angry. — 3017amen

Well, O'Reilly is a professional bullshitter, so what he says is on him (and why are you watching this garbage anyway?) But if you really think that "the majority of atheists is angry," then you know nothing. Atheists are as heterogeneous a group as the population as a whole, and being angry is certainly not a distinctive trait of theirs. (Citing Einstein as an authority on this issue is clueless or disingenuous - he wasn't an authority on sociology of psychology, and he's been dead for more than half a century.)

And by what standard, pray tell, do you judge the reliability of your system of morality? — SophistiCat

Short answer: Same way I judge the reliability of science.

Long answer is about 80,000 words if you care to read it. You could start here for just the objectivity part or the last section of this for a general overview. — Pfhorrest

Yes, I understand that you have some pet utilitarian system, but this doesn't really answer the question. If my system of morality prescribes maximizing the amount of Chinese fortune cookies in the world, the reliability of this system qua moral system will not be judged by its own criteria of success, i.e. by the amount of Chinese fortune cookies.

This was a trick question, of course. The criteria of success for a system of ethics themselves belong in the ethical category. You have to have ethical judgment before you can judge a system of ethics. But if you already have ethical judgment, then what need is for a system?

Do not have children. Over and out. But I'll be back shortly. — schopenhauer1

Enough with the spamming.

Near as I can tell, you mean something like 'coordinate time' when using the phrase 'relativistic time'. — noAxioms

Time is an integral part of relativistic spacetime. If you need a specific measurement of time, you can use proper time in conjunction with a specific timelike worldline, or a coordinate time in conjunction with a specific reference frame - doesn't matter, since they are all mutually convertible.

Can there be time without clocks? — noAxioms

I mean "clocks" in a general sense, as any observable regular physical process, such as an oscillation.

Up to a point, yes. However, by its nature, secular morality allows for challenge. — Txastopher

The flexibility and openness to change of secular moral systems can vary as much as the flexibility of and openness of religious systems (which vary a good deal). But ultimately systems are only as open and as flexible as their users.

Even if every person where to think exactly the same about morality that wouldn't make morality objective. It's an objective fact that all people think the same in that case, but it's still something people think... so it doesn't get anymore 'subjective' than that. Words have meanings.

Morality is something we create, like language is, we do not observe or find morality or language like we find objective facts about the world. And so it doesn't make a whole lot of sense to use terms like true or correct or whatever equivalent. — ChatteringMonkey

Well, morality is what people think in the same way in which electric charge, say, is what particles have. Morality (and language) is no more and no less subjective than facts about the world - it is a fact about the world. I think this subjective/objective dichotomy isn't helpful at all.

So yeah, what philosophers have been trying to do in ethics over the ages, seems pure hubris to me, and doomed to fail. — ChatteringMonkey

Right on. I generally agree with what you say, except it seems like you overestimate the degree to which morality is a social contract. First of all, we need to distinguish social mores and individual moral character. The genesis of social moral codes can plausibly be analyzed that way, but there are probably other contributing causes. And when it comes to personal morality, its development will vary greatly case by case.

Certainty is still the quality lacking in secular morals. — Txastopher

Nah, there's no lack of moral certainty among secularists; you need to look a bit more into history and generally get out and meet more people. Being certain that you are right is more related to character than anything else.

Here's one right here:

A secular system of morality as reliable as the physical sciences is possible. — Pfhorrest

And by what standard, pray tell, do you judge the reliability of your system of morality?

Actually, you never really defined what you mean by 'relativistic time'. You say physical time is that which clocks measure. I think physics would say that a clock measures proper time, a frame independent property of any timelike worldline. — noAxioms

Sure. But as you note later, proper time can be well-defined even in the absence of anything to mark its passage, and that is where I see a problem. You could have a relativistic spacetime with nothing else, and formally it would still have time of every description. But would it be physically meaningful?

Of course, here we run into the more general question of the nature of the physical law. Are laws ontologically prior to things and events that are subject to them? Do they describe potentialities (like what would happen if that empty spacetime did have something in it to demonstrate its relativistic properties)? Or do laws serve only to connect the dots, describe the regularities in our observations? In the latter case - and only in the latter case - it would be unjustified to talk about time in the absence of anything that is time-like. I think I lean towards the Humean regularity view.

One might object that clock time, as instantiated by physical clocks (regular processes), is a naive concept and that relativistic time is more fundamental. But clocks are epistemically prior to our concept of time. The reason we came up with the theory of relativity in the first place was to give a better account of clock-based time (among other things). Without clocks what is the point of relativity?

An empty, flat spacetime with a positive cosmological constant is an interesting case. Formally, there is a temporal process (expansion of spacetime), but with nothing clock-like to register the passage of time, does it still make sense to talk about time? Does it even make sense to model it with a Lorentzian manifold and Einstein's field equations?

No.

The article states: "In fact, unless inflation went on for a truly infinite amount of time, or the Universe was born infinitely large, the Universe ought to be finite in extent." I admit I was only addressing the first possibility, but the second possibility remains just an assumption. — Relativist

As is the alternative. It is widely understood that the universe (or at least the inflationary "pocket" that we occupy, if we go with the still somewhat speculative eternal inflation hypothesis) was either always finite or always infinite. Neither option is granted the default status by cosmologists (your epistemological arguments notwithstanding). The question is decided by the weight of evidence, or lacking that, by considerations of simplicity; these two epistemological criteria constitute the foundation of scientific epistemology. As far as the former, there have been some publications that claimed that a closed (finite) spacetime topology fits some of the observational evidence better than the flat topology of the standard FLRW model, but their conclusions have not yet gained widespread acceptance. As for simplicity, here the flat semi-Euclidean spacetime wins over the more structurally complex closed topologies.

The first article showed that, according to accepted physics, spatial extension can only be infinite if there is an infinite past - that's why I focused on past time. — Relativist

If you mean this article, then it doesn't say that, and it would be pretty incredible if it did, because the best established cosmological model to date implies an infinitely extended universe without the assumption of an infinite past. Note that the article spends considerable time talking about inflation, which is less well established than standard Big Bang cosmology, but inflation doesn't imply what you claim either.

This does not apply to my claim. Sure, it's coherent - his statement entails no logical contradiction, but it circularly assumes the infinity exists, and it is that assumption that I challenge. — Relativist

There is no circularity, because the refutation does not seek to establish the positive claim and makes no assumptions of its own. It only shows that the anti-infinitist argument doesn't work - the specific argument that you were making originally involving "completeness." (Morriston makes a similar case in Must the Past have a Beginning?)

As for your first order/second order abstractions, that's something completely different. I won't go further than just to say that I am not buying your epistemological construction.

Physics will never be able to prove the past is infinite, all it can possibly do is to show that no past boundary of time has been found. — Relativist

I was talking about spatial extension. Simply put, if space is infinite, which seems plausible from what we know, and if the rest of it looks much like what we can see around us, which is very plausible, then there's your actual infinity (if by that you mean an infinite number of material objects).

My argument is in the spirit of David Conway’s, in that I utilize the concept of completeness. However, Smith’s refutation doesn’t apply to my argument. — Relativist

How can an infinity of days become completed? — Relativist

You do not so much utilize the concept of completeness as just plug it in and expect it to do the work for you. Try to unpack the reasoning and you will see that it either does not apply or it begs the question against the existence of actual infinities.

Smith does address the sort of argument that you are hinting at in his section VI:

the collection of events cannot add up to an infinite collection in a finite amount of time, but they do so add up in an infinite amount of time. And since it is coherent to suppose that in relation to any present an infinite amount of time has elapsed, it is also coherent to suppose that in relation to any present an infinite collection of past events has already been formed by successive addition. — Smith, Infinity and the Past

(And he goes on to address Conway's and Craig's arguments in that vein.)

I’m not making the bold claim that an infinite past is logically impossible, I simply claim that there’s no conceptual basis for considering it POSSIBLE, and therefore it’s more rational to reject it. — Relativist

I fail to see the distinction that you are trying to draw here.

Personally, I would not refer to time as measured by a clock as "physical time". I would call it "clock time" or some such. Time as measured by a clock is stochastic. It only follows from the second law of thermodynamics, which is not fundamental law. It doesn't even make an assertion that is true in all possible worlds that have the same fundamental law as ours. And that have a Bug Bang.

There is a possible world where my fair coin has always come up heads, even though I have tossed it a quadrillion times. Likewise, there is a possible world in which my pocket watch has always run backwards, without being broken in any way. — Douglas Alan

The second law of thermodynamics gives a preferred overall direction for time, but the rate of time is established by any number of regular physical processes, such as the vibrations of a cesium atom, which are used for the standard atomic clock. It is the availability of such physical processes - plus an overall direction - that gives us time as we normally understand it in physical sciences. (A direction is still necessary to provide an order to the cycles of a physical process - otherwise you can't really say that this cycle occurred earlier or later than that cycle.) We can also talk about subjective psychological time and other kinds of time - these I distinguish from what I call physical time (or clock time, if you prefer). The coordinate time of the Minkowski or the Lorentzian manifold is one of the species of time that, I argue, is not identical to this generalized concept of physical time.

The second law of thermodynamics may not be fundamental, in the sense that it is reducible to physics at a lower scale, but all you need for it to obtain is a non-equilibrium state - and then it becomes as inevitable as any fundamental law. Almost as inevitable. I take your point about it being statistical. But does this strike a blow against the idea that thermodynamics is essential to our understanding of time? I understand probability and statistics epistemically, so if you tell me that the probability of an outcome is 1 - 10^-45 (that's the probability of a quadrillion coin tosses not all coming up heads), that's probably better than the confidence level of all our fundamental physics experiments combined.

The fact that clocks are not reliable in all possible worlds, or after the heat death of the universe leads me to exactly the opposite conclusion: We should not be making any profound metaphysical conclusions at all based on clock time. We should stick to relativistic time. — Douglas Alan

I am with you, to a point: I am not big on metaphysics. I would rather confine myself to more modest phenomenological models.

Note that when you object to thermodynamics playing any part in the definition of time on the grounds that other possible worlds with the same fundamental laws may not exhibit such thermodynamic asymmetry as is observed in our universe, you are already deep into metaphysical theorizing, perhaps without even realizing it.

Two percent isn't low. I'd say flu's 0.1% is low. — Michael

Two percent is about the same mortality rate as the Spanish flu (I don't know where the 20% figure came from), which killed about 30 million people by the time it ended. The coronavirus has a similar infection rate as the Spanish flu. However, as has been quoted here, its mortality may be overestimated, and its infection rate may go down as well if we make the best effort to contain it. But the "nothing to worry about" attitude certainly isn't going to help in that.

And yet, if space is finite, it's contents are finite - which would entail some upper bound. Current physics indicates that space, and its contents, extends in space through a temporal process (described here). This means the extent can only be unbounded if the past is unbounded (which the article also states). — Relativist

The article says quite clearly, and more than once, that we don't know whether the universe is spatially finite or infinite. We can estimate a lower bound on its size, but not an upper bound. The simplest topology consistent with our observations at large scales is an infinite, flat space; this is what the most common current cosmological model posits (so-called FLRW model). However, there are also closed topologies that are consistent with the same observations.

As for your conceptual anti-infinitist argument, this is an old and surprisingly persistent confusion. Quentin Smith had a nice analysis of this and several other such arguments in a 1987 paper Infinity and the Past.

@Luke didn't you go over this with noAxioms and others for ages and ages on the old forum? That did you no good: you are still stuck on this idea that there is no motion under eternalism. Do we need to flog this dead horse for 20 more pages? Why don't you give it a rest and find something else to argue about?

It's been a long time since I studied special relativity (we spent several weeks on it in Physics 101), but IIRC, relativity doesn't provide a forward and reverse direction of time. It just provides two directions of time, and they are symmetric. — Douglas Alan

You remember right, but that wasn't at issue - we've both acknowledged that this is the case. My point is that relativistic time is not identical to physical time (the time that physical clocks measure). There are at least two reasons to think so: First, it is an idealization that wasn't designed to perfectly track physical time. Second, we have specific examples - probable or at least possible - where relativistic time diverges from physical time.

Why is this important? To bring this back to the original topic, a common argument for the B-theory and against the A-theory is that the theory of relativity, though it may not rule out the A-theory, does not offer any support for it either. The A-theory requires additional assumptions that are not part of SR or GR. The implicit thesis here is that we ought to base our theory of time on the theory of relativity and nothing else. But this thesis is weakened if the identity between relativistic time and physical time is weakened.

How about instead of petulantly demanding answers to stupid questions you use your own head? You demand to know why a device like a monitor, camera or book can only store a limited amount of information. Did you already forget that this was the very premise of your stupid argument?

Ugh, why do I even waste my time on this...

I don't see the problem. There are two directions of time. Neither of these directions of time ever changes. The only thing that might change is which direction of time is pointing towards greater entropy could change. But the direction is not changing. Only what is in that direction might change. — Douglas Alan

The problem that I see here is that there is no inherent connection between the direction of time given by the time coordinate of the relativistic spacetime and the direction of time from the entropy gradient (which in turn is a proxy for physical time). Even if it so happens that in our universe these two never diverge, when you make a commitment to relativistic time, you are opening yourself to this contingency where your theory may diverge from reality.

And in case of heat death, which is currently taken to be the most likely future of our universe, this is more than just a contingency. If the prognosis is right, then eventually relativistic time will diverge from physical time.

So as a proxy for physical time, relativistic time is fine, most of the time, but it should not be taken to be identical with physical time, on pain of paradoxes.

You are not addressing the problem. What part of the universe you could not potentialy see on your monitor? You can either name what kind of object or information it is that your monitor can not visually convey, or you have to admit your monitor can convey any and every possible information. — Zelebg

If your monitor - or, say, any device or method for identifying distinct objects - can only register a limited number of objects, due to the way in which it is constructed, and you have registered that many objects, then all that you can say is that there exist at least that many distinct objects. This is the point that you fail to grasp.

Suppose, for example, that your device can register only up to five distinct things, and suppose that the world has more than five distinct things. What conclusion do you draw from this: too bad for your device or too bad for the world?

This idea, that your device or method can bias, limit or even fully determine what what you can observe is known as observation selection bias or observation selection effect.

The question is whether this encyclopedia of everything has infinite number of pages or not. The answer is no, because there is no reason why your monitor could not display any of those pages, and the number of pages your monitor can display is finite. — Zelebg

Perhaps you will realize your mistake if you reduce the size of the page to the extreme (although a similar exercise with reducing the number of pixels on the monitor failed to convince you). If you only have one character on the page, and there are, say, 100 letters, digits and other signs that you can depict with one character, does this mean that there cannot be more than 100 distinct entities in the world?

Faulty hypothesis — jgill

Well, no, the number of distinct digital photos of a given resolution is finite. But so what? He might as well have said: I am going to count the number of distinct things in the world using the fingers on my right hand. Let's see... Tree, house, shoe, smartphone... oops! Only one finger left - I better lump everything else into one remaining thing. There! The number of distinct things in the world is five!

What do you make of it? — Zelebg

Well, first it is unclear what it is that you claim to have proven. The question you claim to answer is "How many distinct things can there be in the world?" But the answer will depend not only on how the world is, but also on how you cut it at its joints, so to speak: what kind of things are you looking for? Distinct geometrical shapes? Distinct species of animals? Distinct entities posited by fundamental physics? The answer to your question will depend on the chosen mereology, and here the problem is that a priori, without knowing anything about the world, we can hardly even decide on an appropriate mereology. And even if we do know something about the world, our idea of what the proper mereology ought to be can change as we learn more about it. On the other hand, with a trivial choice of mereology (e.g. "Everything is either Donald Trump or not Donald Trump") we can get a pretty good answer without doing any work at all.

But the most glaring problem with your approach is that the answer to your inquiry is completely depended on your instrument of choice. If your instrument cannot register something, then that thing does not exist. If it cannot distinguish between two things, then they are the same thing. The result therefore is bogus: it tells you nothing about how the world really is, it just tells you about the limitations of your method.

I’m not sure if you are saying there is a problem with my argument or not. If there is, point to which statement of mine is supposed to be unwarranted. — Zelebg

Doesn't it bother you that the number of distinct things in the universe is limited by an arbitrarily chosen resolution of your camera? With an 800x600 24-bit pixel camera you can register at most ~8*10¹² distinct things. If instead you used an 800x600 16-bit pixel you would register at most ~3*10¹⁰ distinct things. And with a 1x1 1-bit pixel camera you would register only 2 distinct things. Haw can this be?

Alas, I'm not sure where the confusion is arising. If you believe that GR entails eternalism, the forward direction of time is given straight-forwardly by the direction of increasing entropy. (Modulo situations in which there is no such clear direction, such as post-heat death of the universe. But since there won't be philosophers existing then to worry about the problem or to experience what it is like to live in this time, this would seem to be moot to an eternalist.) — Douglas Alan

GR doesn't know anything about entropy - it's not part of the theory. For those who base their theory of time exclusively on GR - call them "relativity fundamentalists" - entropy is a stolen concept. They have to limit themselves to GR's coordinate time, which increases monotonically (in simply connected topologies), but in an arbitrarily chosen direction. Therefore, if it is possible for the entropy gradient to reverse itself along the length of one worldline, then a relativity fundamentalist faces a problem, because her theory of time is not sensitive to this change. Either she has to add something extra to the theory (which, incidentally, is what presentists do as well and for which they are criticized by fundamentalists), or she has to bite the bullet and say that the direction of time given by the entropy gradient can sometimes be wrong. (And then when is it right - and why?)

In the less contentious heat death scenario physical time effectively disappears, since there are no physical clocks to mark its passage, but the coordinate time continues - another contradiction that can be solved by acknowledging that mathematical time is not identical with physical time.

I don't understand what your hangup is. "Static" is an unfortunate choice of a word in Miller's article, because it normally means that either there is no change over time or that time is not in the consideration, and neither of these common meanings are relevant here. But Miller says exactly what she means by the Static Thesis: it refers to the B-theory of time, according to which there is no objective partition of time into present, past and future; present, past and future are relational terms. But there is still time in the eternalist's account, just as in the presentist's!

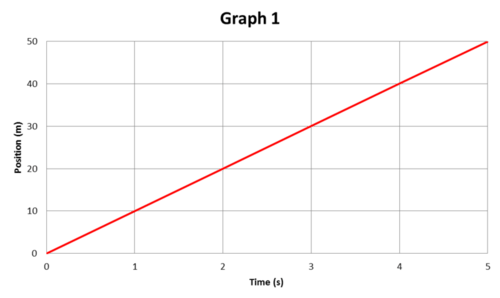
Here is a simple space-time diagram:



Both the presentist and the eternalist agree that the diagram depicts movement of a body through space. But the presentist adds that there is a fact of the matter about where on the diagram the body is right now, while the eternalist says that there is no such fact of the matter (not without reference to a specific observer). Nevertheless, the eternalist will agree with the presentist that the body is located at 10 m from the coordinate center at time 1 s and at 20 m at time 2 s, and that the difference constitutes a change in the body's position.

The argument is simply that relativity of simultaneity isn't sufficient by itself to imply a block universe. An additional premise is required, which is that all events to the past of any observer's surface of simultaneity are fixed and certain. — Andrew M

But that would not be sufficient for a block universe either. Unless observers are spread throughout the entire spacetime, their past light cones sweep only part of it, resulting in a moving block. Which is why he adds the stipulation of all observers - meaning, apparently, the entire spacetime block. Which, of course, assumes the conclusion.

And in any case, whether the argument is for a moving block or for the full block, nowhere does relativity do any work here. All you need, according to this argument, is the assumption that there are some observers for whom the past is fixed.

There is a significant difference between full color, grayscale, and a picture that can only show black / white pixels. It doesn’t follow ‘there are only two distinct things in the universe’ from the ability to ‘encode all the information in the universe with a digital dataset’. You are obfuscating delimiters between different binary strings encoding many different things. What follows is just that any information can be encoded with a minimal set of only two bits, nothing more follows. — Zelebg

Yeah, that was the point of the reductio. One black/white pixel can encode one bit. 800x600 24-bit pixels can encode 11.5 million bits. But that's just a quantitative difference that doesn't bear on the problem with your argument.

This is more evidence for eternalism if you ask me. E.g., why is it that "metaphysical time" just happens to agree with the arrow of time placed by the direction of increased entropy? What a fortunate coincidence, since it would be a crazy world otherwise.

For eternalism, there is no problem here.

On the other hand, I guess presentists could just say that the world had a 50/50 chance, and fortunately, it came up heads. — Douglas Alan

Not sure what distinction you are drawing here. Are you suggesting that, unlike the presentist, for the eternalist the nature of space and time contains nothing over and above that which follows from the theory of relativity? (And so for the eternalist there is no such thing as the arrow of time.) I am not so sure. At least I don't see why the eternalist has to be so exclusively committed to the relativistic spacetime.

But yeah, the arrow of time is another can of worms.

Oh hey! I didn't realize that you have joined this form. You've been missed :)

I think you're just saying that relativity doesn't entail an arrow of time, nor is it dependent on there being one. Nevertheless, relativity is consistent with there being an arrow of time. Relativity is not a theory of everything. — Relativist

I agree, and I think that this is an underappreciated point. Note that relativity is compatible with absolute simultaneity as well* - it just doesn't require or entail or suggest one. Just as the arrow of time would be an extra structure not licensed by the theory, so would simultaneity.

Does the fact that a feature is not required by a theory, even such a universal and well-supported theory as the Theory of Relativity, imply that it is not a real feature of the world? Not necessarily. It depends on whether the theory was constructed and tested in a way that would be sensitive to the existence of this feature. Since Relativity is agnostic about simultaneity, it doesn't give us an unambiguous answer. But this does put the pressure on the A-theorist to supply a justification.

* Time loops that are possible in GR make global simultaneity problematic. But first, we don't know if they actually exist - all we know is that they are compatible with GR. And more importantly, I don't think that global A-properties should be a requirement for A theories; local properties should be enough, given the phenomenology to which their proponents appeal.

So, taking your "proof" to its logical conclusion, if you could encode all the information in the universe with a digital dataset - that is to say, a sequence of zeros and ones - then all you would end up with would be a bunch of zeros and ones. And since any zero or one is just like any other zero or one, it follows that there are only two distinct things in the universe, which repeat many times over. Brilliant!

When you put a provocative question in the title of the thread, most people are just going to respond to the title without bothering to read further. I learned this the hard way. Well, most people won't bother reading further than the title anyway, but at least if you don't bait them you might get fewer useless replies.

As always, it's impossible to tell when someone claims to represent opponents' arguments made elsewhere, whether they are doing it accurately. Anyway, the arguments as presented sound pretty confused, which doesn't inspire one to follow their refutation, especially since the refutation is pretty confused as well.

For example, after extensive paraphrasing, the blogger ends up with the following thesis:

(1) Relativity of simultaneity + all observers’ 3D worlds are real at every event = block universe

I take it from the context that "observers" here are not meant literally, but rather as virtual probes that could potentially be dropped anywhere within the spacetime block. Oh, wait. Well, he does go on to state the obvious: that the second premise already presupposes the conclusion. But then for some reason he backtracks and adds that the first premise is needed as well, although he doesn't explain why.

I love SEP, and I certainly wouldn't mind more of a good thing, but is it really a model for what Internet ought to be? It is a pretty small step from a print academic encyclopedia, basically conforming to the Internet ideal circa early 90s (when SEP was conceived). Plus what said.

My favorite article? I can't be bothered reviewing and comparing the articles that I have read so far, but here is some random hotness: Chance versus Randomness. Comprehensive, comprehensible, authoritative, authorial, and up-to-date: "First published Wed Aug 18, 2010; substantive revision Thu Feb 8, 2018" (just as the OP article states, revisions are done in four-year cycles).

The article, instead of providing anything that might actually give me some insight into the answer, just provides a lot of history. Okay, history can be interesting. But I'd prefer actual insight to a long exposition on many failed attempts. — Douglas Alan

I don't think you understand the concept of a modern encyclopedia. Encyclopedia is not for publishing original research or stating one's own opinions (though opinion cannot - and should not - be completely eschewed even when attempting an objective survey). The objective of an encyclopedia like the SEP is to give you the lay of the land - both in its historical and its contemporary dimensions. Where to go from there is your choice. Drill down the references that seem most promising - or set out on your own if none of them are. With the background knowledge, at least you will not work in ignorance and isolation.

I did some skimming of the references you provided that were actually available to me. One thing I noted that was of interest is that in a closed timelike curve, there can be no consistent entropy gradient that gives time its forward arrow. If there were such a gradient, you couldn't return in the loop to the same point in space-time, since that point must have the same entropy, and consequently it wouldn't be a closed curve. It seems that this would make traveling around one unpleasant. But, I suppose if it were large enough, you could refrain from going all the way around the loop, and I suppose there could be a gradient that does not change direction in the part that you stay on. Well, this is probably neither here nor there for this discussion, but I found it an interesting worry. — Douglas Alan

This also brings up another relevant question: does the coordinate time of a mathematical model (in this case the theory of relativity), physical time (time that is measured by physical clocks) and metaphysical time always coincide? I am more than a little skeptical of the metaphysical time (as distinct from the other two), although some A-theorists find themselves more-or-less forced to assume it in order to rescue the hypothesis. But as for mathematical vs. physical time, hypothetical time loops in GR spacetime may be one of the instances where they pull apart. And they highlight a problem with relativity as a model of time: relativity does not have a concept of the arrow of time. Sure, the coordinate time has two opposite directions, but there is no fundamental distinction between them; the designations of "future" and "past" are purely conventional in the model. If what you are saying about closed timelike curves is correct, it appears that even as the physical time reverses its flow, the coordinate time does not notice the fact, merrily ticking along. (Another interesting question is what happens after the heat death or, in some models, at the Big Bang, where physical time may effectively disappear, even as coordinate time goes on. But that is a discussion for another time, as it were.)

Relativity does have the concept of the passage of time, and notably it admits differential rates of time, when the duration of proper time is shorter along some future-directed worldlines than others, as in the twins paradox - in effect giving us future time travel. The way we, as empiricists, can know that this really does capture at least some aspects of the flow of time is that this is something that has observable physical consequences: the differential rates of time's passage can actually be marked and recorded by physical clocks in properly conducted experiments. But the question remains: are there limits to the fidelity of this model when it comes to describing time?

I am not yet sure what to make of past time travel. Not surprisingly, there is plenty of literature on this issue as well, including discussions of causality paradoxes (see for instance SEP articles on time, or Kutach's article in A Companion to the Philosophy of Time, or Smeenk and Wüthrich's article in The Oxford Handbook of Philosophy of Time). But I think I'll leave this can of worms for another time...

In what sense do virtual or real particles live outside the mathematical equations? — MathematicalPhysicist

We get a sense that some theoretical entity is more than a mathematical contrivance if it does not go away when we change the model, i.e. if we find it indispensable. Our sense of the reality of the thing also strengthens when we find more independent ways of probing it with empirical tests. However, these criteria are not so solid and there is room for much ambiguity in edge cases. Physicists do not usually obsess over questions of whether something is really real or somewhat real or not quite real.

You seem to agree that we must of necessity occupy some very special place in the universe (a habitable place in a universe that is nearly everywhere uninhabitable), but then plow on with your pet theory anyway. But if we were living in a simulation, what would that have changed? In the simulated universe (even if it is only a small, generic chunk of it that is being simulated) we would be just as lonely. And the Rare Earth hypothesis would have the exact same status in a simulated universe as in a real one, because of course what is true of an actual thing must also be true of its simulation.

The debate around Rare Earth hypothesis has nothing to do with us being "special" (whatever that means) - it is simply about how common inhabitable planets are in the universe, which is an empirical, scientific question (even if we are not in a good position to answer it now or possibly ever).