Comments
-
The Christian narrative
So it seems you have gone with adding the premise: "classical theologians are wrong about what they think they are saying, and have been wrong since the Patristic era, because when they use "is" it must refer to numerical identity."
But I've already mentioned the response here. They would claim that this is absurd, since God's unity is a prerequisite for there to be number, and multitude is, by definition, a property of the limited and finite.
So what is it that is similar? If there is no relation, how is there a similarity?
For between creator and creature there can be noted no similarity so great that a greater dissimilarity cannot be seen between them.
— Fourth Lateran Council, 1215
That's the basic idea of the Analogia Entis.
Presuming we read "Mark is human" and "Christ is human" as that Mark and Christ participate in a common nature, then we are not here talking about identity. That is, you have moved from identity to predication. If we were to follow that, you would end up with Christ and The Holy Spirit merely participating in godhood in the way that Mark, Christ and Tim participate in being human. You would have three gods, not one. Your conclusion would be polytheistic.
What individuates particulars that share a nature and formal identity? That's an important question here. It's also a wholly metaphysical question.
Participation is a metaphysical notion as well. Creatures participate, God is what is participated in. -
The Christian narrative
Any work on Peirce that covers his studies should do. It's not an ancillary fact, but central to his whole project. I thought Realism and Individualism: Charles S. Peirce and the Threat of Modern Nominalism by Oleskey was good, but the great popularizer of Peirce, John Deely has the compact Red Book as well. -
The Christian narrative
It's "one nature, three persons." Consider the analogous case of human nature:
Mark is human. (A is B)
Christ is human. (C is B)
Therefore Mark is Christ. (A is C)
This is obviously false. Leaving out that all predication vis-á-vis God is analogical, you would still need to assume a properly metaphysical premise like:
"More than one person cannot subsist in the same nature."
Traditionally, in "the Holy Spirit is God," "is God" refers to the Divine Nature. I suppose another premise that would work is: "'is God' must refer to univocal, numerical identity." However, this is exactly what is denied. As noted earlier, numerical identity is taken to be posterior to (dependent on) God, the transcendental property of unity, and measure. Numerical identity is a creaturely concept. From earlier:
Right, numerical identity (dimensive quantity) is posterior to virtual quantity (qualitative intensity) and anything's being any thing at all. Unit (and thus number, as multitude) is posterior to measure. Which is just to say that, to have "three ducks" requires "duck" as a measure, etc. God's unity is transcendental however, in the sense that all being is unified. "Thing" and "something" are also considered derivative transcendentals (in the same way beauty is). They are prior to numerical identity in that you cannot have "numbers of things" without things; multitude presupposes units. The supposition here is that numbers exist precisely where there are numbers of things, hence their posteriority, although they are prior as an absolute unity in God (normally attributed to Logos).
Part of the idea of their pre-existence God is that all effects exist in their causes. But it's also the case that no finite idea is wholly intelligible on its own (just as multitude is not intelligible without unit). Hegel's Logic is largely extending this idea. Only the "true infinite" can be its own ground.
I have not personally seen many theologians reaching for non-classical logics, particularly not Thomists. They generally take this sort of objection to result from a failure to make proper distinctions, often paired with a question begging assumption of the univocity of being.
And again, the overarching observation that the task folk here set for themselves is not to see where the logic goes, but to invent a logic that supports the Christian narrative.
Historically, the Analogia Entis has its origins in Aristotle, with the study of logic. Nominalism and the assumption of univocity are a much later, theologically motivated rejection of analogy (one that emerged in a somewhat similar fashion in Islam a few centuries earlier). In general, the historical approach of the nominalists and the key reformers they influenced was indeed to say that the Trinity is simply affirmed through faith alone, even if it is seemingly contradictory under the assumption of univocity. By contrast, the classical view would be something like: "the Trinity is beyond human reason but not contrary to it."
Personally, I find this sadly ironic. The main concern of the nominalists was that somehow natures "limited God," making God less fully sovereign and powerful (so too for creatures' possession of any true freedom). Yet, their innovations simply reduce God to "the most powerful being among many," very strong, but just a being like any other. Infinite being comes to sit on a porphyrian tree next to finite being; God sits to the side of the world instead of being fully transcendent ("within everything but contained by nothing"). God becomes incapable of granting creatures true freedom or causality without Himself somehow losing a share of these. God becomes the "divine watchmaker," exercising a wholly extrinsic ordering upon being, as opposed to being the generator of an intrinsic ordering bound up in "natural appetites" (ultimately a "Great Chain of Love" emanating outwards in analogical refractions from the angels down to non-living elements). It is, in many ways, a greatly reduced vision of God. -
Virtues and Good Manners
But that's just your own desire for, not peace or goodness, but preservation of all that you've become accustomed to.
You made the point better than I could. There is the thorny issue here of identifying virtue. Classically conceived, the virtues should be as beneficial for the poor man as the rich woman, etc. Manners, in being structured by the current social order (which may or may not be virtuous), can be more or less aligned to virtue.
Actually, in theory the virtues should be most beneficial for those beset by bad fortune. Good fortune can lift anyone up, to at least some degree. Whereas the idea is that virtue allows people to flourish even under dire situations. The idea being that it is better, at least everything else equal, to be temperate instead of gluttonous, prudent instead of rash, courageous instead of cowardly, or, in terms of "physical virtue," strong instead of weak, skilled instead of unskilled, etc.
But, we might wonder how well this idea "cashes out" in the modern context. -
Language of philosophy. The problem of understanding being
Let’s talk about identity. What is the role of time for you in the determination of identity? In my way of thinking, identity requires temporal repetition.
I guess I disagree here, for the aforementioned reason that I don't think it makes sense to talk about time in the first place unless something is already the same across the sequence. If there isn't already sameness, you just have wholly discrete being(s). The very ability to notice difference, for it to be conceptually present, requires that there also be sameness.
That was, I took it, Eddigton's point about Kant and Hume that I shared, and I think it's a good one. It seems to me that a phenomenological approach that assumes that temporal experience is prior to sameness/identity, is in fact, already presupposing a certain sort of sameness and identity that is prior to temporality itself. To use a metaphor, it's assuming that all the frames on the "reel of experience" are already part of the same reel, such that one can "play it forward." Whereas, if we drop this assumption, we would be forced to get rid of the film reel and we would instead have a bunch of wholly isolated frames, detached from one another (no prior similarity).
But, as noted earlier, I also think it is easy here to pass between conceptual priority and priority in the order of experience, and ontological priority. A wholly phenomenological argument against something like the "block universe," (which assumes that the block universe is equal with itself) that relies on assuming that the order of experience just is the order of ontological priority, seems rather shakey. -
The Christian narrative
may be that Pierce read Augustine, but the notion that his philosophy should be understood in the context of Christian theology is incorrect.
To be clear, I haven't suggested anything of the sort. What I said is that there is a long history of theologians looking at the cosmos as a revelation of the divine nature (the "book of nature;" Romans 1:20) and that this can be extended to Pierce's discovery of the triadic nature of relations. If a triadic structure is taken to be essential for a meaningful cosmos, this can be used for a transcendental argument vis-á-vis the threeness of God. -
The Christian narrative
We'd have to clarify that. "One thing with three faces" (or masks) is a common formulation of the modalist heresy. Granted that the distinction from one ousia three hypostases is subtle. It might not really be that relevant here though because the idea isn't that the sign relation, nor any of the other triads, are perfect models of the Trinity. In the case of being/knowing/willing it is also the case that each are distinct (and indeed, the persons of the Trinity are distinct, the Father is not the Son; denying this leads to the patripassianism hersey.)
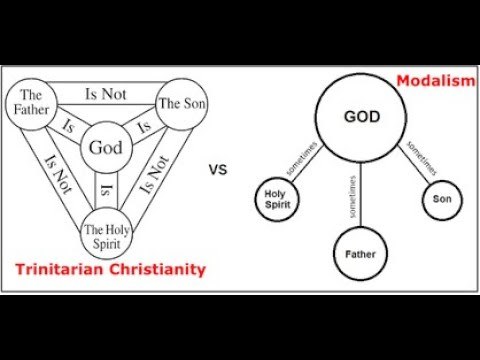
Augustine is more interested in different triads in his later work, such as the being/knowing/willing of the Confessions, or lover/beloved/love (he has many). Saint Bonaventure also looks at a plurality of triads—being and mind are refracted through analogy like a kaleidoscope, in many different ways.
Anyhow, as John Deely never gets tried of repeating, the sign relation is "irreducibly triadic." It is defined relationally, just as the Trinity is. A sign isn't an assemblage of parts, since each component only is what it is in virtue of its relation to the whole. The sign and the Trinity aren't perfect images of each other, the idea is rather that all of creation reflects the Creator, and thus the triadic similarity shows up even in the deepest structures, yet no finite relations can capture the Trinity. -
Virtues and Good Manners
Not long ago I happened to come across a very short clip where John Milbank was contrasting culture with manners. Being 44 seconds long, it's not particularly deep, but I think I get the basic idea from his other work, which is that a code (i.e., largely procedural) of civility, a step below legalization, actually supplants notions of virtue. Or more accurately perhaps, the elevation of procedural rights grounded in the autonomous agent over any notion of the human good means that rules as a sort of social lubricant to avoid friction between individuals replaces notions of virtue as the harmonious internal and external ordering of the person, within themselves, but also as they are ordered to the world and their society.
Manners and virtue obviously aren't in conflict per se. But manners might become seen as "fake," "inauthentic," and arbitrary when detached from virtue? Isn't that sort of the idea with the "phonies" in The Catcher in the Rye or the ticky tacky people of Malvina Reynolds' Little Boxes, or the Beat writers, etc.?
Well, at least one random person on Reddit agrees with this judgement. I quickly found:
I think that some people are using it as a way to act special and above people for no reason and to radiate the holier than thou energy to other people. They have no reason to use it other than the fact to act better than people and to get more attention Than they deserve. No one is gonna care about what side you put your forks or spoon on just bc it’s “bad manners” or “rude” they just use those words to describe how they don’t like it.
To hide behind their uncomfortabilities in life. Nothing is really rude anymore bc ppl use it as a way to hide behind things they don’t like. If I don’t wave at the person who let me cross how is that rude?? It’s petty/little shit like that that people think is rude and pisses me off, no one cares anymore what you do.
lol, they certainly aren't afraid of putting it in stark terms.
Patrick Deneen argues that the liberation from custom favors the "elite." I am not so sure about this. I think he may be conflating "most well off or flourishing," with "has the most wealth and power," here in a pernicious way. But it's a relevant and interesting analysis:
Custom may have once served a purpose, Mill acknowledges—in an earlier age, when “men of strong bodies or minds” might flout “the social principle,” it was necessary for “law and discipline, like the Popes struggling against the Emperors, [to] assert a power over the whole man, claiming to control all his life in order to control his character.”9 But custom had come to dominate too extensively; and that “which threatens human nature is not the excess, but the deficiency, of personal impulses and preferences.”10 The unleashing of spontaneous, creative, unpredictable, unconventional, often offensive forms of individuality was Mill’s goal. Extraordinary individuals—the most educated, the most creative, the most adventurous, even the most powerful—freed from the rule of custom, might transform society.
“Persons of genius,” Mill acknowledges, “are always likely to be a small minority”; yet such people, who are “more individual than any other people,” less capable of “fitting themselves, without hurtful compression, into any of the small number of moulds which society provides,” require “an atmosphere of freedom.”11 Society must be remade for the benefit of this small, but in Mill’s view vital, number. A society based on custom constrained individuality, and those who craved most to be liberated from its shackles were not “ordinary” people but people who thrived on breaking out of the customs that otherwise governed society. Mill called for a society premised around “experiments in living”: society as test tube for the sake of geniuses who are “more individual.”
We live today in the world Mill proposed. Everywhere, at every moment, we are to engage in experiments in living. Custom has been routed: much of what today passes for culture—with or without the adjective “popular”—consists of mocking sarcasm and irony. Late night television is the special sanctuary of this liturgy. Society has been transformed along Millian lines in which especially those regarded as judgmental are to be special objects of scorn, in the name of nonjudgmentalism. Mill understood better than contemporary Millians that this would require the “best” to dominate the “ordinary.” The rejection of custom demanded that society’s most “advanced” elements have greater political representation. For Mill, this would be achieved through an unequal distribution of voting rights...
Society today has been organized around the Millian principle that “everything is allowed,” at least so long as it does not result in measurable (mainly physical) harm. It is a society organized for the benefit of the strong, as Mill recognized. By contrast, a Burkean society is organized for the benefit of the ordinary—the majority who benefit from societal norms that the strong and the ordinary alike are expected to follow. A society can be shaped for the benefit of most people by emphasizing mainly informal norms and customs that secure the path to flourishing for most human beings; or it can be shaped for the benefit of the extraordinary and powerful by liberating all from the constraint of custom.
Aristotle also has a relevant section in Book IX of the Ethics where he talks about how forms of government affect friendship. So, in the corrupted forms of government, you see different corrosive effects on true friendship. Tyranny leads to friendships based on fear and flattery. Oligarchy leads to friendships based on jealousy and advantage. Democracy (by which means a sort of mob rule) tends towards a sort of false equality and refusal to recognize distinctions in virtue. By contrast, the constitutional polity sets and equal ground for friendship, good will (the ground of manners), and concord (joint striving towards a common good). This rings true for some first hand accounts of Soviet life I've read at least.
An interesting idea here is that true friendship, the willing of the good for the other for their own sake, requires virtue as a prerequisite, since, without virtue, we cannot even consistently will the good for ourselves. I think "well-rounded" are harmonious are the right ideas here. -
Language of philosophy. The problem of understanding being
I don’t know of any philosopher who advocates becoming as ‘sheer’ change devoid of relationality. For Deleuze it is in the nature of differences that they always produce themselves within and as assemblages, collectives. The relative stability of these multiplicities does not oppose itself to change but evinces continual change within itself that remakes the whole in such a way that the whole remains consistent without ever being self-identical.
Exactly, which requires sameness and identity. Hence, the principles being equal (or even co-constituting, at least in the order of conception), or even three: actuality, potency, and privation. -
The Christian narrative
However Peirce's semiotics (sign-object-interpretant) was developed in a completely different intellectual context from Trinitarian theology.
This is factually incorrect. Charles Sanders Peirce's theory of signs is based explicitly on his study of scholastic theories of signs that were developed originally by Saint Augustine. The basic triadic structure is not new to Peirce, but is first put forth in De Dialectica, one of Augustine's early works. He explicitly relates it to the Trinity in later works, e.g. De Doctrina and De Trinitate. Peirce considered himself a "Scholastic-realist," although how well that term applies to his later thought is a topic of debate. -
The End of Woke
First, I'll just point out that I think it's a mistake to conflate "emancipatory" with "critical theory" and "definitely not post-modern." Even in less explicitly activist texts, the "free rollicking of thought," the opening of "new lines of thought," or the deconstruction of systems so that new ways of thought and action can come into being are often presented as desirable in themselves. The very word, "freedom"—"libertas" and all its cognates—is value loaded in its Western context, and that certainly doesn't seem to be different even in more theoretical texts. A freedom that is associated with potency, and the generation of greater potentiality vis-á-vis thought becomes itself emancipatory. But Woke has also drank deep from this conception of emancipation as the freedom (as lack of constraint) of thought and action (which is of course part of liberalism as well, although in a different register). This is one of the key points on which it diverges from the older Christian, Islamic, and Marxist activism of the 20th century.
To my eye, this looks like an example of @Leontiskos'
"putting second things first," however. The freedom of thought, an increase in potentialities available, of lines of action and thought, are themselves only good as a means of reaching choiceworthy ends, better means to those ends, etc. Greater potentiality is, of itself, not actually emancipatory nor is it desirable. Taken wholly by itself as an end, it's a slide towards multiplicity and nothingness. It's only choiceworthy itself if it is "unblocking" changes that are actually improvements. This is the old liberal inversion of placing a procedural freedom above the good, which is accomplished when it considers potency a good in itself.
They are relativist to a point.
Yes, it varies, but they do seem to tend towards various forms of anti-realism as well, including historical anti-realism. I think you are selling short the level of commitment here. One of the things right-wing media made the most hay over was straightforward pronouncements of the relativism and anti-realism coming out of activist circles.
Statues, names on buildings, memorials, etc. become such a focus because history can very much be re-written by the current victors, and while this is sometimes framed as merely "uncovering the truth about the past," it is also sometimes framed more explicitly simply in terms of power dynamics ("he who controls the present controls the past"). It is often "power all the way down," when the adversary controls it, and something to be uncovered when the forces of virtue control it. But the apparent contradiction here, or supposition of a "real" bedrock realism, is in fact also consistent with the "power all the way down" narrative. Those welding "power that goes all the way down," are not obligated to frame it as such, and indeed it would be unwise for them to do so.
My general impression is that, broadly speaking, the median Woke position is simply contradictory. It is morally and epistemically anti-realist and strongly relativistic, while at the same time being absolutist. This is, in many cases, an unresolved, and perhaps often unacknowledged contradiction. But my point would be that, accepting some of their starting points, contradiction is actually not a fatal problem. One can dismiss the demand for contradiction-free reasoning as nothing more than a power move (and indeed this has been done). You can see this more in the right-wing analogs of Woke (which are often conscious responses to it), which are even more explicitly relativistic and anti-realist, following a logic that terminates in something like "might makes right," where "might" has been given a much wider theoretical understanding than mere physical strength or kinetic force.
Don’t confuse flows and concatenations with value-free causal bits. These flows are anything but value-neutral. And they are anything but motive and purpose-neutral.
Sure, although I would say the aesthetics of such an interpretation have already begun to deflate our fellow "concatenations." But my point was more about adopting a study of them that attempts to bracket out or withhold all moral judgement. This seems to me to be, by definition, a deflation vis-a-vis value.
The fact that we are concatenations and flows of values and desire means that no one can stand outside of some stance or other to judge from on high, including the philosopher who writes about such flows. They are not a neutral observer but are writing always from within context , within history, within perspective. There is no perspective which doesn’t already have a stake in what matters and how it matters, but this doesn’t prevent one from talking about it from within one’s relation of care and relevance to the world.
Right, but then this is also taken as a reason for bracketing out or eschewing moral judgement. It's that line of reasoning I find faulty. Consider that Socrates does not need to "step outside his humanity" to judge, universally, that "all men are mortal." He can do this just fine while remaining a man.
The idea is not that the wise man steps outside the world to stand alongside a Good that also lies outside the world. The Good is everywhere, in all things. Rather, there is merely the concession that it is possible for some to be wiser than others. -
Language of philosophy. The problem of understanding being
It's a good question. Freedom and power were traditionally understood in terms of actuality, potency itself being nothing, and so inherently most static in that it is wholly incapable of moving itself. There is often a reversal here though. Potency becomes least static, freedom becomes the potential to do or be anything. Yet this only makes sense if potency is in some way actual, if it can spontaneously actualize itself, e.g., explanations of our contingent reality as simply 'brute face,' or of reality as primarily, of fundamentally will, a sort of sheer willing.
Well interestingly, Aristotle and Aquinas following him in On the Principles of Nature thinks we need three principles, not a reduction to one, or a dichotomy (e.g., difference and sameness). Those are:
Matter/potency
Form/actuality
Privation
Absence is in there, and it must be if finality necessarily lies outside whatever is moving. -
The Christian narrative
It comes out of the Doctrina Signorum, the understanding of signs laid out originally by Saint Augustine, which predominates across the Scholastic era and was the main inspiration for C.S. Peirce's semiotics and his essential categories of Firstness, Secondness, and Thirdness.
Basically, any relation that can mean anything at all involves three things:
-An object that is known (the Father)
-The sign vehicle by which it is known (the Word/Logos, Son)
-The interpretant who knows (the Holy Spirit)
And you can trace these to the two creation stories in Genesis, where the first is God's Word speaking things into existence (often taken as their forms, e.g., by Rashi), and a second where God shapes things out of dust and then breaths His Spirit into them. God the Father is, in a sense, always "what is known," since He is the First Principle, whereas all intelligibility comes through the Logos, and the sharing in God's Spirit is necessary for experience.
If this triadic structure is required for any meaning (or in terms of physics, any information) then there is a transcendental argument for the necessity of the triadic relationship for anything to mean anything at all (to anyone), and if "the same is for thinking as for being," this holds for being as well. Now, science often tries to view things a dyads, but it does this with simplifying assumptions and by attempting to abstract the observer out of the picture. There ends up being problems here for all sorts of things (e.g., entropy, information, etc.), but more to the point, true dyadic relationships don't seem to appear anywhere in nature. Everything is mediated. We can think of two billiard balls hitting each other as dyadic, but if we look close enough the description involves all sorts of mediation. When we get to the smallest scales, we get entanglement instead of dyadic interaction, which, according to some physicists, is inherently triadic (Rovelli likes to say "entanglement is a dance for three," the point being that quantum states are always relative to an observer or system).
So, this can be taken as the structure of the Trinity mirrored in creation. Likewise, in De Trinitate, Augustine charts the inherently triadic organization of mind in detail too. That's a hazy outline, but the basic idea. The idea that everything is mediated also goes along with the work of Dionysius the Areopagite. In Eastern terms, we might also speak of the Divine Essence, Its Uncreated Energies, and creation.
Saint Bonaventure's Itinerarium Mentis in Deum is sort of a summa here, explaining how God is known in and through created things, through our inner life (microcosm vs macrocosm), and finally by being directed directly upwards, and he ties this beautifully to the six wings of the Cherubim and the architecture of the Temple. The outer reality directs is inward, and inward we look upwards (the pattern of Saint Augustine). -
Language of philosophy. The problem of understanding being
Can there be certainty without stasis?
If stasis precludes life? Is a
This reminds me of a quote I've shared before:
Kant realized that Hume’s world of pure, unique impressions couldn’t exist. This is because the minimal requirement for experiencing anything is not to be so absorbed in the present that one is lost in it. What Hume had claimed— that when exploring his feeling of selfhood, he always landed “on some particular perception or other” but could never catch himself “at any time without a percepton, and never can observe anything but the perception”— was simply not true.33 Because for Hume to even report this feeling he had to perceive something in addition to the immediate perceptions, namely, the very flow of time that allowed them to be distinct in the first place. And to recognize time passing is necessarily to recognize that you are embedded in the perception.
Hence what Kant wrote in his answer to Hamann, ten years in the making. To recollect perfectly eradicates the recollection, just as to perceive perfectly eradicates the perception. For the one who recalls or perceives must recognize him or herself along with the memory or perception for the memory or impression to exist at all. If everything we learn about the world flows directly into us from utterly distinct bits of code, as the rationalists thought, or if everything we learn remains nothing but subjective, unconnected impressions, as Hume believed— it comes down to exactly the same thing. With no self to distinguish itself, no self to bridge two disparate moments in space-time, there is simply no one there to feel irritated at the inadequacy of “dog.” No experience whatsoever is possible.
Here is how Kant put it in his Critique of Pure Reason. Whatever we think or perceive can register as a thought or perception only if it causes a change in us, a “modification of the mind.” But these changes would not register at all if we did not connect them across time, “for as contained in one moment no representation can ever be anything other than absolute unity.”34 As contained in one moment. Think of experiencing a flow of events as a bit like watching a film. For something to be happening at all, the viewer makes a connection between each frame of the film, spanning the small differences so as to create the experience of movement. But if there is a completely new viewer for every frame, with no relation at all to the prior or subsequent frame, then all that remains is an absolute unity. But such a unity, which is exactly what Funes and Shereshevsky and Hume claimed they could experience, utterly negates perceiving anything at all, since all perception requires bridging impressions over time. In other words, it requires exactly what a truly perfect memory, a truly perfect perception, or a truly perfect observation absolutely denies: overlooking minor differences enough to be a self, a unity spanning distinct moments in time.
"The Rigor of Angels: Kant, Heisenberg, Borges, and the Ultimate Nature of Reality."
Sheer change and difference wouldn't really be "change." If one thing is completely discrete from another, if there is no linkage or similarity and relation, then, rather than becoming, you just have sui generis, unrelated things (perhaps popping in and out of existence?). This isn't becoming, but rather a strobe light of unrelated beings. So, leaving aside the difficulty that the past seems to dictate the future, that things seem to have causes, or the difficulties with contingent being "just happening, for no reason at all," it seems hard for me to see how there could be any sort of "sheer becoming." All things that exist are similar in that they exist. If we had "different sorts of unrelated existence," "sui generis types of being," they wouldn't have any relevance for each other. In unrelated moments, we wouldn't have change, just unrelated existence and non-existence.
Now, there is a conceptual priority vis-á-vis difference, and this is important to keep in focus. It's one of the great findings of information theory. But information theory deals with "what something is," and not "that it is," essence but not existence. It skips the former. We can see this in the fact that a perfect set of instructions to duplicate any physical system would not, in fact, be that system. A perfect duplicator, call it Leplace's Printer, needs both instructions and prior existent materials. Information assumes some prior distribution, even if only an uninformed prior, and some recipient. Arguably, this makes it intrinsically triadic (as the advocates of dyadic mechanism wont to point out as a deficit, taking this to mean it is in some way subjective and thence illusory.
However, for those who inherited some of the empiricist modes of thought, the order of knowing has become the order of being, and the priority of difference in discernment is taken to be identical with an ontic priority. I would disagree, for anything to be different it must first exist and be something, "this" or "that," and not nothing in particular. Act follows on being. And in any metaphysics of participation, this linkage is even clearer. The conceptual priority of difference to discernible essence is important, it just isn't an absolute ontic priority. Indeed, the order of knowing and the order of being are, in general, mirror images of one another, reversing the order of each. -
Language of philosophy. The problem of understanding being
Sort of like the Sapir-Whorf hypothesis for philosophy? It's an interesting though. However, it seems to me like Sapir-Whorf has fallen into ill repute in its stronger forms and the empirical support mustered for its weaker forms is, from what I can tell, is quite modest. Certainly, a lot of people have wanted it to be true, and I can see why, as it would suggest that merely speaking differently would open up all sorts of new horizons, but I am a bit skeptical. At least, prima facie, I would think that language is flexible enough to allow for either development in thought. English has being as a noun and verb, and Latin has ens/esse.
For instance, Indo-European languages have produced plenty of process/relational metaphysics. I think that critiques of the "metaphysics of presence" have often themselves painted too much with a monochrome brush (a sort of static presence itself maybe?). Certainly, there is Parmenides, Plato's forms, or Brahman as an ultimate and unchanging reality, but there has also been Heraclitus, Nagarjuna, and countless expositions on the Holy Trinity as fundamentally relational (self-giving love), living activity (or Brahman as activity). Aristotle sometimes gets lumped in as a key purveyor of "static being" or "substance metaphysics," but, were I forced to lump him into either category, I'd probably place him on the "process metaphysics" side. Hegel would be another example.
Not that there isn't a real issue here, although I would diagnose the problem more as two sorts of tendencies stemming from the Problem of the One and the Many. I think it's fair to say that, on average, and particularly since the Enlightenment (or maybe the Reformation), Western thought has tended too much towards the One (toward Parmenides). It has, at times however, lurched into excesses in the other direction. But I see the story as more about an attempt to chart a path between Scylla and Charybdis, rather than Western (and Indian?) thought having remained firmly in the clutches of one or the other. Plato's forms themselves were an attempt to chart a sort of via media here, and Aristotle is largely following his teacher's lead, but showing how the principles of his psychology can be expanded to an explanation of physics, i.e., "being qua changing."
For instance, the fullness of life and understanding attributed to God in much of the "Neoplatonic" tradition (across Pagan, Islamic, Jewish, and Christian thought), God as pure act, strikes me as something that cautions against any conception that is too "static." I'm reminded here of Derrida's "Plato's Pharmacy," which is focused on the ol' "problem of presence." However, I recall thinking that Derrida might be a bit off base in contrasting speech/writing here. In the Phaedrus at around 275a-276d, Plato is pretty clear that his claims about the insufficiency of writing have more to do with the lack of an intellect that knows what is said, and in the context of his overall metaphysics this reveals itself to be more about the insufficiency of finite things, which are more or less just bundles of external causes, and so not intelligible in themselves (being always referred to something else). The point is, as with Saint Augustine's "inner word," participation in Logos. Yet I'd hesitate to call this static. In a way it has to be most alive, lacking nothing. For Augustine and later thinkers in his tradition, it couldn't be a being, or even, univocally, "being," but was "beyond being" (or being/becoming). Dionysius says something on this to the effect of "It is false to say that God exists, but also false to say that God does not exist. But of the two, it is more false to say that God does not exist." -
The Origins and Evolution of Anthropological Concepts in Christianity
Not to add more wrinkles, but in his Orthodox Psychotherapy, Hierotheos Vlachos argues that soul (psyche) is said in two ways in Scripture, as the life of the entire man (including the body) and as our spiritual essence as spiritual beings. He draws on Saint Gregory Palamas here to help make the distinction, likening the spiritual essence of man (one use of soul) to our/God's essence, and the broader usage of soul to refer to our whole life to our/God's energies. That is, the life of the body is the actuality of our soul as a spiritual essence. I am pretty sure I have seen similar distinctions in other places, and made by Catholics (although some Catholics lean more towards a greater separation of body and soul).
As to the churches, that's an interesting comparison. The difficulty for me is that Orthodox Churches are quite rare where I live. I travel pretty far to go to mine and most of the services I attend are at a "mission" that holds its services in a Lutheran Church.
I'd tend to agree that, of the Orthodox Churches I've been to, they seem to do more the capture the idea of the liturgy as taking place outside of time, in the eternal throne room of Revelation, with the whole communion of the saints. And yet, Pope Benedict was writing about this same thing not long before he became pope.
I think it might partly be merely a difference in membership though. Orthodox Churches are rare here, so they are either large or attached to monasteries. Catholic churches are everywhere. I have 5 within ten minutes of me and I live in a rural town with one stoplight. In expanding they have often taken over buildings used by Protestants, and so their aesthetic style is sort of contained by the building they started with. Vatican II was followed by a pretty significant period of iconoclasm it seems as well, along with an experiment with new styles (like the lovely Chapel of the Sun in Sedona Arizona). So, some Catholic churches are very bare, and then some (like the largest near me), do more to incorporate the famous Gothic style (which also took so much from Dionysius the Areopagite in its own way).
The biggest difference in emphasis for me is the centrality of the crucified Christ versus Mary at the front, and the presence of Christ as almighty creator in the dome. This to me is emblematic of a relatively greater focus on the Crucifixion and Atonement, versus the Incarnation. The Stations of the Cross are another example of this. Both of these place an emphasis on the body of Christ, but in different ways.
But, I will here draw an even starker contrast, which are the entirely functional Evangelical church buildings I've been too, which often feel like, or are even built into, strip mall type settings. Here, full coffee shops and donuts are often out front and food is carried into the sanctuary for the service, a far cry from mandatory fasting before the Eucharist.
This might be the best representation of a tradition that attempts to wholly look beyond the physical. It also tends to look beyond the formal, with little formal structure of the "service" (which is explicitly no longer a "liturgy"). Often, the worship service is performed by a band, rather than hymns and Psalms being chanted by the church. Indeed, formal structure is generally seen as inhibiting authenticity, and so too for any physical formality. The idea of the Eucharist as wholly symbolic and mental would seem to go right along with this. What is important is what is going on "inside," not the bread and wine (or now often grape juice), if it even makes an appearance (it often doesn't most weeks).
When I think about this, it seems very strange. The tradition that tends most towards literalism ends up also paying the least attention to concrete instantiations of the faith. And yet maybe it makes sense in a certain way. In the Anglophone context, ethics if often thought to be the main substance of the Church. But this is often paired with a view of ethics as sitting entirely outside nature as command. Likewise, a view of God as primarily will, and of notions of nature as a potentially nefarious limit on that will, would tend towards demoting nature in a way.
So it makes sense in a way, but it still seems to me that fundamentalism and an attempt to excise the influence of Greek thought from the faith might make equally as much sense when paired with an extremely concrete practice, e.g., one that tries to life out the concreteness of the old covenant (maybe Messianic Judaism is sort of an example?)
I will say that in terms of sermons I've heard these do tend to suggest the strongest mind/body and nature/supernatural dualism, even though, at the same time, the general rejection of asceticism, monasticism, celibacy, etc. seem to in some ways go in the other direction. It is a tradition that does not focus on the physical, but precisely because it focuses on the physical so little, it has little time for a sort of Platonic unease with the body. -
The Problem of Affirmation of Life
If you liked Nietzsche I would give Dostoevsky a try. In a lot of ways they have very similar biographies and personalities. Nietzsche was a tremendous fan of Dostoevsky as well. And yet in key ways they could hardly be more different.
Notes from the Underground is a good starting point because it is quite short and less meandering than a lot of his work. Nietzsche was also a huge admirer of that work in particular, although one might suspect on this point that he wasn't totally getting what Dostoevsky was trying to lay down, since, for all their similarities, they come to radically different conclusions about ethics, suffering, happiness, and Christianity. The Brothers Karamazov is his great classic, but it's also a pretty mammoth tome.
Now, understanding the tradition Dostoevsky is coming out of is much harder. I cannot think of a good work that sums it up. It's a sort of project to digest for sure. The book Orthodox Psychotherapy is a good one though.
Another classic that looks at suffering and happiness is Boethius Consolation of Philosophy. It's a charming book that blends together the "first medicine" of Stoicism, which paves the way for the "second medicine," the Platonic ascent as informed by Aristotle, Plotinus, Saint Augustine, etc. -
The Origins and Evolution of Anthropological Concepts in Christianity
Indeed, the idea of resurrection was not new for that time. However, according to my information, it was not the central teaching for all of Judaism as a whole
This is what I understand too, broadly speaking. Although there seems to have been a fair amount of diversity. However, the Pharisees, who play an outsized role in the NT, did believe in a resurrection, as did the Essenes. Unfortunately, the exact religious context is sort of murky. It's sheer luck (or Providential will perhaps) that the Dead Sea Scrolls were found and we have come to know as much as we do about the general context.
I have seen the claim though that the resurrection was more central to Judaism in the late Second Temple period, and that it is rather Christianity, and Judaism increasingly being defined in opposition to it, that led to a pivot away from the theology. More mainstream, it is generally taken that this is why the Jews moved away from using the Septuagint, and the Septuagint has more texts that are friendly to a theology of resurrection.
In conclusion, I would like to present you with an idea from the Orthodox confession about holism. Holism is a view of man as a single, inseparable, spiritual-mental-physical personality. There is no opposition of spirit and matter, but their interpenetration and interaction. As light permeates the air, so the soul permeates the body, forming a single whole. Unfortunately, I do not know for what reason, but none of the Orthodox priests I met mentioned this topic until I became familiar with it myself. Can you share with me about this area if you have knowledge on this topic?
Sounds familiar. The Eastern Fathers and later thinkers stay closer to the formulation of the tripartite soul (appetitive, spirited, rational), whereas this psychology evolved in the West into the idea of the concupiscible appetites, irascible appetites, and rational appetites (will/intellect). I do think this tended to do more to locate the "lower appetites" in the body as set against an immaterial component. Whereas the nous remains more defuse. I do recall this being a tension Saint Gregory Palamas addresses in the Triads, where he affirms a role for the body in the contemplative ascent as against Barlam's "Western" view. However, the more famous high scholastic voices like Saint Bonaventure and Saint Thomas are more nuanced, and I think they can be read as often saying something compatible, but with a different emphases (although the beatific vision as being primarily intellectual could be read in starker terms. I think the more developed Eastern theology of the Transfiguration marks a difference in emphasis here too. -
The End of Woke
I find this assertion strange because the annals of Woke protest letters/debates are full of assertions of an expansive moral and epistemic relativism/anti-realism.
Consider, for example, “thou shalt not be a white supremacist.” In 2017, the president of Pamona College (Claremont, California), David Oxtoby, wrote an email to the entire campus in response to protesters who had shut down a speech intended to be given by Black Lives Matter critic Heather Mac Donald. In the email, Oxtoby expressed his disapproval of the shutdown, arguing that it conflicted with the mission of Pamona College, which is “the discovery of truth” and “the collaborative development of knowledge.”
[The students wrote an open response letter...]
“The idea that there is a single truth — ‘the Truth’ — is a construct of the Euro-West that is deeply rooted in the Enlightenment, which was a movement that also described Black and Brown people as both subhuman and impervious to pain,” the students’ letter stated, according to The Claremont Independent. “This construction is a myth and white supremacy, imperialism, colonization, capitalism, and the United States of America are all of its progeny.”
“The idea that truth is an entity for which we must search, in matters that endanger our abilities to exist in open spaces, is an attempt to silence oppressed peoples,” it continues.
https://www.nationalreview.com/2017/04/pomona-students-truth-myth-and-white-supremacy/ -
The End of Woke
How is this not an argument against the very possibility of totalitarianism tout court, regardless of the ideology consumed by its practitioners? And yet, totalitarianism does exist, and it does not seem impossible that someone who has digested Deleuze or Nietzsche could practice it.
Likewise, your former objection would seem be an objection to the possibility of self-interested behavior tout court. Yet both self-interest as a motivation, and relative selflessness, also seem to exist; there is a meaningful distinction between them. It's the same with rejections of the possibility of weakness of will or the existence of norms.
Might I suggest that if an ideology demands the denial of the very possibility of many of the more obvious features of human life—if it demands that the ideology be affirmed over the obvious—this is itself a sign of potential totalitarianism?
I have nothing against an attempt to find a unifying principle that can be found throughout the appetites, be it "union with the good" or the search for "actuality" or "intelligibility" (arguably all three being the same thing). However, difficulties arise when it is denied that this unifying principle is realized analogously across different appetites, i.e., when all desire and appetition is reduced to a univocal understanding of some term, be it "utility" or "intelligibility." This is, IMO, perhaps the cardinal sin of liberalism, and one its descendants have tended to take on board. An anthropology that is so thin as to make no differentiation between epithumia (e.g., hunger), thymos (e.g., offended honor), and logos (e.g., the desire to "be a good person") is too thin to explain human history, politics, or ethics.
Second, the move to endorse a sort of amoral, disinterested analysis is itself the imposition of a value judgement. I get the basic idea. If we get rid of the moral valence, the blame, and adopt the dispassioned stance of the buffered self, we will avoid getting angry at people (anger is here negative), and thus avoid making the "mistake" of judging people or acts in moral terms.
The problem here is twofold. First, the supposition that getting rid of blame or moral judgement is going to "improve politics" or our "political judgement" seems problematic. On the Stoic view that the passions are simply bad, or at least "bad for reason," it makes sense. Yet the Stoics are wrong here. Rather, what is ideal is to experience just anger over what warrants anger, and likewise to experience just admiration of what warrants admiration. The passions are not a problem any more than the appetites are; only their improper orientation is problematic. The move to exclude morality from political thought is akin to amputating one's hand because one hasn't trained it to preform properly.
A view that advocates the reduction of the human being to a raft of social forces, flows, knots of language, etc., might very well be palliative in that it reduces inappropriate or overwrought anger. However, it can just as easily support callous indifference to suffering and vice. Such a reductionist account also destroys our notions of merit and goodness. It removes the beauty from history and ethical acts. One can certainly study a raft of social forces. One might even try to tinker with it to produce "choice-worthy outcomes." But does one resist serious temptation or suffer hardship for the sake of eddies of social force? Does one stand upon the ramparts in battle and risk maiming and death to save "flows," "sequences," and "concatenations?"
The same problem one finds in liberalism repeats itself here. Civilization requires the pursuit of arduous goods. It requires selfless leadership, a willingness to endure significant hardship and resist extreme temptation even in times of peace, and heroism in times of war. A denial of thymos and logos leaves no ground for such pursuits. A view that dissolves the subject, and thus merit, aside from being metaphysically and psychologically flawed, also fails as being properly pragmatic for society (let alone aesthetically pleasing).
We might suppose then that mercy, clementia, is wanted more than amoralism. That is, "men with great hearts," rather than C.S. Lewis's "men without chests."
The second issue is that such appeals generally tend to be illusory. Dispassion, in the Stoic sense, might be more or less established in individuals, but apatheia is not apathy. It doesn't dispense with values. Were we to dispense with them, there would be no proper standard by which to measure anything. Rather, what one cares to elevate tends to end up being left outside the "bracket" and is raised up on account of all other contenders having been dispatched.
The idea of man as eddies of social force (granted not as fully matured) is exactly the sort of thing I think Dostoevsky argues against to great effect throughout Crime and Punishment. Raskolnikov is only aligned to his natural compassion when he is forgetful of this "new understanding." -
The Christian narrative
Well, it's complex. Saint Thomas cites Saint Augustine more than any other thinker (10,000+ times!) and Augustine suggests we can see the divine image (including the Trinity) by looking within. The second half of his book on the Trinity is a sort of phenomenological dive into the triads that fill the very conditions of experience. Likewise, there was a long tradition of seeing God, and the Trinity in created things (Saint Bonaventure is a great example here). Thomas isn't really at odds with these, but he doesn't think you can demonstrate the Trinity.
However, if one takes the view that all relations are inherently triadic and that the semiotic triad is the precondition for anything to be meaningfully anything at all, I think it's possible to construct a sort of transcendental argument towards the Trinity. -
The End of Woke
Totalitarianism has to lock in, to totalize something. Doesnt it totalize a particular value system? If one says that a radical relativist acquiesces to totalitarianism
because they sanction an ‘anything goes’ approach to values and ethics, how are the systems that are ‘ going’ their own way treated by these radical relativists? Doesn’t anything totalitarian have to get going and then ossify into a self-perpetuating structure? Isnt the indefinite temporal repetition of the same system or structure a necessary condition for calling anything totalitarian? If so, then an ‘anything goes’ relativist would have to embrace the proliferation of an unlimited multiplicity of diverse and incompatible totalitarian systems.
Relativism, even in its extreme forms, does not need to imply that we prefer or will all possible eventualities equally. Indeed, extreme forms of relativism are most coherent (perhaps only coherent) in the context of volanturism. "Anything goes," and "all assertion involves violence" does not imply "so we ought do nothing, ought not tip the scales in any direction." It can suggest this (e.g., ancient skepticism) but it need not. Any such endorsement of neutrality or "live and let live" would itself prove to be insubstantial, merely another assertion of one value over others. Thus, nothing precludes totalitarianism or recommends tolerance and pluralism.
As Hannah Arendt famously put it: "The ideal subject of totalitarian rule is not the convinced Nazi or the dedicated communist, but people for whom the distinction between fact and fiction, true and false, no longer exists." Orwell riffs on a similar insight throughout 1984, and Soviet writers have gone into depth on how this was applied as praxis.
If so, then an ‘anything goes’ relativist would have to embrace the proliferation of an unlimited multiplicity of diverse and incompatible totalitarian systems.
Why? Are they committed to some sort of inviolable principle that leads from the truth of relativism to this sort of open-ended tolerance? I don't see why they would be.
To wit:
But all these are only preliminary conditions for his task; this task itself demands something else—it requires him TO CREATE VALUES. The philosophical workers, after the excellent pattern of Kant and Hegel, have to fix and formalize some great existing body of valuations—that is to say, former DETERMINATIONS OF VALUE, creations of value, which have become prevalent, and are for a time called "truths"—whether in the domain of the LOGICAL, the POLITICAL (moral), or the ARTISTIC. It is for these investigators to make whatever has happened and been esteemed hitherto, conspicuous, conceivable, intelligible, and manageable, to shorten everything long, even "time" itself, and to SUBJUGATE the entire past: an immense and wonderful task, in the carrying out of which all refined pride, all tenacious will, can surely find satisfaction. THE REAL PHILOSOPHERS, HOWEVER, ARE COMMANDERS AND LAW-GIVERS; they say: "Thus SHALL it be!" They determine first the Whither and the Why of mankind, and thereby set aside the previous labour of all philosophical workers, and all subjugators of the past—they grasp at the future with a creative hand, and whatever is and was, becomes for them thereby a means, an instrument, and a hammer. Their "knowing" is CREATING, their creating is a law-giving, their will to truth is—WILL TO POWER.—Are there at present such philosophers? Have there ever been such philosophers? MUST there not be such philosophers some day? ...
Nietzsche - Beyond Good and Evil - Ch. 5 We Scholars - Section 211
In NEW PHILOSOPHERS—there is no other alternative: in minds strong and original enough to initiate opposite estimates of value, to transvalue and invert "eternal valuations"; in forerunners, in men of the future, who in the present shall fix the constraints and fasten the knots which will compel millenniums to take NEW paths. To teach man the future of humanity as his WILL, as depending on human will, and to make preparation for vast hazardous enterprises and collective attempts in rearing and educating, in order thereby to put an end to the frightful rule of folly and chance which has hitherto gone by the name of "history" (the folly of the "greatest number" is only its last form)—for that purpose a new type of philosopher and commander will some time or other be needed, at the very idea of which everything that has existed in the way of occult, terrible, and benevolent beings might look pale and dwarfed.
Section 203
Or also:
"What is good?—Whatever augments the feeling of power, the will to power, power itself, in man.
What is evil?—Whatever springs from weakness.
What is happiness?—The feeling that power increases—that resistance is overcome.
Not contentment, but more power; not peace at any price, but war; not virtue, but efficiency (virtue in the Renaissance sense, virtu, virtue free of moral acid).
The weak and the botched shall perish: first principle of our charity. And one should help them to it.
What is more harmful than any vice?—Practical sympathy for the botched and the weak—Christianity."
- Antichrist 2 -
The End of Woke
I still think it is worth considering why such pluralist sources such as CT and post-modernism, vastly lead to the same progressive conclusions. If it was even 59% it wouldn’t be a good question, but it has to be more like 90% or more. Something is off about the PM and CT methodologies, where all of these more relativist/ pluralist thinking structures, like a funnel, yield the same societal conclusions.
(The pluralist/relativist baseline is why they avoid any sense of self-awareness of their own brand of facism and absolutism that can result when they have power and seek to impose these vastly uniform progressive conclusions.)
Prima facie, it didn't have to lead that way. Many of the early adopters of Nietzsche who rescued him from obscurity leaned to the right. I actually think systems of power, decentralized incentive structures, cultural biases, the systems of power inherit in careerism, and an oversaturated job market, etc. are quite good explanations here (which supports the original position). It's also worth noting that in the US context Christianity had held up remarkably well in comparison to the rest of the West until quite recently. Hence, it could remain a sort of "mainstream" custom to rebel against (even for foreigners, due to the outsized US influence), and our culture has a marked preference for iconoclasm. Anti-realism can suggest the embrace of custom on aesthetic or other grounds, but this line didn't suggest itself.
However, note that the emergence of a right wing (post-Christian) post-modernism skews quite recent. I don't think this is incidental. In bourgeois coastal society, and particularly in academia, traditionalism finally became properly transgressive. (I happened to be reading Origen and Saint Maximus at the same time as Byung-Chul Han and Mark Fisher and it struck me that the former two were by far the more radical and transgressive in the current context, and not because of a traditionalist absolutism, but because of their radical optimism, aesthetic outlook, asceticism, and total lack of irony).
At the same time, a new post-Christian branch of the GOP coalition grew in size and influence, meaning such theorists would actually have allies and support to pull on. -
The End of Woke
Concepts like status, self-interest, power and control can inform diametrically opposed positions depending on how the subjectivity, or ‘self’, they refer back to is understood. If we start from the self as homo economicus, a Hobbesian figure the attainment of whose desires need not have any connection with the desires of others, then we either settle for a Darwinian Capitalism or find a way to insert into this self an ethical conscience which we will not always be able to depend on. If instead we see the self not as an entity but as a process of unification, self as self-consistency, and desire as oriented toward anticipatory sense-making ( We don’t desire things, we desire coherence of intelligibility), then there is no i weren’t slot between the needs of my own ‘self’ and the needs of other selves. The unethical is then not a result of bad conscience but a failure of intelligibility. The unassimilable Other is found wherever injustice occurs (slavery, genocide).
Sure, you could describe it lots of ways. You could also think of it as a system of (perverse) incentives.
I have argued that the doctrine of nihilistic will to power is not a plausible explanation for the moral absolutism characteristic of wokism. Such absolutism can only justify itself on the basis of a realist-idealist grounding of some sort, which happens to be the stock and traded of Critical theory. I suggested in another post that the most noxious totalitarian tendencies of wokism can be moderated or even eliminated as more activists discover Habermas’s hermeneutical, communicative brand of Critical Theory and begin to leave behind the violently oppositional language of folks like Adorno, Fanon and Gramsci.
I'd place the main influence for the appearance of absolutism within the earlier activist traditions, which were firmly embedded in Christian and Islamic contexts, and made use of prophetic language. However, is "moral absolutism" really characteristic of Wokism?
I know some pretty Woke folk, and I cannot think of a single one who would endorse moral absolutism if asked. Rather, you'd get epistemic relativism and meta-ethical anti-realism. I happened to be in an ethics class during the height of the Great Awokening and this was precisely my experience.
Generally, any absolutism is held to as a sort of performative contradiction; the absolutism of Woke is primarily performative and volanturistic (indeed, both absolutism and negativity towards performative contradiction seem like the sort of things that are likely to get written off as a sort of cis-het-white-male-Western-etc. normativity, merely an assertion to be met with counter assertion, or even a sort of epistemic violence that tries to enforce a logical binary on expression).
Second, I'd be wary of conflation totalitarianism and moral absolutism. You can, and often do, see one without the other. Indeed, even moreso on the right, you see arguments for totalitarianism precisely because relativism and anti-realism are the case. Whereas plenty of quietist, pacifist, isolationist movements hold to a strong moral absolutism. Getting rid of absolutism doesn't necessitate a move away from totalitarianism; it can in some cases motivate the opposite move (indeed, I think the case in point is such an example). -
The End of Woke
Anyway, another CT insight is that even resistance (wokeness or anti-wokeness) can be turned into a commodity in late stage capitalism.
:up:
Mark Fisher has a charming explanation of this in Capitalist Realism. But other theorists see this not as a property of late-capitalism, but of reality and discourse itself. There is no "escaping capitalism" because it is simply the structure of reality—a rather terrifying thought. -
The End of Woke
I just wonder why this process which sounds like it should be neutral as to outcome always yields the same political conclusions. Liberal wokism is the only result of postmodernism - how is such uniformity of outcome possible given such undefined unformed clay as “bodily, material and social interactions.”
It doesn't always. There are right-wing descendants of Nietzsche who also draw from Derrida, Deleuze, etc. as well as critical theory, although they tend to also mix in influences no one else pays attention to, like Evola and Spengler. For example, there is Nick Land, Curtis Yarvin, Costin Alamariu, the whole Dark Enlightenment Crowd and the various "Neoreactionary" projects. Dugin and some other Russians might count; indeed people who are deep into this stuff tend to point to a lot of people who aren't writing in English. They are obviously vastly rarer and not particularly welcome in the academy, but they have been influential through other avenues, particularly in the right wing media space and through their evangelism of Big Tech leaders. Here, the groundlessness of hierarchy and values are precisely why they need to be forcefully asserted (not made known, but constructed and endorced).
There are also some eliminitive materialists (analytics) who pick up on post-modern theory. The science they rely upon to ground their eliminativism is for them a social construct, subject to all sorts of caveats and anti-realist arguments. Nevertheless, reality is at root difference, adaptation, conflict, and natural selection. Their theories are "adaptive." These folks seem to tend towards something like the "natural selection meets whig history" defense of liberalism.
Note however that a denial of "moralizing" is hardly unique to this area of thought. Nozick, as an exemplar of conservative liberalism, and Rawls as an exemplar of the progressive variety, both differ from politicians expressing either ideology in that they deny any role for merit and virtue. The "Good" is privatized and what is left is a procedural "right." The desire to bracket away the "normative" and a tendency to see the Good as both a private issue and ultimately irrational or subrational (if even real), as well as a distaste for moralizing, is more a generic Enlightenment idea, at least in the Anglo-empiricist tradition.
This actually brings to mind the epithet "social justice warrior." There is a bit of truth here, in that conflict and crusade are part of the ideological framing. Warrior societies tend to generate wars, and I'd argue that "activist" societies will tend to likewise generate social conflicts. If these are the arenas where status is won and identities are built, than one must "take to the field."
60s radicalism gave birth to a generation of yuppies, and the co-option of the hippie movement into a sort of consumerist hedonism. Perhaps something similar will happen here. I sort of doubt it though. 60s radicalism could follow this path because the academy still represented a viable career path for those more attracted to radical critique, and other pursuits like the priesthood still attracted a fair number of people who were oriented towards either the "spiritual" or "active" (charitable) life, while the path towards an individualist hedonism also remained easy to follow and hadn't proved itself to be "insufficient" within the larger cultural and artistic context to the same degree.
But median wage growth stagnated in 1979, almost half a century ago now, and economic mobility began to decline. The path to hedonistic consumerism closed up. I would argue that increasing earnings within a lifetime and a sense of "personal progress" are much more important to this way of life than absolute earnings. Meanwhile, academia and traditional non-profit roles filled up (and academia is now facing a catastrophe, as enrollment will actually start to dramatically decline). 1990s depression and angst replaced 70s and 80s hedonism (grunge and gangster rap versus hair metal and new wave). Secularism and relativism make building a life of meaning difficult. "Find a career that is meaningful for you is terribly open ended, especially when given to someone raised in the context of secular moral anti-realism and modern consumerism.
New Age and secularized Eastern religions offered one escape path here, but the Christian ethic of social justice and the ideal of freedom and perfection as the communication of goodness to others (agape descending, not just eros leading up) is pretty hardwired into Western culture, such that secularized Buddhist mindfulness can be found lacking in a certain degree of outwards focus.
So, there is a closure of other outlets, which funnels people towards social justice activism as their "worthy aim." At the same time, people are shut out of lives spent pursuing these higher ends because academic and non-profit jobs becomes extremely coveted and scarce, and the rise of the low paid adjunct and unpaid intern make the "life of meaning" increasingly class-based, in that one needs wealthy parents to (comfortably) support such a career. This pushes people aligned to activism as a "way of life" or "source of purpose" into all sorts of other areas of the workforce, from boring local government jobs, to medical research, to K-12 education, and particularly Big Tech. And then these become a site for conflict, because they are actually often set up precisely to avoid such issues, while social media reduces the cost to begin and organize activism (while also creating echo chambers).
That's at least how I heard a Silicon Valley CEO describe his and his peers' journey to Trump. A lot of these were younger CEOs, big Obama supporters, and tended to initially be quite open to the post-2008 "Great Awokening." But as it picked up steam (and because they tend to hire from its epicenter in elite universities) they began to face an actively hostile workforce who saw their employers as "the enemy" who needed to be wholly reformed from the inside. Or at least, this is how the experience felt to him, and he described a lot of hostile meetings, internal protests, etc. that ultimately soured him on the left.
And this is perhaps where mainstream responses to Woke are most deficient. Because of the anthropology that dominates modern thought, there isn't much acknowledgement of the rational appetites. Yet I'd argue that people's desire to "be good" or "do what is truly right," is, when properly mobilized, the strongest motivator of behavior, trumping safety, pleasure, or even thymos. When this desire becomes aimless or frustrated, trouble will arise (which reminded me of another article on the parallels between Woke and Evangelical Christianity). -
The End of Woke
I'm certainly not committed to the idea that all philosophy is good, and that all philosophical "progress" is necessarily part of some sort of providential unfolding. It seems to me that some philosophies are bad, having a largely negative impact on philosophy itself and culture in various epochs. That is, the good is sown alongside the bad in history, and the history of philosophy has been no different. So, prima facie, I'm not necessarily looking for some sort of theodicy of reason whereby all ideologies will necessarily lead towards a better future (except as perhaps an example of which paths not to follow). Hence, a properly broad view doesn't need to find a silver lining in every cloud.
That said, I do have a hard time thinking of influential philosophies that are wholly without merit. As respects individual thinkers though, and on the balance—I'm not sure if Ayn Rand or "Bronze Age Pervert" represent any sort of teleological advance, let alone Richard Spencer. If they result in "progress" it will be accidently.
Much of the critique Ive read so far ranges from ad hominem attack on character flaws in the activists ( status seeking) to historical regressiveness ( it’s a return to fascist thinking or a twisted variant of Romanticism.
Appeals to status seeking can be merely descriptive as well. It doesn't seem they are prima facie wrong. If they were categorically off-base, then it would also be the case that segregationists and white nationalists cannot be acting to defend their own status and interests. Yet that is, quite explicitly, what they claim and understand themselves to be doing. In their newer forms, they just claim that everyone else is also doing the same thing, covertly or not, and that they're at least honest about it. However, earlier defenders of segregation were much more covert about their ends, and yet I hardly think we can avoid the conclusion that these too were also partly motivated by defending their status and control over resources.
Second, I think I'm the only one who mentioned fascism and the idea (Milbank's, although the seeds can arguably be found in Dostoevsky) is that the logical conclusion of the ontologies of violence is fascism. That is, when there is no transcendent order of peace, goodness, or truth, instead only contingent systems of power, difference, and conflict—when truth, law, and morality are not a participation in Logos, but are rather constructed through acts of force (e.g., discourse, statecraft, capital, language games)—then violence is original, and there can be no counter-violence which truly transcends violence. There is only ever assertion over and against counter-assertion, will to power against will to power (plus or minus some post hoc rationalization, which is itself merely another assertion of value). This is precisely the spiritual logic of fascism.
Now, against this, I suppose are the "natural selection meets whig history and Hegel" accounts of the superiority of liberalism, where liberalism is superior because it is more stable, wins wars (through the promotion of technological and economic innovation and growth), and is better at both non-violent (physical violence) coercion and positive conversion, and kinetic struggles in the long run. But even if this were true (and the growth/power trajectories of China and the US versus say, Northern Europe, suggest it isn't) this isn't a normative justification of liberalism, but just an endorsement of it as a strategy of power. Plus, if liberalism entails democracy and liberalism cannot be electorally successful, that's an internal contradiction in the strategy. It might be that liberalism simply represents a bundle of "self-replicating, persistent strategies" that could be even better assembled under a fascist technocracy (e.g., "neo-feudalism.")
The other point is that the philosophies of critique can only tear down, often due to an obsession with ideological purity and negative liberty (Hegel's charge against French radicals). So, they themselves don't pivot towards fascism. Instead, they tear down existing norms, narratives, and institutions, but lack the wherewithal to replace them with anything viable. This opens of an ideological power vacuum where more robust discourses of violence can flourish, which is, I would argue, precisely what we have seen. If the left continues to meet political failures, which I think is very likely due to technological and economic changes, even if they have some merely electoral successes, I wouldn't be surprised if more explicitly ideologies of power rise to the top there as well, as characterized by the "ironically unironic" embrace of neo-Stalinism in far left online spaces, where Kulak memes might prove to be what "ironic" Nazism was to right wing spaces a bit over a decade ago.
As for Woke becoming the dominant ideology the way Neoliberalism has been in 50 years, in 50 years China and India will be the world's largest economies. The EU in particular is on a growth trajectory to become increasingly irrelevant, and the war in Ukraine has shown that it seems likely to continue to underperform its economic standing in both hard and soft power. It would take a radical sea change for these ideologies to be allowed to get anywhere in China, even if they were popular there (whereas they are popularly ridiculed on Chinese social media). I don't think India will prove exceptionally fertile ground either. Whereas sub-Saharan Africa will be to that epoch what Southeast Asia was to the 90s-2020s, the main target for new investment and consumer markets, and there are a lot of reasons to suspect Woke would need to be radically transformed to have an appeal there too. I'm just not sure that it will make sense in these settings, and a look at how Woke analogs have developed in Japan and Korea might be a good indicator here. In particular, the Sexual Revolution seems key to Woke, and yet this is probably the number one area where thought indigenous to the developing world has said: "no thanks," and "please stop trying to force this on us." -
The End of Woke
Well, there was no "anti-woke crowd" before wokeness, and wokeness ironically created much of the sentiment that it claimed to oppose, such as racism. I actually think wokeness is largely self-generated. I think it has to do with a "civil rights warrior" mindset that had largely run out of issues to champion, and so it had to start conjuring them in the form of "micro aggressions" and whatnot. Since at least World War II we have created a sort of internal righteousness monster that needs to be fed. If there are no obvious injustices then injustices must be conjured up or else minor issues must be magnified, even at the cost of great collateral damage.
Absolutely. I wrote an article a while back that World War II has become the "founding mythos" of modern liberalism. In doing this, it has made (generally manichean) conflict and struggle a bedrock part of identity formation in a way that is unhelpful.
But then there is also the disparagement of custom that is so obvious in thinkers like J.S. Mill, which has become almost a heroic virtue in contemporary society. It's a sort of trope of modern hero narratives that the heroic protagonist has no time for custom and "paves their own way." This can be seen all over the Marvel movies, or in hit shows like Buffy the Vampire Slayer, etc. The antagonist, by contrast, often represents a sort of stereotyped, medieval, authoritarian archetype.
It's made for plenty of great media, but the problem comes when transgression is valued for transgression's sake. That's how you get caustic, counter-productive, purely performative activism. I'd also argue that it's how we got a real resurgence in unapologetic fascism and neo-Nazism. Hitler became the face of evil, the ultimate taboo, and so of course those who value transgression cannot keep themselves away from Hitler, even if only ironically at first (e.g., the Sex Pistols used to parade around in swastika shirts). But the "taboo appeal" of Hitler and fascism seems to have actually transformed into a potent recruiting tool for unironic Nazis. I'd argue that at least some of the continued appeal of the Confederate flag has similar roots.
I don't think I'd say that we necessarily ran out of issues to champion. I'd say the larger issue is that every issue tended to take on the urgency and Manichean dimensions of the Civil Rights Movement. For instance, migration has obviously often been reframed as simply a continuation of the Civil Right Movement, where opposition to a maximalist immigration policy becomes a sort of explicit racism in the way Jim Crow was. Or Ta-Nehisi Coates (among plenty of others) looks at the Arab-Israeli conflict, and sees the Civil Rights Movement as the obvious analog. Some environmental issues disproportionately impact some minority populations, and so it becomes a Civil Rights-style issue, etc. -
The Origins and Evolution of Anthropological Concepts in Christianity
That's a very broad question. I think it comes down perhaps to evangelical zeal and praxis, although providence is another option!
But Christianity did a lot that was new, particularly through synthesis, including its understanding of the resurrection and judgement, and divine union. It just wasn't a totally new idea. Also, the OT leaves Sheol/Hades very ambiguous and my understanding is that there were vying interpretations in the Second Temple period.
...which reminds me of another influence. Homer has the shades of the dead existing after the death of the body, and Virgil picks this up too. The state of the body has consequences for the dead. Those who have not had proper burial rights end up having to wander around the Styx for a lifetime before they can make it to the Elysian Fields (in Virgil). However, the existence of the shade isn't dependent on the body. Sheol is much more ambiguous, but the Greek rendering of it as Hades in translations of the OT suggests a certain conceptual overlap by the time of the Septuagint.
How literally such scenes were taken surely varied a great deal of course. -
The End of Woke
Well, al-Gharbi traces it back plausibly to early 19th century American politics, particularly in the context of abolitionism (which was also a quite religious context, although that's true for most American politics in that century, but it's even more true for abolitionism). Back then though it was being "wide awake." "Woke" in its current format seems to date to 1970s African American activism. It wouldn't surprise me if it was being used, somewhat ironically, for more outlandish conspiracies in that period.
On a related note, there is a broad irony here in "intellectual histories of Woke"—those created by both critics and allies alike—largely or wholly consisting of "dead white men." Whereas the various waves of feminism (predating post-modernism), abolitionism (from whence it gets its name), liberation theology, or, particularly in the European context, the blend of Pan-Arabism to Islamic feminist, to even Salafist thought that flavors their version, etc., all seem to play larger inspirational roles. I don't think it would be unfair to say that Woke has been more Dworkin, less Derrida, or more Huey Newton, less Nietzsche, and more Malcom X, and less Marcuse.
The prophetic and evangelical language comes in because Woke springs from movements (fairly recently and sometimes still) grounded in Christianity and Islam. Sometimes this gets traced back only as far as Marxism, but the whole idea of the Marxist "conversion" is a self-conscious adaptation of the Christian frame it emerged in (early early-modern communists having been Christian radicals whose main inspiration was the Book of Acts). The heavy focus on embodiment is also arguably more a legacy of this other tradition that has made it into broadly post-modern academia, and not vice versa.
That's also why I don't think some of the behavior is going anywhere. It has a 2,000 year legacy in the West. And some of the issues related to demographic tensions are only likely to get more acute in coming decades. Yet the philosophical underpinnings are not unimportant. They helped to unmoor the evangelical and prophetic approach from any philosophy of proper authority and from values themselves. In this, the prophets become their own standard, whereas the tradition of prophetic critique is that the standards the prophets appeal to lies beyond them. This is what allows for a witches' brew where Salafi clerics from groups with members who speak openly about beheading gays and driving "Jewish filth" from the Middle East can be invited to speak under pride banners.
As noted above, I don't disagree with the relationship here between the prophetic tradition and Woke, although I am not sure about Woke somehow being a more authentic and successful transcendence of the pagan celebration of force (which was itself always contained by a fatalism and sense of piety that modern forms of neo-paganism tend to lack). I'd argue that Woke is largely different from the earlier social justice movements that it takes as its main sources of inspiration precisely because it has shifted to a philosophical underpinning that embraces the post-modern "ontologies of violence," and notions of difference as inextricably bound up in warfare.
I don't think this point isn't ancillary. It seems to make it impossible to articulate why Woke should be embraced above any other ideology. If all ideologies are historically situated power grabs, why support one over the other? Any appeal to standards and values here will itself simply be just another attempt to hold one set of values above all the others, a move of domination.
As such it harkens to an undercurrent that has always had appeal. We find an aesthetic of the victim, potentially very powerful, in the figure of Jesus Christ. The aesthetic of the victim personified. However, this aesthetic was never dominant. The cross quickly turned into a symbol of dominance itself. In its name crusades were fought, witches were burnt, and churches were erected. All of these were never in the spirit of the victim, but always of the victor. Churches were erected on the burial grounds of the vanquished, trials were inquisitive, treating the suspect as an object and the crusades were little more than an excuse to plunder. Nietzsche wrote about the herd mentality cultivated by Christianity, but this herd was only a herd because it had a leader. The herd never led itself but always embraced the principle of the strong man. In short, the aesthetic of victory always dominated. So, for 2000 years we have lived with an aesthetic of violence, conquest, and growth.
This brings to mind the responses of John Milbank, David Bentley Hart, and others. Their claim is that it is only through an aggressive misreading and highly selective account of Christianity and Christian history that post-modern critics are able to support their genealogical narrative of Christianity as "just another face of power," their point being that, if this narrative is itself merely "one more interpretation," then the ontologies of violence don't end up being unavoidable. Rather, they themselves simply represent another possible choice in interpretation, one among many open to us.
In line with that, I'd take qualms with the word "quickly." Christianity only stopped being an (at times quite aggressively) persecuted minority and became a legal religion over three centuries after it began. Compared against the history of the United States, with 1776 as our starting point, today we'd still be more than half century from Christianity even becoming legal (and still by no means hegemonic, Julian the Apostate would lie in the future). Indeed, we would be lined up with a particularly brutal persecution, an attempt to exterminate the faith. The First Crusade is almost 1,100 years after Christ's ministry. It is closer to our time than to Christ's or Saint Peter's.
The cross no doubt became a "symbol of dominance," but that is not all it has been. Rather, it functioned according to Maritain's best law of history, drawn from the Parable of the Sower (Matthew 13:24-30), that the good is sewn with the bad, and that the two grow up alongside one another. Each historical phenomenon, be it the industrial revolution or Christianity, has had positive and negative effects. That's how Christ chose to describe the fruits of his own mission. But against the view that reduces this to something like the Will to Power, I'd recommend something like Solovyov's view of history as the threshing floor on which falsity is paired away from truth. In history, we come to know ideals by their fruit. -
The Origins and Evolution of Anthropological Concepts in ChristianityRelics are also relevant in the other direction. There can be nuance on relics, but on pretty much any sound theological view handing over gold so that you can touch the severed hand of some saint is not going to ensure you have a bumper crop or make the fairest local maiden fall in love with you. The origins of the later dualism seem to have something to do with the desire to pull back from this sort of far extremity of the identification of the holy man or woman with their body, where it becomes a sort of magical power than might be employed towards all sorts of ends.
-
The Origins and Evolution of Anthropological Concepts in Christianity
You pose an interesting question with a well thought out OP. A difficulty here is that the relevant ground to cover is incredibly broad, because there are so many different veins of Christian and Pagan thought that are relevant.
Just some things I noticed:
Christianity introduced a revolutionary concept of resurrection at the time
As you note, this is also present in what is often taken to be the latest book of the OT, Daniel (although some still argue for an earlier, exilic dating). There is also Ezekiel's vision of the Valley of Dry Bones coming back to life, which is almost always dated to the Babylonian Exile and thought to be the work of a single man for various textual reasons (593-571 BC). The idea of the resurrection shows up in some of the Septuagint texts, but the most relevant point is that Jewish belief (and lack of belief) in the resurrection of the dead was a hot issue by the time of Christ's ministry and Acts actually has Saint Paul playing different camps off against each other on this issue when he is hauled in for questioning.
"Platonism," broadly speaking, had also already worked its way into Judaism by this point. It's in the Old Testament wisdom literature, Sirach, but particularly The Wisdom of Solomon, and Philo, probably the most famous ancient Jewish Platonist, was writing when Jesus was young. So, Platonism (as a broad set of Middle Platonist ideas) has a doorway into Christian thought because it is already a potent force in the Roman Empire and within its Jewish communities, and because it is in some ways written right into OT and NT texts (e.g., Wisdom and John).
What if there is no separate, disembodied soul existing apart from the person? What if the human body is not a cage, not a mortal and base vessel, but a valuable creation destined for glorification? What if humanity is valuable as such, in its inseparable wholeness of spirit, soul, and body, and its resurrection after death is the sole truth about the afterlife, offering hope for a complete existence in a transformed state?
That's a direction a lot of the early monastics went in. Creation is inherently good, and so is the body (hence the resurrection), hence the body in its natural state is good. A quite common theme in the Patristics is that man's current state is unnatural, and that man is in need of healing to achieve Adam's former state. In the unnatural, fallen state, man is disordered, and this is why the body, or "flesh" is problematic, because man has not been properly ordered and harmonized. Whereas, the holy man is rightly ordered to the body. Hence, the "flesh" and "world," or "passions" to be rejected are not the body, physical reality, and emotion, but rather all misaligned love and disordered desire and activity.
Not that there weren't threads that tended more towards rejecting the body and physical reality, Gnosticism being the prime example. These never went away fully, although I think it's fair to say they were defeated and became subterranean for long periods. Hence the idea that the body of holy men becomes itself holy, leading to the cult of bodily relics (the opposite extreme).
The condemnation of Origen and Evagrius are a pretty important chapter in this push and pull.
Not to make things more complicated, but there was another view layered on top of this, a trichotomous view of man as body, soul, and spirit (often drawing on Saint Paul's writing, which does seem to offer up such a distinction in many ways).
The drive towards dualism is bound up in a larger trend in the thought of late antiquity, where it starts to look inwards (this owing to the instability of political life and political opportunity for the well-educated, as power shifts to the legions and public life and the culture of public duty deteriorates). Stoicism and Pagan Neoplatonism play a large role here, and shaped Christianity quite a bit, for centuries to come since the same ideas keep coming back. The early-modern period saw a sort of resurgence of these Stoic elements (https://en.wikipedia.org/wiki/Neostoicism), which did a lot to set the ground for Descartes, the "Buffered Self," and stricter mental/physical and subject/object dualisms. The other big factor often identified here is Franciscan spirituality, which at times tended towards a very "other-worldly" focus. I don't think you can see even the outlines of this problem in Saint Bonaventure, who is still striking a far more harmonious balance, but these tensions do seem to start to build pretty rapidly not long after.
Now, at the risk of saying something overly broad, my feeling is that the "dualism" of late-antiquity and the middle ages tended not to raise the same philosophical or spiritual problems that dominated the early and later modern periods because the standard metaphysics was quite a bit different. The soul's ability to "exist" apart from the body is less stark in a metaphysics of participation and of creation as more akin to emanation, where substance is understood through an act of existence that is ultimately God's. The Divine Comedy, for instance, is extremely "bodily" and Dante does not have to go beyond his body to a "spirit realm" to encounter the dead. Indeed, he can see the damned and those being purged just fine from the body; it is only traversing the heavens that requires "transhumanization" and an ambiguous sense of leaving the body behind (the ambiguity mirroring Saint Paul's vision). By the time Descartes is writing, things are much different. Substance is a sort of independent substrate, and while I think his solution to the resulting mind/body problem is a poor one, I can see why it was appealing.
An interesting topic indeed. Origen and Evagrius are good examples of the "flight from the body," and Saint Maximus the Confessor a good example of the correctives that were offered to this that tended to become more dominant (for a period). -
The Christian narrative
That unit is prior to multitude isn't really about the Holy Trinity, it's just relevant to speaking about the topic. Unlike Bob, Aquinas does not think the Trinity can be known through natural reason, only that God exists.
On the topic of willing/knowing mentioned , this, particularly article 9, would be the relevant question.
God, being eternal, knows all that is or ever will be actual. But God also knows all things that are potential since they exist as potential.
But I think this may just be the same misunderstanding in play again, i.e., that all distinctions must be real, such that willing and knowing can not be meaningfully distinguished unless they are metaphysically distinct. But that a fire is hot, heats, and illuminates, does not require three distinct flames or three distinct composite parts of a flame. Likewise, we see many things with our eyes, but we need not suppose a distinct act of seeing for each thing that we see, although obviously we can meaningfully distinguish between seeing different things. -
The Christian narrative
Right, numerical identity (dimensive quantity) is posterior to virtual quantity (qualitative intensity) and anything's being any thing at all. Unit (and thus number, as multitude) is posterior to measure. Which is just to say that, to have "three ducks" requires "duck" as a measure, etc. God's unity is transcendental however, in the sense that all being is unified. "Thing" and "something" are also considered derivative transcendentals (in the same way beauty is). They are prior to numerical identity in that you cannot have "numbers of things" without things; multitude presupposes units. The supposition here is that numbers exist precisely where there are numbers of things, hence their posteriority, although they are prior as an absolute unity in God (normally attributed to Logos).
Part of the idea of their pre-existence God is that all effects exist in their causes. But it's also the case that no finite idea is wholly intelligible on its own (just as multitude is not intelligible without unit). Hegel's Logic is largely extending this idea. Only the "true infinite" can be its own ground. -
The Christian narrative
Despite claiming god to be a simple, it juxtaposes will and intellect; subject and object; father and son and so on. But those distinctions are the very thing denied by divine simplicity. The argument rests on this contradiction. Now we know that a contradiction implies anything, so we should be wary of an argument that is so dependent on contradiction.
As I pointed out earlier, this is a misunderstanding. There is only a contradiction if we assume that:
A. Any distinctions made vis-á-vis God require/imply composition. However, this is not the case. And;
B. All distinctions must be real, and not rational/notional distinctions.
This demand that all distinctions be real is particularly problematic. Must a glass that is called "half empty" necessarily be a different, distinct metaphysical entity from the same glass when referred to as glass "half full?" Such a demand would be particularly fatal for nominalism. It would require that any meaningful speech about anything be based on real metaphysical distinctions. If "true" can be said of all that is, for instance, it would require that truth somehow be metaphysically distinct from, and thus beyond the category of being (which is itself supposed to be maximally general). The goodness of a good car would have to be metaphysically distinct from the car, etc.
If we say: "a simple thing exists," and "a simple thing is non-composite," are we thereby actually committed to a composite simple thing, since we must distinguish the simple thing's existence and its non-composite nature, because we have made a distinction between the two? And because it might also be called "something" or "true," is it also composed of "somethingness" and "truth?" -
The End of Woke
There is nothing amoral about the classical economic notion of selfishness, which is why al Gharbi’s thesis is so compatible with it, and in fact depends on the same Enlightenment-era notions of the autonomously willing subject.
In its original development, maybe not, although key figures there tended towards a view of morality as mere sentiment (although sometimes divinely authored mere sentiment!). However, it developed towards the idea that people behave mechanistically, at least in the aggregate. People are "rational agents" only in the sense of utilizing data computationally to achieve their ends; however, morality is excluded from this (wholly instrumental) "rationality" and rolled into the black box of utility instead (i.e., essentially marking it under "tastes"). Morality, to the extent it shows up, comes into play in the ultimately irrational preferences agents rationally attempt to fulfill. Anything more substantive is bracketed out to a special "normative" sphere, outside the purview of positive economics.
Hence, work in political economy can speak of supply and demand curves for terrorism, fatalities, or suicide bombings. Campaigns of gang rape can be reduced to "costly signaling" and "hand tying" as a sort of rational, game theoretic maneuvering exercise to maximize utility payouts. Man, as described in reality, functions amorally. Morality, when it shows up again in normative political economy, is superadded onto a presumably complete description (obviously, there is a sort of presumption here, in that it must be assumed that the part, economic and political life, can be properly described without reference to a whole that presumably includes morality and value).
Nietzsche's descendents are far from the only ones who think they have ascended above good and and evil. There is also certainly a strong view that clear-eyed analysis looks at efficiency and optimization, and that when one steps into the realm of explicit values one has in a sense stepped downwards, into "doing politics" and not "political science." Consider the opinions of some "effective altruists" towards "morality" versus efficiency.
But, it seems to me that in all these cases the very claim that it is better to have risen "above" morality is:
A. Itself a moral claim; and
B. Self-delusion.
One can place oneself above the intellectual plebeians who still think in moral terms—in terms of righteousness (as the some partisans of the positive over the normative often do)—but this ends up being itself a value-laden dismissal. To turn against moral judgement is already to have made one—against what is seen as simple-mindedness, absolutism, false universality, etc. Such a move is not actually free of values, but rather simply committed to different values, such as irony, distance, subversion, or procedural neutrality.
Yet, we might just as well argue that man is a moral creature and indeed that this is precisely what elevates him above the brutes or the status of a mere machine. Indeed, perhaps we ought to hold such a view. That is, to try to erase value from one's considerations of politics and the world is not to "rise above" good and evil, but simply to become evil. It is a sort of intellectual and moral degeneracy that is then locked in place by an overweening pride in one's own superiority and capacities. It's the result of the distinctly modern pathologies of a straitjacket intellectualism that makes the current limits of man's systems and language the limits of being (and the possibility of union with being) and a volanturism that makes the will curve in on itself and becomes its own object, the Augustinian curvatus in se, which reaches a limit in a sort of black hole-like event horizon of total self-absorption—Dostoevsky's Underground Man and Nietzsche's unstable late work being prime case studies here.
I am not even sure if Nietzsche would agree with his post-modern descendents here. Almost no philosopher spends more time blaming other philosophers or hurling value-laden invective.
Nor do I think the progenitors and partisans of Woke necessarily misunderstand their sources. Since we have no dyed in the wool Wokelati here, let's consider what their response might be to the criticisms in this thread:
They would probably say something similar, that the claim that their ideology/movement is flawed because it focuses too much on "moralizing," is itself a value judgement in line with cis white male normativity. Indeed, your list of names turned into adjectives proves their point; these are all cis white men of a past epoch who have failed to fully transcend their privilege. They are most useful in terms of the inspiration they gave to later feminist, indigenous, etc. thinkers, not in themselves.
They have not "failed to understand" by engaging in moralizing, they have simply rejected a cis white patriarchal normativity that pretends to oppose itself to value while also privileging its own hegemonic values. They would reject claims about spectral evidence or any "error of moralizing." Consider the language of anti-colonial revolutionaries or of figures like Malcom X and Martin Luther King. These are loaded with value. It has been this discourse that has helped liberate the oppressed. That fields dominated by white men find it to be, in a sense, "distasteful" or a "misunderstanding," is only due to their own biases. Understanding is constitutive, and identity is constitutive in the very possibility of understanding.
Hence, they are not misunderstanding the way knowledge and truth claims are constituted. Rather, their claims to epistemic priority on issues related to marginalized groups are based precisely on this understanding. It is why they have a privileged epistemic perspective. Intelligibility only exists within systems of discursive discourse. The idea that they are dealing primarily in emotion or "spectral evidence," or that they are engaged in inappropriate "moralizing" would itself be an attempt to privilege a white and male view that is uncomfortable with the assertiveness of values in feminine, indigenous, etc. terms.
Second, the claims in this thread that they are going "too far," or are guilty of alienation also itself privileges entrenched cis, white, patriarchal systems of dominance. The person facing systematic racism, systematic sexism, aggressions, etc. is being asked to simply accept that they suffer them longer, even after a lifetime of having suffered them, so as to not offend the sensibilities of the dominant group. The very focus on "productivity" in calling their methods "counterproductive" is white and male biased. Critics are also "on the wrong side of history," their criticism being akin to demands that Civil Rights Era activists or those struggling to overthrow colonialism "settle down" and "wait patiently." S
Since intelligibility is inherently bound up in community membership, those outside a community should practice good allyship by listening and being receptive. Certainly, they cannot point to a standard of "proper understanding" as set by a list of "canonical" old, cis, white, men, all from philosophy, a discourse totally dominated by whiteness and patriarchy, while calling extensions of those thinkers in much more diverse fields a sort of "error." This would be to miss that intelligibility only exists within those discourses and identities that lie external to their standards, which is a tyrannical attempt to absolutize their own normative standards (even if these are claimed to be "non-moralizing.")
Or, in summary, critics need to: Check. Their. Privilege. :clap: :clap: :clap:
Well, it isn't wrong about some things. But a problem here is that "experts on fascism" have been ringing the tocsin about immanent fascism for decade after decade. The Tea Party was immanent fascism, W. Bush was a fascist, the Clinton pivot to the center, Reagan, Nixon, 1950s consumerism and consensus, all a step from fascism. So, that takes some of the wind out of it. At the same time, the "Long March Through the Institutions" was in some sense a stunning success, but in other ways it was a failure in that it simply collapsed faith in/support for key institutions, particularly the justice system, media, and academy (in part because the ideology suggested just this sort of outcome).
It's certainly true that every Presidency now seems to involve unprecedented new acquisitions of power by the executive branch, and to a lesser extent the judiciary. This is because the legislative branch has become an extremely dysfunctional, and in many ways hated, institution. This is a phenomenon that goes beyond the particularities of US politics. Trump's second term might be exceptional in this regard, but it follows a long pattern. The centralization of power and lawlessness are longer term trends, but the video puts all the emphasis on Trump. I don't think that's necessarily off base. There are many issues more specific to Trump. But it's worth recalling that Marius, Sulla, etc. had to trample institutions and norms before there could be a Caesar or an Augustus. The "left" and "right" took turns destroying the Republic, and who ended up in control when all of the illusions finally broke down was largely an accident of history. The late Roman Republic strikes me as the right analogy here for a host of reasons. -
The End of Woke
Perhaps. If true though, I can't say I'm upset, as woke is a poison pill for ideology. Hopefully if the GoP doubles down on identity politics, this will swing us back to the middle again as they fracture their growing coalition.
I'm not sure if it will play out the same way. The noxious "White nationalist" faction notwithstanding, the fact that the GOP has embraced an identity politics of culture and tradition means that one can "convert" and be taken into the fold much more easily. One is not forced to sit outside the core of the movement as a mere "ally," but can become a leading protagonist in its story, which in turn spurs on more evangelical activity. The similarities lie more in the focus on identity, grievance, narratives of power, skepticism of institutions (instruments of power), and as Doyle puts it, "admission of spectral evidence," (i.e., personal feelings of grievance as indicative of moral wrong). There is also a similar distrust of scientific, journalistic, academic, etc. institutions as mere instruments of power, a sort of epistemology of power to go along with the metaphysics of power. The "nu-right" is a heavily aesthetic movement, drawing a lot from ancient epics and art, and so you also have an "aesthetics of power." The preference for classical art styles for instance, is not mere reactionary preference for the old, but obviously because these are taken to by symbols of imperial power and warrior spirit.
Sun and Steel is incredibly popular here for example, as well Jünger's Storm of Steel. Indeed, I think Musk has advocated both.
It's interesting to note too how much this bleeds into the "empirical sciences." The core anthropology still largely assumed for political economy (now economics and political science) was developed long before the statistical methods that helped the social sciences assert themselves alongside the physical sciences as "properly scientific." A lot of it was closer to armchair speculation (although obviously informed by powerful insights and observations). Yet the legacy has remained robust.
You can also see this in narratives in biology, or even the way nature documentaries get narrated. The reception of Darwin has remained ideologically charged. Consider the Lion King’s harmonious “Circle of Life” versus the famous 56th canto of Tennyson’s In Memoriam A.H.H.:
Man, her last work, who seem’d so fair,
Such splendid purpose in his eyes,
Who roll’d the psalm to wintry skies,
Who built him fanes of fruitless prayer,
Who trusted God was love indeed
And love Creation’s final law —
Tho’ Nature, red in tooth and claw
With ravine, shriek’d against his creed
One can hardly choose between the two simply on the basis of "scientific data." -
On Purpose
Enactivism can be consistent with more traditional Aristotleianism, Thomism (even of the existential variety), or more "Neoplatonic," thought, although it often isn't. Robert Sokolowski is an example of someone who is firmly within the tradition of phenomenology and enactivism and yet sees his work as consistent with Aristotle and Saint Thomas.
There is a slippery distinction here though. I'd like to say there are really multiple camps within phenomenology. They all take a lot from Husserl, but some stay closer to his original project and inclinations, some are more aligned with more broadly "post-modern" views and metaphysics (I know they don't consider them that), and then there are a lot of mostly, but not entirely, Catholic philosophers who see the project as consistent with realism (and tend to stress its Scholastic roots). But, all of them seem to have people who like enactivism, although they sometimes seem to draw quite different implications from it, or explain it in different ways, but the core themes and terms are the same.
None of them seem to have much use for Hegel's phenomenology.
Anyhow, to go back to the main topic, I think a difficulty in speaking about ends, telos, and human purpose is that terms like "transcendental," "absolute," and "platonic," which have come up in this thread, often get used in somewhat equivocal ways across traditions.
While goodness and truth are "transcendental properties of being," in the tradition coming out of (neo)Platonism and Aristotleianism, these are merely "logical/conceptual" distinctions. They don't add anything to being. They are being as considered from some particular perspective. Goodness (and so ends) is being considered in terms of desirability (i.e. the appetites, most appropriately, the will). Obviously, only living things have appetites, unless we speak in a quite analogous sense (e.g., "the air in a balloon 'wants' to expand"). So life and mind are quite relevant, in that only minds make conceptual distinctions and Good/True are conceptual distinctions. But conceptual distinctions also aren't arbitrary. This gets us a proper sort of perspectivism and relativism, without anti-realism re values and purpose.
Indeed, I am not sure you can have anti-realism re values without also ultimately having a sort of anti-realism re telos and purpose; they both become an illusion of sorts, leading physicalism back into a sort of mental/physical dualism. -
The End of Woke
To avoid replacing one form of tyranny with another, he advocates for a renewed commitment to liberalism and a revival of Enlightenment principles such as free speech, open debate, and individual liberty.
Sounds eminently sensible to me.
This is largely Fukuyama's answer in his recent "Liberalism and it's Discontents," which treats the same issue, but also the excesses of neoliberalism (which he sees as taking classical liberalism to an extreme, largely due to assumptions borrowed from economics).
Fukuyama is, at first glance, pretty open to critiques of the most popular forms of modern liberalism. He allows that the difficulty for Rawls and Nozick (major figures for progressive and conservative liberalism respectively) is that they have extremely "thin" anthropologies. Man is something of an atomized appetite machine who needs to be controlled by contracts and systems of "nudges and prompts," so that his inherent selfishness and gratification-seeking doesn't conflict with other's similar behavior (John Millbank's point, which is more radical, is that this is really just the Reformed theological tradition's view of man and nature, only with grace and God removed).
Fukuyama famously adds thymos back into the equation, arguing that people also seek a sense of honor and recognition, and that this is why identity fails to simply dissolve despite the pressures of capitalism and liberalism. He even makes an abortive appeal to virtue in the book. He compares a young woman going to school, supporting her sick mother, who is still actively politically involved, with a wealthier young man who spends all his time on video games and pornography, and is completely checked out of political life and any "common good." His point is that, pace earlier liberals, we can judge the former better, because we are judging based on their character, not based on immutable facts about them. He thinks we can make some judgements about the human good, even if only those goods that support liberalism itself. However, he doesn't really address the real issue, which is that a denial of human telos (or our ability to know it), makes virtue impossible to define.
Anyhow, I bring up Fukuyama because he is one of the better, fairer attempts to defend the view that what is needed is a return to classical Enlightenment liberalism. It's a short book too.
I think it is wrong though. I think Fukuyama errs because, while he finds a role for thymos, he ignores logos, that people want to do what they think is "truly best." Thymos alone doesn't explain rich men in the Roman empire giving up all their status and wealth and becoming celibates to pursue the monastic life or philosophy. It doesn't explain Marxist revolutionaries who were willing to take on suicidal missions to further what they saw as a path to a truly better society. Self-interest doesn't explain athiest martyrdom.
Fukuyama makes the Wars of Religion out to be entirely battles of thymos, when in reality they were, above all else, wars over logos. Likewise, even on smaller scales, people don't abandon good careers, status (thymos), and consumption to do things like care for sick parents as a means of pursuing thymos or epithumia (pleasure), they do it because it agrees with logos, i.e., what they believe "a good person should do." One need not even suppose any "objective good," for this to be true, one only need allow that people's notion of goodness is often the deciding factor in what they choose to pursue, and that it often trumps pleasure and status seeking.
This is a big miss because the classical liberalism Fukuyama defends is arguably most choice-worthy precisely because the "marketplace of ideas," and universal education it fosters allows people to best fulfill the desires of logos by allowing them to explore goodness and virtue. Arguably, this oversight could actually be used to support "classical liberalism" against its competitors.
I am more skeptical. I think that classical liberalism is largely defined by its anthropology, so that any system with an appropriate role for thymos and logos probably becomes something quite different. However, this doesn't mean it jettisons the things Fukuyama thinks are most valuable about liberalism, namely:
1. Accountable government (normally through some form of elections)
2. A strong, independent, professional civil society
3. A centralized state monopoly on force
4. Rule of law and property rights
So, whether you'd want to call a reform based on a "thick" anthropology "liberalism" or not seems besides the main point to me.
To bring it back to Woke though, the post-modern thread Fukuyama objects to likewise suffers from this thin anthropology because it is an outgrowth of liberalism itself. I think it's also unable to take logos seriously. The "metaphysics of power" normally tends to dissolve the subject possessing logos, and to make logos merely an illusion of power, or nothing but power itself. However, I don't think the foundations of this movement are actually philosophically sound, and even if they were, their logical conclusion will be fascism (what we are indeed seeing), not some sort of radically left egalitarianism. -
The End of Woke
Thus, contemporary configurations of power can quickly neutralize and absorb emancipatory movements, turning them into administrable forms of identity within broader regimes of control.
Or it can make old identities new targets of power. In the context of the Great Awokening, gender seems most relevant (although religion is important too, "Evangelical" sometimes gets used almost like a slur). In the US context, the Democratic party seems to have a "young male" problem, with voters under 20 being more likely to vote for Trump than those over 75, and Trump winning the male Latino vote. This goes along with the marked "gender gap" in political ideology that has been observed across developed countries. I cannot help but think that this is because broad aspects of male identity have been deemed "problematic" by some vocal social reformers. That is, presumably "toxic masculinity," is just about the toxic parts of masculinity, which certainly exist, but there are obviously very important disagreements about which aspects these are.
I am not very big on the whole "War on Boys" narrative, although it does seem to touch on some real issues. I'd prefer to frame it as more an issue of the "education of the chest," that C.S. Lewis speaks of in The Abolition of Man, i.e., the training of the passions and the "spirited part of the soul," thymos. The problems Lewis identified only seem to have gotten worse, and they obviously affect women as well. My point would be that men raised to be "men without chests," become desperate for some sort of thymotic influence. Thus, they end up being easy prey for precisely the sort of "toxic masculinity" reformers want to abolish. So it ends up being a sort of self-reinforcing cycle.
Edit: note the timing
Count Timothy von Icarus

Start FollowingSend a Message
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum

