Just to be absolutely clear, I am saying that since it is false that "No people are not dinosaurs." it must necessarily be true that at least one person is not a dinosaur. And thus that there are no people is incompatible with the given premise.

... all what have a horn and no horn?? — bongo fury

Try this. If I have no money, I have no money in my left pocket, and no money in my right pocket. So where's all my money? In my pockets, obviously. So I take all my money and give it to you and because I have no money I give you nothing, and I still have no money in my pockets, even though I just gave you all the money in my pockets.

Just to be absolutely clear, I am saying that since it is false that "No people are not dinosaurs." it must necessarily be true that at least one person is not a dinosaur. And thus that there are no people is incompatible with the given premise. — unenlightened

Yep, I get it :lol: thanks :pray:

'No people are not dinosaurs' means that all people are dinosaurs. If this statement is not true, it could be that it is not true because either no people are dinosaurs or not all people are dinosaurs.

On the first reading, that no people are dinosaurs, only C "some people are not dinosaurs" would be consistent and hence true, even though it would be understating the case.

On the second reading, that not all people are dinosaurs, A: "some dinosaurs are people", C: "some people are not dinosaurs" and D: "No dinosaurs are not people" might be true, and B: "All people are dinosaurs" would be false.

On consistency, a couple of visuals:




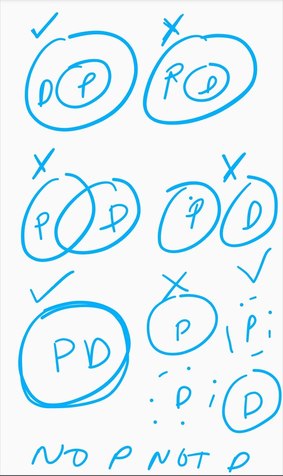
The first pic, the statement. The second, the negated consistencies with A-D.

[Edit: Fixed visual typo]
[Edit 2: Fixed as per @bongo fury]

I think (but could be wrong again of course) that you've got your choice of tick/cross on your final scenario wrong in each frame? I'm reading that final pic each time as "no people, some dinosaurs"? I.e. dotted circle meaning no people?

Aren't we all agreed we are allowed to read "no people are dinosaurs" as allowing for there being no people?

Perhaps not, if we take Aristotle's alleged stance?

But as others (including @snakes alive and @unenlightened) pointed out correctly, we don't need to, to prove option (c)?

Also, what about "no people, no dinosaurs"?

Cheers. Fixed. (No room for no P no D :sad: )



:lol:

@Alexis Schaffer

"No people are not dinosaurs"

No people = There doesn't exist an 'x' that meets the criteria.

There doesn't exists a person which is not a dinosaur.

This equals to:

Everyone is a dinosaur.

And this is false, which means:

There is at least one person who is not a dinosaur.

A) Some dinosaurs are people
B) All people are dinosaurs
C) Some people are not dinosaurs
D) No dinosaurs are not people

Neither A or D suffice because you never said that a dinosaur can be people / a person.
B is false, as there is someone who's not a dinosaur.
C is true, as it is compatible with our statement.

:up:

C) Some people are not dinosaurs — Alexis Schaffer

A) Some dinosaurs are people
B) All people are dinosaurs
D No dinosaurs are not people = dinosaurs are people

that leaves C as the one that dose not fit.

This user has been deleted and all their posts removed.

This user has been deleted and all their posts removed.

I was reminded of this (for me) very embarrassing thread when quoting Quine here:

But the configuration of prefixes '~∀x~' figures so prominently in subsequent developments that it is convenient to adopt a condensed notation for it; the customary one is '∃x', which we may read 'there is something that'.
— Quine, Mathematical Logic — bongo fury

the argument itself does not grant people. — tim wood

Doesn't it at least deny:

1) ∀x~(Px & ~Dx)

I.e. for all choices of x, no personhood without dinosaurhood?

And wouldn't that denial:

2) ~∀x~(Px & ~Dx)

i.e. for fewer than all choices of x, no personhood without dinosaurhood

... seem to suggest that for some one or more remaining choices of x, personhood without dinosaurhood? ... i.e.,

∃x(Px & ~Dx)

as per Quine's definition?

Oh good. Someone found the No-cat I was talking about a few days ago. Was wondering when that would happen.

This user has been deleted and all their posts removed.

if the false proposition were that no yurgs were not dinosaurs, then you're in the position of affirming the existence of yurgs. — tim wood

Yes, being asked to deny the non-existence of yurgs of a certain type is being asked to affirm their existence, surely?

If you are disconcerted by that step, maybe you (like me, often) slipped into thinking the invitation was to deny, instead, some spurious inference to the existence of yurgs of the opposite type?

This user has been deleted and all their posts removed.

C follows immediately. — JosephS

:up:

I'd really appreciate it if you could also briefly discuss your thought process as you solved it! — Alexis Schaffer

I solve visually. Part of my work. I can't.

Yes? When was the last time you saw a yurg? — tim wood

You wrote the puzzle :wink:

No yurgs are green, or, all yurgs are blue, on the other hand, cannot be presumed to imply there are any yurgs. — tim wood

:up: Cool, e.g.,

For all choices of x, not yurg without blue. (Could be zero yurgs.)

you would have them as proof of the existence of yurgs. — tim wood

No, but their negations, yes. E.g.,

For fewer than all choices of x, not yurg without blue... hence, for some one or more remaining choices of x, yurg without blue.

~∀x~(Yx & ~Bx) => ∃x(Yx & ~Bx)

By the way, though, also the green:

~∀x~(Yx & Gx) => ∃x(Yx & Gx)

Or even just non-yurg:

~∀x~(Yx) => ∃x(Yx)

and

~∀x(Yx) => ∃x(~Yx)

Also of course

∀x(Yx) => ∃x(Yx)

I.e. a universally quantified conditional (just like a universal categorical) needn't imply existence ('import') of the type of object named in the antecedent; but the quantifier itself always refers to the whole universe of assumed entities, and hence always facilitates implication of some existential statement or other.

All choices are wrong because there aren't any dinosaurs to use for comparison.