-
 Andrew M
1.6kUnderlying both DEs is the fundamental relationship: The instantaneous rate of change of something is proportional to the amount at that time. The first DE has the imaginary i in its "constant", and eiθ=cos(θ)+isin(θ)eiθ=cos(θ)+isin(θ) works its magic. — jgill
Andrew M
1.6kUnderlying both DEs is the fundamental relationship: The instantaneous rate of change of something is proportional to the amount at that time. The first DE has the imaginary i in its "constant", and eiθ=cos(θ)+isin(θ)eiθ=cos(θ)+isin(θ) works its magic. — jgill
So I opened an account at the bank with $100 at an imaginary 314% interest rate. A year later, the bank claims I owe them $100! They say that if I keep my account open for another year, I'll get my $100 back. Should I trust them, or just pay the $100 and close the account?
Edit: The interest rate should be 314% (not 3.14%) -
 Kenosha Kid
3.2kThanks, that's pretty well the only point I was getting at. — Wayfarer
Kenosha Kid
3.2kThanks, that's pretty well the only point I was getting at. — Wayfarer
Oh okay. Ha ha!
I'm not sure I follow your last sentence (and I read the SEP section). If, on measurement, the superposition state information is lost to the environment (apart from the measured value) then what else could be required for apparent collapse to have occurred? — Andrew M
Decoherence leads to random phases between different trajectories. You can't guarantee a singular value upon measurement without invoking e.g. MWI. But nor do e.g. excluded or otherwise saturated points so thank you for bringing it up. It is yet another physical consideration ignored in the idealised screen that will eliminate possibilities in a way dependent upon the exact state of the lab equipment.
This is just unitary QM. For example, MWI and RQM both agree with this prediction and are both referenced in the paper you linked — Andrew M
Yes, I was thinking about this afterwards. I was thinking that in MWI the second observer would just branch. I'm not sure what the consensus is on branching during non-destructive measurement, but thinking about it you're probably right. Essentially a branch in MWI is just an additional term in the MB wavefunction. Whether the maths tells us branching has occurred will be time-dependent.
Zurek is a decoherence guy and he agrees with the Wigner's friend predictions. You seem to be treating decoherence as objective. — Andrew M
Decoherence usually is. Reading the full paper, Zurek is saying that, in light of the recent Wigner's friend type experiments, it's not. I'm not so sure about that. He's solving a problem that probably doesn't need to be solved from either end. Generally decoherence is treated objectively and is insufficient to yield collapse
Thanks for the link, btw. I hadn't read that paper. I kind of wish I had before submitting a follow up thread to this. -
 Metaphysician Undercover
14.3kDecoherence usually is. — Kenosha Kid
Metaphysician Undercover
14.3kDecoherence usually is. — Kenosha Kid
"Decoherence" is fundamentally flawed. It assumes coherence as the natural, observer independent condition of existence. But coherence is the property of a "system". Such a system is either completely artificial, or an arbitrary designation, or a combination of these two. In both cases, it's a human construct. Therefore coherence is a manufactured condition, either as a physically constructed system, or as an arbitrarily applied theoretical ideal. It is self-contradictory to conceive of coherence as the independent state of existence, when it is clearly a manufactured condition. -
 Kenosha Kid
3.2k
Kenosha Kid
3.2k -
 SophistiCat
2.3kSo I went back to Cramer's papers from 1980s onward in an attempt to gain a better understanding of the transactional interpretation. I think I managed to unconfuse myself a bit regarding the "orthodox" TI, but I am still not sure about your take on it.
SophistiCat
2.3kSo I went back to Cramer's papers from 1980s onward in an attempt to gain a better understanding of the transactional interpretation. I think I managed to unconfuse myself a bit regarding the "orthodox" TI, but I am still not sure about your take on it.
The core of the theory is an emission-absorption process, such as when two atoms exchange energy or (as in your presentation) an electron is emitted and later absorbed by a solid. (I think scattering is handled similarly, but I haven't looked into it yet. There is also an issue of weakly-absorbed particles, such as neutrinos, which may not have a future boundary; I know that Cramer has looked into this, but I haven't.)
The process is initiated by a "transaction" between the emitter and the absorber, which is described by the following reversible pseudo-time sequence:
- The emitter produces two half-amplitude waves: a retarded wave going in the forward time direction and an advanced wave going in the backwards time direction (with a negative
energyenergy eigenvalues and phase-shifted by 180 degrees). - The absorber is "stimulated" by the retarded wave from the emitter (offer wave) and also produces a pair of half-amplitude waves going in opposite time directions. The advanced wave from the absorber (confirmation wave) reaches back in time towards the emitter.
- When there are multiple potential absorbers, they all produce confirmation waves with amplitudes proportional to the amplitude of the offer wave. The absorber is selected randomly, with the probability of selection weighted by the square of the amplitude - this is where the Born rule comes into play.
- Once the emitter and the absorber "handshake" (which is sometimes described by another pseudo-process, which I haven't investigated), the "tails" of the wavefunctions going back in time from the emitter and forward in time from the absorber cancel out, as are the imaginary parts of the waves between them, leaving only the superposed real parts of the offer and confirmation waves. To any observer this will look as if a wave traveled from the emitter to the absorber.
(I hope I got this right.)
The above is offered as an interpretation of the standard quantum mechanics formalism, rather than some additional physics. The steps do not describe a causal time sequence of events - they merely serve a pedagogical purpose.
The rationale for the interpretation comes from the fact that, as KK mentioned, some relativistic formulations of the wavefunction equation have two solutions: w and its complex conjugate w*. But complex conjugation is equivalent to time reversal (although it also implies negative frequency, energy and charge) - hence the advanced wave that is produced alongside the retarded wave in TI. Plus the Born rule, etc. - the conjugate of the wavefunction is ubiquitous in the formalism.
It is interesting that both the TI and the Everett MWI start from similar philosophical positions. Both are realist about the wavefunction (in contrast to Copenhagen). Both are offered as minimalistic interpretations that do nothing more than take the math seriously (as Sean Carroll, an Everettian, puts it). "From one point of view, the transactional interpretation is so apparent in the Schrodinger-Dirac form of the quantum-mechanical formalism..., that one might fairly ask why this obvious interpretation of the formalism had not been made previously" (Cramer). But they still end up in very different places. To me it seems like TI goes further out on a limb than MWI. I am uncomfortable about the pseudo-causal narrative of the "transaction." But perhaps the more profound aspects of the interpretation escape me.
This bit I don't understand though:
Because the electron's birth and death are the true boundary conditions of its wavefunction! And here we turn to relativity. The relativistic wavefunction is of the form [E−V]2=[p−A]2+m2. This puts time and space on equal footing (both energy and momentum are squared), requiring knowledge of the particle at two times, not just one. — Kenosha Kid
Whether it's the non-relativistic Schrodinger equation (which has only a retarded solution) or a suitable relativistic equation (which has both), the equation alone does not determine where and how the absorption/measurement will happen - hence the "measurement problem." I am not sure what point is being made here specifically about the relativistic equation.
(The Schrodinger equation can be produced as a non-relativistic limit of a more general relativistic formulation. How then do two solutions reduce to one? Turns out that two versions of the Schrodinger equation are equally valid reductions: the other one has only an advanced solution.)
Now, as for the "not many worlds" interpretation:
There is no guarantee here that this will eventually reduce the number of intersections with the screen to 1. But we are a long way from the original Copenhagen picture of an electron that might be found anywhere. I expect that, if we could solve the many-body Dirac equation for the universe (well, it would have to be some cosmologically-consistent generalisation of it), it probably would resolve to 1 intersection. — Kenosha Kid
I still don't see how this can be. Boundary conditions are, by definition, local. And yet when we do experiments like quantum interference, we find that the measurements depend mainly on the incident wave. Why aren't results confounded by such strong dependence on the boundary conditions? - The emitter produces two half-amplitude waves: a retarded wave going in the forward time direction and an advanced wave going in the backwards time direction (with a negative
-
 Kenosha Kid
3.2k(I hope I got this right.) — SophistiCat
Kenosha Kid
3.2k(I hope I got this right.) — SophistiCat
Damn straight!
some relativistic formulations of the wavefunction equation have two solutions: w and its complex conjugate w* — SophistiCat
All, I believe.
But complex conjugation is equivalent to time reversal (although it also implies negative frequency, energy and charge) — SophistiCat
Time, frequency and energy are all inextricably linked. Essentially energy is frequency with decorative physical constants, and is reciprocal to time (time interval and frequency are Fourier transforms of one another). So the odd one out here is charge.
To me it seems like TI goes further out on a limb than MWI. I am uncomfortable about the pseudo-causal narrative of the "transaction." But perhaps the more profound aspects of the interpretation escape me. — SophistiCat
I think it's more economical than parallel universes. But it doesn't resolve the measurement problem by itself. Since the transaction goes both ways, the final state is "known" at the start, so it is still probabilistic, however nothing real collapses probabilistically.
Whether it's the non-relativistic Schrodinger equation (which has only a retarded solution) or a suitable relativistic equation (which has both), the equation alone does not determine where and how the absorption/measurement will happen - hence the "measurement problem." I am not sure what point is being made here specifically about the relativistic equation. — SophistiCat
So this is where the theoretical background ends and my argument begins. Taking the Dirac equation and TQM as gives, we understand that in order for an electron described by a particular time-dependent wavefunction to be emitted by the cathode and absorbed by a point on the screen, the cathode must itself be in a state that would emit an electron with that wavefunction, i.e. it must have an available electron to emit.
The advanced wave must be similarly causal but in reverse. For an advanced wave to be emitted from a particular point on the screen (describing an electron hole in reverse), that point must be capable of doing so. Otherwise the retarded wavefunction depiction of the electron leaving the cathode is unjustified in the first place.
That state also has its own history in our future (recalling that QM is backwards-deterministic) and we can repeat this process by considering events in that future history that are consistent with the future of the electron. This can only eliminate possible locations on the screen.
Andrew M has pointed out that other factors that equally depend on the precise state of the set-up should also eliminate possible paths, namely those where the eventually phase randomisations due to scattering in the screen cause destructive interference.
These are factors of the true time-dependent many-body wavefunction that describes the entire experimental setup that a) we couldn't possibly solve and b) we couldn't possibly know the accuracy of (we can't know the precise state of a macroscopic object even if we could store it's wavefunction in principle).
This aspect of the argument is not dependent on relativistic TQM, rather the latter provides us with an obvious way to consider how these incalculable states will inevitably lead to certain trajectories becoming disallowed when we consider not just the past but also the future of the experiment.
(The Schrodinger equation can be produced as a non-relativistic limit of a more general relativistic formulation. How then do two solutions reduce to one? Turns out that two versions of the Schrodinger equation are equally valid reductions: the other one has only an advanced solution.) — SophistiCat
Correct, the conjugate of a solution is not itself a solution. However you can still time-evolve that conjugate and it will behave as expected, traveling in the reverse direction to the solution.
I still don't see how this can be. Boundary conditions are, by definition, local. — SophistiCat
No, not necessarily. We treat them as local because we treat experiments ideally: we have to.
And yet when we do experiments like quantum interference, we find that the measurements depend mainly on the incident wave. Why aren't results confounded by such strong dependence on the boundary conditions? — SophistiCat
In the case of both microstate exploration and decoherence, the precise state changes constantly. An electron on the screen which might forbid an incident electron at time t might not be present at time t'. A particular configuration of scatterers that would destroy the wavefunction at time t might permit it at time t'.
The signature pattern of the double slit experiment is not one event but many thousands. What we see then is not just the value of the probability density of the electron, but also the statistical behaviour of the macroscopic screen. Over a statistical number of events, the pattern must be independent of changes in the precise microstate of the screen. -
 jgill
4kI think it's more economical than parallel universes. — Kenosha Kid
jgill
4kI think it's more economical than parallel universes. — Kenosha Kid
Here's a PU in case you haven't seen one before:
:cool: -
 Andrew M
1.6kFor anyone curious about the puzzle I presented earlier...
Andrew M
1.6kFor anyone curious about the puzzle I presented earlier...
So I opened an account at the bank with $100 at an imaginary 314% interest rate. A year later, the bank claims I owe them $100! They say that if I keep my account open for another year, I'll get my $100 back. Should I trust them, or just pay the $100 and close the account? — Andrew M
...The short answer is, yes, I can trust them. Here's the worked out solution.
Euler's formula is:
e represents continuous growth starting from 1, at a rate indicated by the exponent. If the growth rate is 0, we remain at the starting point (i.e., e^0 = 1). Similarly, starting from $100, we remain at $100 (i.e., 100 * e^0 = 100 * 1 = 100).
While real exponential growth occurs along the real number line, imaginary growth is circular. That is, it can be visualized as rotation around the origin of the complex plane (where the real number line is horizontal and the imaginary number line is vertical).
From the problem above, a 314% interest rate is a growth rate of 3.14, or (approximately) . Plugging that rate into the formula, we get
which is Euler's identity. So, starting with $100, I ended up with -$100 (i.e., 100 * e^i.pi = 100 * -1 = -100). Not a great outcome! But suppose I leave my account open for another year. That is a rate of pi for two years, or 2pi. Plugging that rate into the formula, we get:
So starting with $100, I'll end up with $100 (i.e., 100 * e^i.2pi = 100 * 1 = 100). That is, I'll get my original money back. 2pi radians, of course, is 360° - the money has gone full circle.
So the best strategy is to borrow lots of money at an imaginary interest rate, wait until it becomes positive (a 180° rotation), and then withdraw it. -
 SophistiCat
2.3ksome relativistic formulations of the wavefunction equation have two solutions: w and its complex conjugate w* — SophistiCat
SophistiCat
2.3ksome relativistic formulations of the wavefunction equation have two solutions: w and its complex conjugate w* — SophistiCat
All, I believe. — Kenosha Kid
I was hedging because in his 1980 Phys. Rev. D paper Cramer writes that for particles with spin other than 1/2, e.g. bosons, there are a number of alternative relativistically invariant wave equations, at least one of which is first order in time. I don't think he considered the full QFT formulation though.
The advanced wave must be similarly causal but in reverse. For an advanced wave to be emitted from a particular point on the screen (describing an electron hole in reverse), that point must be capable of doing so. Otherwise the retarded wavefunction depiction of the electron leaving the cathode is unjustified in the first place. — Kenosha Kid
Are there really any absolutely forbidden points of interaction? Instead of being absorbed, can't the electron scatter instead?
In the case of both microstate exploration and decoherence, the precise state changes constantly. An electron on the screen which might forbid an incident electron at time t might not be present at time t'. A particular configuration of scatterers that would destroy the wavefunction at time t might permit it at time t'.
The signature pattern of the double slit experiment is not one event but many thousands. What we see then is not just the value of the probability density of the electron, but also the statistical behaviour of the macroscopic screen. Over a statistical number of events, the pattern must be independent of changes in the precise microstate of the screen. — Kenosha Kid
So let's consider a limiting case where exactly one spot on the screen is available for interaction at any one time - an advance electron hole, as it were. This is what you hypothesize might indeed be the case, right? We can fairly assume that such points are uniformly distributed over the surface of the screen*. If the availability of electron holes imposes an absolute constraint on where an interaction can occur, then instead of the interference pattern we should see just that - a uniform distribution.
* Or in any case, we can't expect their distribution to coincide with the amplitude of the incident wave.
I think it's more economical than parallel universes. — Kenosha Kid
Well, if I understand correctly, the Everett interpretation is characterized more by what it doesn't do - arbitrarily impose a collapse - than by what it does, so in a way it's hard to be more economical than that (although it does ditch those advanced solutions. Hm... could you combine the two?...) But I didn't mean to imply that parsimony and fidelity to the formalism are the only or the most important criteria in evaluating philosophical interpretations. I am rather ambivalent on that point. -
 Kenosha Kid
3.2kthere are a number of alternative relativistically invariant wave equations, at least one of which is first order in time — SophistiCat
Kenosha Kid
3.2kthere are a number of alternative relativistically invariant wave equations, at least one of which is first order in time — SophistiCat
But also first order in space, I think? So the four solutions (advanced spin up, advanced spin down, retarded spin up, retarded spin down) reduce to two (advanced and retarded).
Are there really any absolutely forbidden points of interaction? Instead of being absorbed, can't the electron scatter instead? — SophistiCat
Sure, but scattering also obeys the Pauli exclusion principle, so there must be two holes: one for the scattering electron to go to, and one for the scattered electron to go to (see the Feynman diagram in the OP). And those scattered electrons can scatter again, each requiring two holes, and so on and so forth. So it's a proliferation of backwards hole emission and transmission events.
So let's consider a limiting case where exactly one spot on the screen is available for interaction at any one time - an advance electron hole, as it were. This is what you hypothesize might indeed be the case, right? — SophistiCat
Possibly but not necessarily, hence the not many worlds interpretation :) The electron wavefunction at the screen yields the possible trajectories of the electron independent of the state of the universe and its future history. The possible trajectories can only be eliminated, not added to, by incorporating more and more of the instantaneous state of the screen and more and more of the future of the system.
Does it reduce to one? Not necessarily. Thing is, we can't know, since knowing for sure means solving the time-dependent many-body Dirac equation or some good approximation thereto, not just for the lifetime of the experiment but for the lifetime of the particle.
If the availability of electron holes imposes an absolute constraint on where an interaction can occur, then instead of the interference pattern we should see just that - a uniform distribution. — SophistiCat
No, because the probability distribution of the incident electron will still multiply the probability distribution of acceptor sites.
Well, if I understand correctly, the Everett interpretation is characterized more by what it doesn't do - arbitrarily impose a collapse - than by what it does, so in a way it's hard to be more economical than that — SophistiCat
Fair point, it basically just runs with the mathematics, to its credit. Which is why I think MWI is an improvement over Copenhagen.
although it does ditch those advanced solutions. Hm... could you combine the two? — SophistiCat
Isn't that the not many worlds interpretation? -
 SophistiCat
2.3kthere are a number of alternative relativistically invariant wave equations, at least one of which is first order in time — SophistiCat
SophistiCat
2.3kthere are a number of alternative relativistically invariant wave equations, at least one of which is first order in time — SophistiCat
But also first order in space, I think? So the four solutions (advanced spin up, advanced spin down, retarded spin up, retarded spin down) reduce to two (advanced and retarded). — Kenosha Kid
He mentions a "relativistic Schrodinger equation," which has has a (P2 + m2)1/2 term.
Sure, but scattering also obeys the Pauli exclusion principle, so there must be two holes: one for the scattering electron to go to, and one for the scattered electron to go to (see the Feynman diagram in the OP). And those scattered electrons can scatter again, each requiring two holes, and so on and so forth. So it's a proliferation of backwards hole emission and transmission events. — Kenosha Kid
Does the incoming electron always scatter on another electron? If it's a solid that it interacts with, wouldn't it be something more complicated? Anyway, let's run with the assumption that in any type of interaction the electron is constrained by the requirement of having a (potentially infinite) chain of suitable boundary conditions.
No, because the probability distribution of the incident electron will still multiply the probability distribution of acceptor sites. — Kenosha Kid
Not if there is only one available site - in this case the electron wavefunction becomes irrelevant. The electron wavefunction removes at most a measure-zero amount of potential interaction sites, so without loss of generality we can assume that for any incoming electron, every point on the screen is available before we consider the conditions at the screen. But if the conditions at the screen are such that only one site is available, then that is what will dictate the actual distribution of impacts, not the electron wavefunction.
In case of "not many" available sites, the impacting distribution will factor in, but it will be smeared. -
 Andrew M
1.6k@Kenosha Kid
Andrew M
1.6k@Kenosha Kid
Once the emitter and the absorber "handshake" (which is sometimes described by another pseudo-process, which I haven't investigated), the "tails" of the wavefunctions going back in time from the emitter and forward in time from the absorber cancel out, as are the imaginary parts of the waves between them, leaving only the superposed real parts of the offer and confirmation waves. To any observer this will look as if a wave traveled from the emitter to the absorber. — SophistiCat
So that is how it appears to an observer. What actually happens once a handshake has occurred, and thus a single destination has been determined? In the double-slit experiment, does the electron follow a definite, albeit unknown, trajectory through one and only one of the slits, similar to pilot wave theory? Or does an electron essentially just disappear from the source and appear at the destination a short time later (a kind of non-local electron/hole exchange)? Or does it travel all possible paths to the destination as a wave? -
 SophistiCat
2.3kIn TI the electron is still essentially its wavefunction, right until its inglorious end. So it will go through both slits, interact with itself, etc. The twist is that there are two interacting wavefunctions - retarded and advanced. You can go with Feynman path integral formulation for the wavefunctions (as I think would be @Kenosha Kid's choice), but that is detachable from TI itself.
SophistiCat
2.3kIn TI the electron is still essentially its wavefunction, right until its inglorious end. So it will go through both slits, interact with itself, etc. The twist is that there are two interacting wavefunctions - retarded and advanced. You can go with Feynman path integral formulation for the wavefunctions (as I think would be @Kenosha Kid's choice), but that is detachable from TI itself.
It being a wave (waves) takes care of some well-known interpretational challenges that trade on the wave-particle ambiguity, like delayed choice and quantum eraser. That said, some challenges have also been proposed that are specific to TI, such as the quantum liar experiment. -
 Kenosha Kid
3.2kIn the double-slit experiment, does the electron follow a definite, albeit unknown, trajectory through one and only one of the slits, similar to pilot wave theory? Or does an electron essentially just disappear from the source and appear at the destination a short time later (a kind of non-local electron/hole exchange)? Or does it travel all possible paths to the destination as a wave? — Andrew M
Kenosha Kid
3.2kIn the double-slit experiment, does the electron follow a definite, albeit unknown, trajectory through one and only one of the slits, similar to pilot wave theory? Or does an electron essentially just disappear from the source and appear at the destination a short time later (a kind of non-local electron/hole exchange)? Or does it travel all possible paths to the destination as a wave? — Andrew M
The last one. The retarded wavefunction is as per the Copenhagen interpretation but without collapse. -
 Kenosha Kid
3.2kNot if there is only one available site - in this case the electron wavefunction becomes irrelevant. — SophistiCat
Kenosha Kid
3.2kNot if there is only one available site - in this case the electron wavefunction becomes irrelevant. — SophistiCat
Ah, it becomes emitted or not emitted in a time-dependent way (i.e. the longer the experiment, the higher the probability of transmission).
So that is how it appears to an observer. What actually happens once a handshake has occurred, and thus a single destination has been determined? — Andrew M
Just to clarify, the transactional interpretation doesn't itself eliminate probabilism, it just eliminates collapse. If the electron could end up in multiple possible final states, the state is still chosen probabilistically, but the electron "knows" in advance which it has shaken hands with.
The elimination of final states is an additional physical consideration that takes seriously the time-reversibility of the equations. In order for a particular site to receive the electron, it must be in a state capable of emitting an advanced wave. -
 Kenosha Kid
3.2kSo the best strategy is to borrow lots of money at an imaginary interest rate, wait until it becomes positive (a 180° rotation), and then withdraw it. — Andrew M
Kenosha Kid
3.2kSo the best strategy is to borrow lots of money at an imaginary interest rate, wait until it becomes positive (a 180° rotation), and then withdraw it. — Andrew M
:rofl: Sound financial advice!
So I went back to Cramer's papers from 1980s onward in an attempt to gain a better understanding of the transactional interpretation. I think I managed to unconfuse myself a bit regarding the "orthodox" TI, but I am still not sure about your take on it.
The core of the theory is an emission-absorption process, such as when two atoms exchange energy or (as in your presentation) an electron is emitted and later absorbed by a solid. (I think scattering is handled similarly, but I haven't looked into it yet. There is also an issue of weakly-absorbed particles, such as neutrinos, which may not have a future boundary; I know that Cramer has looked into this, but I haven't.) — SophistiCat
I was just rereading part of the paper on type II emission and absorption events, which are interesting. If we take the emitter to be atom 1, the absorber to be atom 2, and the emission to be a photon, from the lab frame it appears as a photon (its own antiparticle) from the origin is absorbed by atom 1 (the emitter) and then, seemingly unrelatedly, atom 2 emits a photon of the same energy, which continues forever.
If the distance between the two atoms is L, the time between perceived absorption and emission (actually emission and absorption) will be L/c. So if type II is possible, it ought to be detectable experimentally in principle, though in practice it would be hard if the phenomenon is limited to CMB radiation (assuming that's all that could constitute a photon from the origin).
What's more interesting is the idea that causal relationships between events can be apparently unmediated in principle. -
 Kenosha Kid
3.2kNot sure this should really be up there, but a copy of the entirety of Cramer's book The Transactional Interpretation of Quantum Mechanics is on my alma mater's website: https://www-users.york.ac.uk/~mijp1/transaction/TI_toc.html
Kenosha Kid
3.2kNot sure this should really be up there, but a copy of the entirety of Cramer's book The Transactional Interpretation of Quantum Mechanics is on my alma mater's website: https://www-users.york.ac.uk/~mijp1/transaction/TI_toc.html -
 Kenosha Kid
3.2kAnother paper by Cramer (Foundations of Physics, 1973) specifically treating the arrow of time: http://faculty.washington.edu/jcramer/TI/The_Arrow_of_EM_Time.pdf
Kenosha Kid
3.2kAnother paper by Cramer (Foundations of Physics, 1973) specifically treating the arrow of time: http://faculty.washington.edu/jcramer/TI/The_Arrow_of_EM_Time.pdf -
 Metaphysician Undercover
14.3kAnother paper by Cramer (Foundations of Physics, 1973) specifically treating the arrow of time: — Kenosha Kid
Metaphysician Undercover
14.3kAnother paper by Cramer (Foundations of Physics, 1973) specifically treating the arrow of time: — Kenosha Kid
However, there is an alternative approach which, while not in the mainstream
of contemporary theory, represents an effective way of preserving the intrinsic
time symmetry of the relativistically invariant wave equations and thereby
avoiding the ad hoc insertion of an arrow of time into the formalism. — John Cramer
Does this man seriously think that the insertion of an arrow of time is "ad hoc"? -
 Kenosha Kid
3.2kNot sure this should really be up there, but a copy of the entirety of Cramer's book The Transactional Interpretation of Quantum Mechanics is on my alma mater's website: https://www-users.york.ac.uk/~mijp1/transaction/TI_toc.html — Kenosha Kid
Kenosha Kid
3.2kNot sure this should really be up there, but a copy of the entirety of Cramer's book The Transactional Interpretation of Quantum Mechanics is on my alma mater's website: https://www-users.york.ac.uk/~mijp1/transaction/TI_toc.html — Kenosha Kid
From the above:
However, the careful reader will perceive that there is a more subtle time asymmetry implicit in the TI description of the quantum event which is implicit in TI2. There the probability of a quantum event with emission from (R1,T1) to an absorber at (R2,T2) is assumed to be:
P12 = |Psi1(R2,T2)|2 [12]
rather than:
P12 = |Psi2(R1,T1)|2 [13]
i.e., in the TI the emitter is given a privileged role because it is the echo received by the emitter which precipitates the transaction rather than that received by the absorber. Thus the past determines the future (in a statistical way) rather than the future determining the past. — Cramer
The above basically says that the probability of transmission of a photon or electron or whatever is given by the value of the retarded wavefunction spreading out from the emitter at the absorption event, not the value of the advanced wavefunction spreading out from the absorber at the emission event.
This is where the OP and the TI diverge, since the former states that knowledge of the future state of the screen would definitely disallow certain transmissions for which P is nonzero, i.e. the Born rule only tells us about the emission, while the electron knows about the absorption already, being stimulated by that absorption to the equal extent that the absorption is stimulated by the emission.
Hence transactional QM itself remains probabilistic, even as it dispenses with collapse.
Cramer claims to have mapped the above arrow of time to the cosmological arrow in the paper here:
Another paper by Cramer (Foundations of Physics, 1973) specifically treating the arrow of time: http://faculty.washington.edu/jcramer/TI/The_Arrow_of_EM_Time.pdf — Kenosha Kid
However the more I read it the less compelling I find it. He actually talks about advanced waves going forward in time, which is contrary to what an advanced wave is.
A good way to conceive of why the OP says this isn't true is to consider single-electron transistors in quantum electronics.
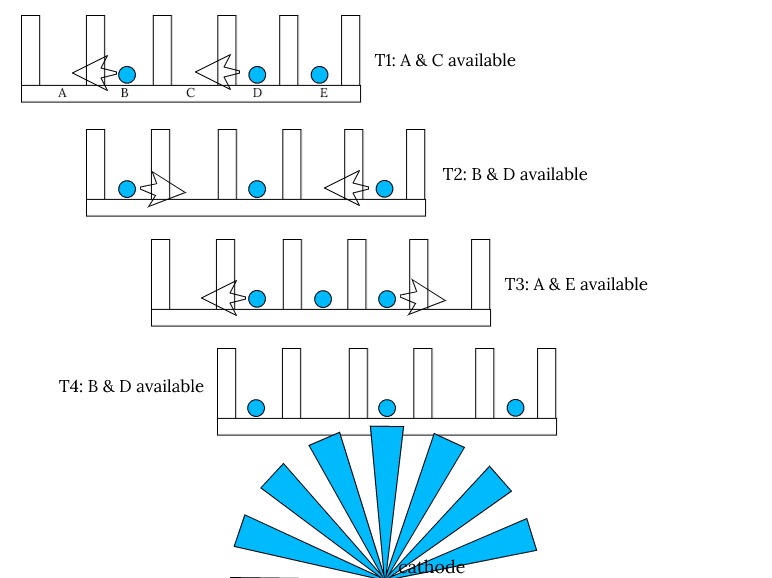
In the first image, taken at time T1, the rightmost electron at site E cannot tunnel into site D because an electron already exists there. (In this case, we're not talking about Pauli exclusion, simply electrostatic repulsion, but feel free to mentally add Pauli exclusion into the picture, which is a much more powerful effect.) Likewise the electron at site D cannot tunnel into site E. But it can tunnel into site C.
It is only once the electron at D tunnels into site C that the electron at E can tunnel into site D (second snapshot at time T2). And so on and so forth. This is analogous to the microstate exploration of the back screen in the double-slit experiment. Let's imagine, for illustrative purposes, that we've built a back screen made entirely of single electron transistors (with fixed gates for now).
Clearly then the true probability of transmission from cathode to any given site A-E is not identical to the absolute square of the wavefunction (denoted by the blue rays coming from the cathode), but also on whether each site has an electron in it or not. If an electron was measured on the screen at T4, we would expect to find the electron in sites B or D most of the time unless the wavefunction at these sites was small. But at T3, we would expect to find the electron at sites A or E.
None of this is captured in P12 (where 2 = (A, B, C, D or E at some time T?)) which is a statement only about the wavefunction coming from the cathode, and yet is physically essential to the probability of finding the electron at a given site at a given time. (NB: if the incoming electron is of sufficiently high energy, and has opposite spin to the occupying electron, it can still get into an occupied site, however it is much less likely to do so.)
We can also see that, over time, all of the sites will be available or not, leading to a statistically useful time-averaged probability per site of the screen receiving an electron from the cathode: that is, we expect statistically that the probability given by the incoming electron to dominate over the noisy data about which sites are and are not available at given times, so we should recover the usual interference patterns.
It's also worth pointing out that a screen in which we can measure the position of an incident particle to some high accuracy is more like an array of single electron transistors than like the ideal metal of naïve Copenhagen interpretation. -
 SophistiCat
2.3kI was just rereading part of the paper on type II emission and absorption events, which are interesting. If we take the emitter to be atom 1, the absorber to be atom 2, and the emission to be a photon, from the lab frame it appears as a photon (its own antiparticle) from the origin is absorbed by atom 1 (the emitter) and then, seemingly unrelatedly, atom 2 emits a photon of the same energy, which continues forever.
SophistiCat
2.3kI was just rereading part of the paper on type II emission and absorption events, which are interesting. If we take the emitter to be atom 1, the absorber to be atom 2, and the emission to be a photon, from the lab frame it appears as a photon (its own antiparticle) from the origin is absorbed by atom 1 (the emitter) and then, seemingly unrelatedly, atom 2 emits a photon of the same energy, which continues forever.
If the distance between the two atoms is L, the time between perceived absorption and emission (actually emission and absorption) will be L/c. So if type II is possible, it ought to be detectable experimentally in principle, though in practice it would be hard if the phenomenon is limited to CMB radiation (assuming that's all that could constitute a photon from the origin).
What's more interesting is the idea that causal relationships between events can be apparently unmediated in principle. — Kenosha Kid
I wonder why he thinks that Type II emission is equally possible as Type I. Type I is an interpretation of a well-studied phenomenon (emission/absorption of EM radiation), while it takes work to explain away Type II. What's the rationale in proposing it? Just a general preference for symmetry? -
 Kenosha Kid
3.2kI wonder why he thinks that Type II emission is equally possible as Type I. Type I is an interpretation of a well-studied phenomenon (emission/absorption of EM radiation), while it takes work to explain away Type II. What's the rationale in proposing it? Just a general preference for symmetry? — SophistiCat
Kenosha Kid
3.2kI wonder why he thinks that Type II emission is equally possible as Type I. Type I is an interpretation of a well-studied phenomenon (emission/absorption of EM radiation), while it takes work to explain away Type II. What's the rationale in proposing it? Just a general preference for symmetry? — SophistiCat
Yes, I think because the general formalism underlying Type I also allows for Type II such that disallowing Type II requires extra by-hand theory. -
 SophistiCat
2.3kI am not sure. The motivation for the Wheeler-Feynman absorber theory is to have a time-symmetric formulation of classical electrodynamics, right? Both Type I and Type II absorptions are symmetric, but I don't see why you have to have both, and not just Type I. Type I is just an interpretation of classical electrodynamics, since it is formally equivalent to it. Type II constitutes a net new addition to the theory, for which we have no evidence.
SophistiCat
2.3kI am not sure. The motivation for the Wheeler-Feynman absorber theory is to have a time-symmetric formulation of classical electrodynamics, right? Both Type I and Type II absorptions are symmetric, but I don't see why you have to have both, and not just Type I. Type I is just an interpretation of classical electrodynamics, since it is formally equivalent to it. Type II constitutes a net new addition to the theory, for which we have no evidence.
Of course, if it could be tested and confirmed, that would be quite remarkable. Cramer says (referring to earlier analyses) that if both types occur, one of them is expected to dominate - presumably, Type I in our case. But that still leaves a possibility of some Type II absorptions. Do you think it's likely that they would have gone unobserved/unnoticed all this time? How difficult would this be to test? -
 Kenosha Kid
3.2kThe motivation for the Wheeler-Feynman absorber theory is to have a time-symmetric formulation of classical electrodynamics, right? Both Type I and Type II absorptions are symmetric, but I don't see why you have to have both, and not just Type I. Type I is just an interpretation of classical electrodynamics, since it is formally equivalent to it. Type II constitutes a net new addition to the theory, for which we have no evidence. — SophistiCat
Kenosha Kid
3.2kThe motivation for the Wheeler-Feynman absorber theory is to have a time-symmetric formulation of classical electrodynamics, right? Both Type I and Type II absorptions are symmetric, but I don't see why you have to have both, and not just Type I. Type I is just an interpretation of classical electrodynamics, since it is formally equivalent to it. Type II constitutes a net new addition to the theory, for which we have no evidence. — SophistiCat
I think it's more that the Wheeler-Feynman (WF) theory automatically includes Type II. It's an addition to classical EM, yes, but you'd have to add something to WF in order to get rid of it. -
 SophistiCat
2.3kGetting back to this topic (sorry, this is tough slog for the old cat-brain):
SophistiCat
2.3kGetting back to this topic (sorry, this is tough slog for the old cat-brain):
Clearly then the true probability of transmission from cathode to any given site A-E is not identical to the absolute square of the wavefunction (denoted by the blue rays coming from the cathode), but also on whether each site has an electron in it or not. — Kenosha Kid
So the bolded part is what I am having difficulty with. We do, of course, observe that the cumulative distribution of impacts on the back screen is in line with the square of the wavefunction from the emitter. If emission occurs whenever, while the distribution of available absorbers on the screen is constrained by external factors, then we won't recover the expected distribution. (In the edge case where at most one site is available at any time, the distribution won't even have any dependence on the impacting wave!)
The only way to recover the expected distribution that depends only on the impacting wavefunction is if the timing of successive emissions is coordinated so as to compensate for the constraint from boundary conditions and produce an undistorted distribution over time. But I don't see how such compensatory mechanism is being realized.
Cramer claims to have mapped the above arrow of time to the cosmological arrow in the paper here:
Another paper by Cramer (Foundations of Physics, 1973) specifically treating the arrow of time: http://faculty.washington.edu/jcramer/TI/The_Arrow_of_EM_Time.pdf — Kenosha Kid
However the more I read it the less compelling I find it. He actually talks about advanced waves going forward in time, which is contrary to what an advanced wave is. — Kenosha Kid
Yes, he says the entire four-vector is reflected at the Big Bang boundary, i.e. time direction of the advanced wave is flipped, but still insists that the reflected wave is an advanced wave. But other than terminology, do you see any issues with his proposal? -
 Darko B
2
Darko B
2
Hi,
Cramer’s proposal cannot work for EM waves, simply because the early universe was not transparent to EM radiation. Advanced electromagnetic waves, traveling backward in time, can reach some 380,000 years after the Big Bang and therefore can’t be reflected at the Big Bang boundary. Instead of that, they should interact with the ordinary dense matter, at least 380,000 years old, as shown in Figure 2 from my recent paper on the problem of the direction of the electromagnetic arrow of time: http://philsci-archive.pitt.edu/13505
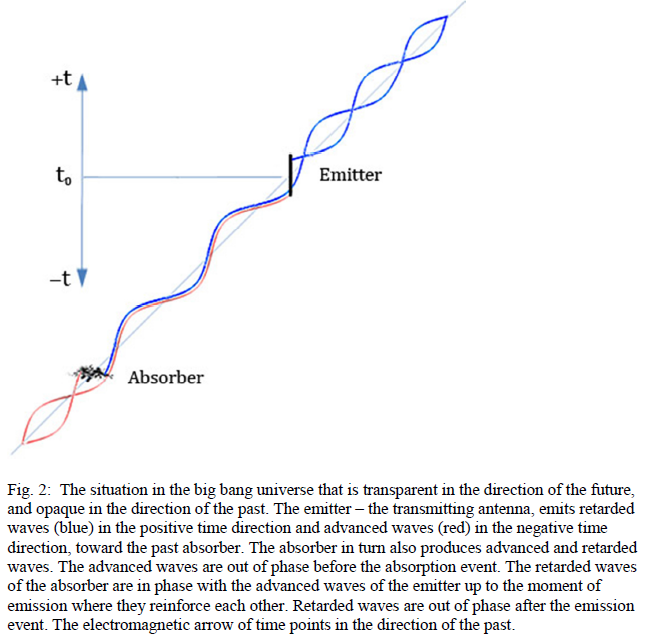
This interaction would seem to us like retarded EM radiation coming about 380,000 years after the Big Bang, although it is actually 1/2 advanced + 1/2 retarded EM radiation. -
 SophistiCat
2.3kCramer’s proposal cannot work for EM waves, simply because the early universe was not transparent to EM radiation — Darko B
SophistiCat
2.3kCramer’s proposal cannot work for EM waves, simply because the early universe was not transparent to EM radiation — Darko B
He addresses this concern in the paper, but this physics is way above my pay grade.
Welcome to The Philosophy Forum!
Get involved in philosophical discussions about knowledge, truth, language, consciousness, science, politics, religion, logic and mathematics, art, history, and lots more. No ads, no clutter, and very little agreement — just fascinating conversations.
Categories
- Guest category
- Phil. Writing Challenge - June 2025
- The Lounge
- General Philosophy
- Metaphysics & Epistemology
- Philosophy of Mind
- Ethics
- Political Philosophy
- Philosophy of Art
- Logic & Philosophy of Mathematics
- Philosophy of Religion
- Philosophy of Science
- Philosophy of Language
- Interesting Stuff
- Politics and Current Affairs
- Humanities and Social Sciences
- Science and Technology
- Non-English Discussion
- German Discussion
- Spanish Discussion
- Learning Centre
- Resources
- Books and Papers
- Reading groups
- Questions
- Guest Speakers
- David Pearce
- Massimo Pigliucci
- Debates
- Debate Proposals
- Debate Discussion
- Feedback
- Article submissions
- About TPF
- Help
More Discussions
- Biological Determinism As The Fundamental Pedagogy of National Socialist Thought (Half of Chapter 1)
- Biological Determinism As The Fundamental Pedagogy of National Socialist Thought
- The Consequences of Belief in Determinism and Non-determinism
- Are we doomed to discuss "free will" and "determinism" forever?
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum





