Who are those many people? — TonesInDeepFreeze

For my part, I hope he's right...

Captures the theme admirably.

Infinity is unknowable by the finite human mind, yet we know the meaning of "infinity"

Anyway, to say that thinking of abstractions requires thinking of a concrete examples does not say that we don't think of abstract objects — TonesInDeepFreeze

The human mind can discover both abstract and concrete concepts in physical object

I agree. The ability of being able to think about abstract concepts, such as independence, beauty, love, anger and infinity, is a crucial part of the human mind.

When travelling to a foreign country, and hearing the word "hasira" for the first time, how in practice can we learn its meaning. If it were a concrete noun, the local could point out several physical examples that could be described by it in the hope that the foreigner was able discover what they had in common. However if it were an abstract noun, would the same approach be possible, as in pointing out several physical examples that could be described by the abstract noun?

If I visited a university with a guide, and the guide pointed at the buildings and people moving between the buildings carrying books, and said "this is a university", would I understand the meaning of "university" just as the concrete concept "a set of buildings" or the abstract concept "a place of teaching"?

I believe that the human mind is inherently capable of discovering when looking at a physical object both concrete and abstract concepts

IE, the human mind knows the meaning of words such as "beauty" and "infinity" and can think about the abstract concepts beauty and infinity, and could have learnt what concept is connected to what word by being shown several physical examples of each.
===============================================================================

What is a concrete example of the concept of 'does not exist'? — TonesInDeepFreeze

The human mind can discover abstract concepts in physical objects



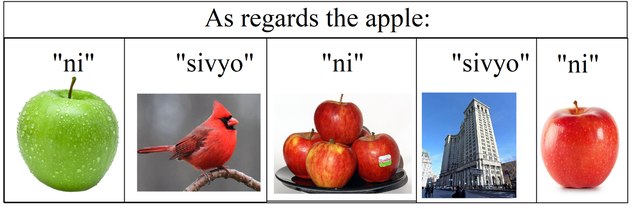
You are a foreigner in a foreign land learning the language. You have already learnt that the word "tufaha" means "apple", and you are now trying to learn the concepts expressed in the words "ni" and "sivyo". To make life more difficult, these are abstract concepts. But what is your best guess as the the meanings of "ni" and "sivyo"? The fact that are able to make an educated guess shows that abstract concepts can be instantiated in concrete examples.
===============================================================================

Also, the lines I'm thinking along is that certain utterly basic abstractions, such as 'object', 'thing', 'entity', 'is', and 'exists' themselves presuppose abstraction no matter what concretes are involved or not. — TonesInDeepFreeze

Abstract concepts don't of necessity refer to physical things, but wouldn't exist without physical things

Yes, the meaning of the abstract concept "exists" is independent of any particular physical thing referred to, whether an apple, a house or a government. However, the abstract concept "exists" would not exist in the absence of physical things.

The concept "Beauty" would not exist if there were no beautiful things. The concept "anger" would not exist if there were no angry people. The concept "governments" would not exist if there were no societies of people. The concept "infinity" would not exist if there were no physical objects.

IE, an abstract concept does not refer to a particular concrete entity, but abstract concepts wouldn't exist if there were no concrete entities.
===============================================================================

Yet, the notion of 'concrete instantiation' is itself an abstraction made of the the two abstractions 'concrete' and 'instantiation'. — TonesInDeepFreeze

New words are learnt either within language or within a metalanguage

On the one hand, words can be learnt by description, where a new word is learnt from known existing words. For example, if I know the words "a part that is added to something to enlarge or prolong it", then I can learn the new word "extension".

However, if only words were learnt by description, there would be the problem of infinite regress. Sooner or later some words must be learnt by acquaintance, where a word such as "beauty" can be learnt by looking at particular "concrete instantiations", such as a Monet painting of water-lilies or a red rose in the garden.

Similarly, I can learn the meaning of the word "concrete" by looking at particular "concrete instantiations", such as a bridge over a river or a skyscraper in a city.

But, as you point out, this suggests an infinite regression, in that it seems that I can only learn the word "concrete" if I already know the meaning of "concrete instantiation".

However, this is not the case, as whilst words by description are learnt within language, words by acquaintance are learnt outside language and within a metalanguage.

IE, the new word "beauty", although it becomes part of language does not require language to be learnt. This avoids the infinite regress problem of learning the meaning of the word "concrete", if in order to learn the meaning of "concrete" I must already have to know the meaning of "concrete instantiation"

Two types are extensionally equal if they reduce to the same set of values when the abbreviations used in their respective definitions are expanded out. Nevertheless they aren't intensionally equal unless their definitions are the same before their expansions. Generally speaking, type theory distinguishes intensional equality, also referred to as definitional equality, from extensional equality, due to the fact that the extensional notion of equality is undecidable unless types are restricted to decidable sets. Whilst intensional equality always implies extensional equality, the converse is only true for "extensional" type theories which are those type-theories that define intensional equality explicitly in terms of the fully-expanded extensional equality. But this implies that type-checking in those theories is generally undecidable and very expensive to compute in comparison to intentional type-theories that don't bother to consider extensional equality when type checking. For this reason, general-purpose theorem proving languages tend to be intensional, meaning that two terms or types are only considered to be equal for the purposes of substituting one for the other in a given context, only after the programmer has both constructed a proof-term that they are equal, and has also granted explicit permission to substitute one for the other in that context on the basis of that proof. So the practical difference between extensional and intensional type theories is the degree of automation that they permit during the process of type checking, i.e the burden of proof that they put onto the programmer.

However, in more advanced mathematical contexts like set theory, "=" is sometimes used to signify identity, indicating that two objects or sets are the same in every aspect. — ChatGPT

Just be a mathematical antirealist and accept that “true” in the context of maths just means something like “follows from the axioms”, with the axioms themselves not being truth-apt. — Michael

Then you'd have to reject the axiom of extensionality, and all axioms which follow from it, and set theory in general. As I explained, allowing that there is an object (abstraction, conception, or whatever you want to call it), which is referred to by a description like "1+1" is "truth" by correspondence. So it would be hypocritical to accept axioms which are demonstrably based in "truth", correspondence, yet claim that they are not truth-apt.

You’re making a mountain out of nothing. — Michael

I'm not trying to make a mountain, just arguing a point, and points are "nothing". You are making points into a mountain by implicitly accepting Platonic realism.

It tells us how to use the "=" sign. It is an instruction, and so is not the sort of thing that can be false. You either follow the instruction or you do not. If you do not follow the instruction you are not participating in the logic of sets. — Banno

The problem occurs when that axiom is interpreted as indicated that when A=B, then A is "identical" to B, in the sense that "A" and "B" each signify the same thing, as @TonesInDeepFreezeargues. This would mean that there is a "thing", with an identity, which is represented by both "A" and "B", such as in the examples provided by @Michael and @TonesInDeepFreeze. However, since there is no necessity of order within a set, and also there is such a thing as an empty set, it is very evident that it would violate the law of identity to interpret the axiom of extensionality as indicating "identity".

Incidentally, I argued extensively with @fishfry, that to read the axiom of extensionality as indicating identity rather than as indicating equality is a misinterpretation. However, it seems like identity is the conventional interpretation, and there are further aspects of set theory which require that equal sets are the same set And that produces a problem.

[

It tells us how to use the "=" sign. It is an instruction, and so is not the sort of thing that can be false. You either follow the instruction or you do not. If you do not follow the instruction you are not participating in the logic of sets.

The law of identity has various forms, but in set theory it is that
A=B iff both A⊆B and B⊆A.
— Open Logic
This is a consequence of extensionality, not an axiom. — Banno

My argument is a very simple one, and I am not trying to build it into a mountain. The point is that the sense of "identity" employed in set theory is not consistent with, therefore violates, a proper formulation of "the law of identity" expressed as an ontological principle. That itself is not a big deal, many philosophers like Hegel for example, have argued that there is no good reason for logicians to have respect for that ontological law. Leibniz, on the other hand, for example, argued that this law, along with the related principle of sufficient reason, ought to be respected. You may portray this as "the law of identity has various forms", but if the forms are inconsistent with each other, that implies inconsistency in what we believe constitutes "identity".

The thing which irks me as a metaphysician, (and why I argue this point fervently), is when philosophers of mathematics insist that the sense of "identity" employed in set theory is consistent with the "law of identity", as stated in ontology. These philosophers will employ examples like Tones and Michael did, of the "identity" of a physical object, implying that the "identity" of an abstraction is analogous, through some misinterpretation of "extensionality".

The reason it bothers me is that the law of identity is the principal tool employed by Aristotle against the sophistry of Pythagorean/Platonic realism. If we allow corruption of that "law", and ignore the difference between "identity" as employed by set theorists, and "identity" as stated in the law, we give up the front line in that defence, effectively surrendering to Eleatic sophistry (ref. Plato, The Sophist)

What Meta is doing is refusing to use "=" in the way the rest of us do. — Banno

If any one of you would look at the evidence of what I've presented, the use of the equation in mathematics, they would see that "the rest of us" use "=" in the way that I describe. The common way, that of the applied arithmetic of the common people, and the applied mathematics of architects, engineers, and scientists, is the way I describe. It is only a select few, those immersed in the advanced mathematics of set theory, who desire, for the sake of this theory, that mathematical objects have an "identity", who choose to make "=" signify something different.

You ignore what ChatGPT told you in the other thread, common arithmetic and mathematics use "=" as equality not identity. And, in this thread GP said, that sometimes in "advanced mathematical contexts like set theory" ... "from the need to express relationships between objects", "=" will signify identity. Why do you refuse to accept what GP told you? That's because Tones told you 'don't to listen to that machine it doesn't support me', or something like that. But what is GP's account really based on? The "way the rest of us" use "=". Clearly, it's Tones who is "refusing to use "=" in the way the rest of us do", not Meta.

But that internal sensations cannot be treated in the way we treat other objects. — Banno

That's exactly the point. Objects each have an unique "identity", like Wittgenstein shows with the chair example. Even if two chairs might appear to be the same so that we couldn't readily decide which is which, we'd still know that through some temporal continuity each maintains its own unique identity. This ontological belief is expressed by the law of identity. Whether that law is actually true or not is not the point, it's just an ontological belief, and by believing it we assume that it's true. Internal sensations cannot be treated as if they have such an "identity". Therefore we make your conclusion, "internal sensations cannot be treated in the way we treat other objects". You seem to readily accept the conclusion which Wittgenstein comes to, without understanding the argument that he presents which produces it.

I see no philosophy nor mathematics in your latest replies to me. It appears you've simply gone off the rails in your crackpot ways. Oh well, maybe next time you'll be able to stay on track and manage a reasonable discussion.

Why do you hope that many people want your posts to be monitored by the moderators?

I see no philosophy nor mathematics in your latest replies to me. — Metaphysician Undercover

Make an appointment with an ophthalmologist.

In this part of the discussion, as in some recent posts, that is not directly about what mathematics itself in fact says, I am not trying to convince anyone else to see it the way I do. Rather, these are explanations of my best attempts to, for myself, have a framework to understand abstraction, truth and related questions. As a very broad generalization, I think of at least these two categories: (1) Matters of fact. (2) Matters of frameworks for facts. With (1), truth and falsity apply. With (2), coherence and explanatory robustness applies.

In this thread, for example: That ordinary mathematics says "1+1 is 2" is matter of fact. But whether ordinary mathematics should say that 1+1 is 2 is a matter of framework. And I take ordinary mathematics not as an account of facts about concretes, but rather as a framework for such facts. And, again, we need to distinguish between what ordinary mathematics does in fact say with what one may think it should say and what one thinks should be the framework for mathematics.

Frameworks involving abstractions sometimes work in an "as if" way. There is a wide range of thinking about what mathematical objects are - platonic, fictional, in some special sense concrete, or even extreme nominalism. But whatever we take mathematics to be talking about, at least we may speak of abstractions "as if" they are things or objects. Not concrete objects, but "as if" they are handled grammatically similar to they way concretes are handled.

Common examples, to the point of cliche, abound, such as that the knight in chess is not a concrete knight, not any particular piece of wood or plastic resting on a particular piece of wood or cardboard, but rather an abstract concept that we speak of similarly to the way we speak of concretes. This similarity does not imply that the abstract chess object that is the knight is a certain piece of wood. And so we use such locutions as "It either moves up or down one square vertically and over two squares horizontally, or up or down two squares vertically and over one square horizontally" The 'it' there must refer to something, and it sure as shootin' don't gotta a physical object. Also, 'moves' and 'square'. Moves can even be made by telephone and the piece that one player moves on his board at home is a different piece from the one the other player moves on his board at home, but it is understood (courtesy of ABSTRACTION) that those pieces represent the SAME knight. One can even perform a game of chess purely mentally, as chess masters actually do. And just as one can perform arithmetic and even more complicated mathematics purely mentally. That is courtesy of ABSTRACTION.

And the number 1 in mathematics is an abstract mathematical object that we speak of in a similar way to the way we speak of concretes, but that does not imply that the number 1 is a concrete object.

Going back to even more fundamental considerations, my starting premise is that experience is occurring. And as 'experience' and 'occurring' are the notions I start with, I must take them as primitive.

Notice that I didn't say 'experiences' plural, because I had not yet gotten to saying that not only does experience occur but that experience has parts and thus there are a multiplicity of experiences. But I do go on to say that if I do not allow there are many experiences, it would be intractable for me to talk about the experience that occurs.

Notice that I didn't say "I am having experiences", since I had not yet gotten to a premise that there is a thing that is named 'I'. But then I do refer to 'I' and as a thing, as it would be intractable for me to go beyond 'experiences are occurring' without being able to couch my experiences with reference to 'I', thus I as a thing.

As I go on, I find that certain other notions such as 'is', 'exists', 'thing' or 'object', 'same' 'multiple'. etc. are such that I don't see a way to define them strictly from the primitives I've allowed myself. So I then take such notions as themselves "built in" to whatever thinking I'm going to be doing.

So, by adding more concepts, I eventually - pretty crudely, without all the steps filled in, since I don't claim to have provided even for myself a rigorous philosophical system - get to the idea that there are other people having experiences, and that enough of these experiences have commonality among people such that we can submit claims about them to a process of judging claims as publicly factual or not, and with finer and finer standards of judgement such as those of the sciences. But the very determinations of fact, let alone the conceptual organization of facts, are vis-a-vis frameworks, and it is not disallowed that one may use different frameworks for different purposes.

For me, the value and wisdom of philosophy is not in the determination of facts, but rather in providing rich, thoughtful, and creative conceptual frameworks for making sense of the relations among facts. And, again, different frameworks may be used for different purposes.

Meanwhile, I would not contest that formation of concepts relies on first approaching an understanding of words ostensively.

As I say, I do not propose this as prescriptive, but rather only that it describes my own humble attempt to make sense of stuff for myself. Hopefully it might be a heuristically useful for others, but I don't insist that it must be.

The axiom of extensionality is not inconsistent with identity theory.

Here is the most trivial model of both the axiom of extensionality and identity theory ('e' for the epsilon symbol):

The domain of discourse is {0 {0}}

'=' for the identity relation on the domain of discourse, i.e., {< 0 0> <{0} {0}>}

'e' for the membership relation on the domain of discourse, i.e., {<0 {0}>}

In that that model, all the axioms of identity theory and the axiom of extensionality are true.

If folk wish my posts moderated, then they have been paying attention to them.

Along the lines of "Bad publicity is at least publicity".

Infinity is unknowable by the finite human mind, yet we know the meaning of "infinity" — RussellA

In mathematics, there are 'points of infinity' (which are not necessarily infinite sets), and the expression 'to infinity' (which is a figure of speech that when we unpack it we don't have an 'infinity' that is referred to), etc. But ordinarily there is no object that we name with the noun 'infinity', rather there is the adjective 'is infinite'. So, at least in a mathematical context, "Infinity is unknowable" doesn't have an apparent meaning to me. On the other hand the meaning of 'is infinite' is quite clear, as it means 'not finite'.

Abstract concepts don't of necessity refer to physical things, but wouldn't exist without physical things — RussellA

That requires a framework that defines 'physical thing' or takes it as primitive. Then, depending on whatever framework is proposed, we could examine whether there cannot be abstractions independent of physical things.

It does seem to me that concepts are formed from prior ostensive inferences. But that is epistemological, not necessarily ontological.

I'm just glad that when I think of mathematics, there is the abstract mathematical object 1 and that there are not as many of the number 1 as there are each of certain physical events in brains starting and stopping.

Pretty much. One can't do philosophy well without being critical, which entails sometimes pissing people off.

The point is that the sense of "identity" employed in set theory is not consistent with, therefore violates, a proper formulation of "the law of identity" expressed as an ontological principle. — Metaphysician Undercover

Here is the axiom of extensionality:

If A and B are sets, then A = B iff every element of A is also an element of B, and vice versa.

Here is the law of identity

A=A

Set out for us exactly how these are not consistent.

The cranks says, "Tones told you 'don't to listen to that machine it doesn't support me', or something like that."

Again, the crank LIES about me.

I never said that Chat GPT is not to be trusted because it doesn't agree with me.

Rather, it is not to be trusted because, over and over and over, it has been demonstrated, by many people, to spout blatant falsehoods. Indeed, even the makers of AI themeleves stress that AI is not necessarily a source of information but rather its primary role is as a composition tool.

Anyone can see for themselves that Chat AI outright fabricates, easily by asking it questions that it does not have ready answers to.

Moreover, in my conversation with Chat AI, it did say "1+1 is 2". But the crank SKIPS that.

To advance his illogical and confused argument, the crank resorts to LYING about what I said. He is pathetic.

Which is to say, he cannot do the impossible.

Still picking up after the crank's daily littering:

The axiom of extensionality is not used to prove axioms. Rather, the axiom of extensionality is used to prove theorems.

/

The denotion of '1+1' is not truth by correspondence. '1+1' is not a statement, thus it doesn't have a truth value.

/

There are non-platonistic senses in which we may speak of mathematical objects.

/

Identity theory is congruent with Leibnitz's principles of the identity of indiscernibles and the indiscernabilily of identicals.

/

Chat GPT is not even remotely a reliable reference. Even a lowly fortune cookie can tell you: "He who cites Chat GPT as authority makes of himself a fool". Anyway, just to see, I asked Chat GPT, and it said that 1+1 is 2, and it did not confine that to set theory. And, of course, the cranks SKIPS that. Anyway, that a supposed philosopher is citing the famously "hallucinating" Chat GPT as an authority indexes how pathetic the Internet has become, not merely how pathetic he has always been.

Yep.

So the reply will consist in an obfuscation of the law of identity by confusing it with an "ontological" principle. Mistaking a language act for a thing in the world.

Another episode from 'The Adventures of the Crank Metafizzled Blunderbound in the Land of Daily Life':

Support Rep: Hello, thank you for calling MetaCard, your card to be used anywhere for making purchases of things about things. How can I help you today?

Crank: It says on my statement that I have 10000 rewards points. Please send them to my address: 666 South Georg Wilhelm Friedrich Hegel Alley, Apartment 0, Unreality Village, Planet Mars. No zip code on that.

Support Rep: I'm sorry, but they are rewards points. We don't send them to you. You use them for discounts on things.

Crank: How can I use them if I don't have them here? If they're real, then you can send them to me.

Support Rep: They are real. But they're not like physical goods that we ship to you.

Crank: Please don't tell me that you too have been infected by set theory! I'm asking you again to send me my points!

Support Rep: I'd like to help. Let me explain it this way: The rewards points are a number. It is noted on your account. Your points may change, but when we say "Metafizzled Blunderbound's points on February 18, 2024" we mean whatever number of points is on your account at that date. So it's a number, not a physical thing. However, depending on what that number is, you may apply that number for discounts on purchases you make. For each point you get ten cents off your puchase. There's nowhere for me to go get your number of points and ship them to you.

Crank: Look, what is that number now?

Support Rep: Metafizzled Blunderbound's number of points is 10000.

Crank. No, no, no! Not is. Equals! The number of my points equals 10000. Not the number of points is 10000. It is nonsense to say that the number of my points is 10000!

Support Rep: I can say 'equals' if you like. But I'm just telling you what your number of points is.

Crank: You are talking nonsense, and these are not even real things, so don't even bother!

Support Rep: So you don't want to apply your points to any purchases?

Crank: How can I apply them if they're not even real?! I surely am not going to be fooled into trying to use something that is not real! And I definitely am not going along with your bad philsophy where you say is instead of equals! Good day to you! (Hangs up.)

Two weeks later:

Bob (Blunderbound's neighbor): Hey, Blunderbound, how's it hangin'?

Crank: Okay, Bob. You should see how I'm tearing apart those set theory guys at The Philosophy Forum. Ha, I even showed them that Chat GPT says I'm right!

Bob: That's great. Hey, check out this waterproof watch I got. Got a discount with my MetaCard points. Have you used any of your points?

Crank: No, I don't use things that are not real. Besides, I don't need a watch. I have my sundial in the backyard. (Music cue, trombone "wamp wamp wamp")

For me, as a kid, New Math was wonderful — TonesInDeepFreeze

That's great. Some kids really liked it, even though their parents didn't. I only tried teaching it in a typical college algebra course using a book by Vance. The first chapter was elementary set theory. My students had the ordinary curriculum in elementary through high school and for the most part were aghast at having to reason that a0=0.

Don't know that book, but

Ax x*0 = 0 is an axiom of first order PA, so it's easy to prove x*0 = 0

and in set theory, the PA axioms are theorems.

/

It was wonderful me in the 5th grade to learn about reasoning in mathematics and seeing things like different base numbering systems and modular arithmetic rather than just memorizing multiplication tables, executing steps in long division and reducing fractions to lowest terms.

Don't know that book, but

Ax x*0 = 0 is an axiom of first order PA, so it's easy to prove x*0 = 0 — TonesInDeepFreeze

Maybe it was aI=I. I don't recall. (In the recovery annex of the hospital recuperating from a broken leg at age 87) :sad:

I really hope you get well soon.

Here is the axiom of extensionality:
If A and B are sets, then A = B iff every element of A is also an element of B, and vice versa.

Here is the law of identity
A=A

Set out for us exactly how these are not consistent. — Banno

To begin with, the obvious. "Every element of A is also an element of B" is insufficient for identity by the law of identity because "A=A" implies that not only the elements, but also the order to the elements of A and B would need to be the same. Furthermore, every aspect of what is named A, and what is named B, must be precisely the same, even the unknown aspects.

Quite simply, stating some feature such as "every element is the same", is insufficient to qualify as identity by the law of identity, because the law of identity, as "a thing is the same as itself", or "A=A", implies that every aspect of the thing must be the same to qualify as "identity.

"A=A" implies that not only the elements, but also the order to the elements of A and B would need to be the same. — Metaphysician Undercover

What?

Why would A=A imply that the order of the elements in B would need to be the same as A?

I think you've lost the plot entirely.

The crank is ignorant and confused about identity theory and the axiom of extensionality, so this at least is reference for how they actually work in set theory:


An axiomatization of identity theory:

Axiom:

Ax x=x

Axiom Schema:

If P is an atomic formula
and
Q is the same as P except y occurs in zero or more places in Q where x occurs in P

then all closures of the following are axioms:

(x=y & P) -> Q

/

Set theory has the primitive 2-place relation symbol 'e'.

We can formulate set theory with the 2-place relation symbol '=' taken as prmitive, or we can formulate set theory with the 2-place relation symbol as defined. The formulations are equivalant.


Primitive:

Adopt the axioms of identity theory and add the axiom of extensionality. But in the language of set theory, the only atomic formulas are of the form xez for any variables x and z (so also zex, yez and zey).

So the axioms of identity theory for the language of set theory include:

Ax x=x

Axyz((x=y & zex) -> zey))

Axyz((x=y & zey) -> zex))

Axyz((x=y & xez) -> yez))

Axyz((x=y & yez) -> xez))

Axiom of extensionality:

Axy(Az(zex <-> zey) -> x=y)

So we have the theorem:

Axy(x=y <-> (Az((zex <-> zey) & (xez <-> yez))


Defined:

Axy(x=y <-> (Az((zex <-> zey) & (xez <-> yez))


So the two formulations are equivalent.


Notice that we have these theorems:


Axy(x=y -> (Az((zex <-> zey) & (xez <-> yez)), which carries, for the language of set theory, Leibniz's indiscernability of identicals.

and

Axy((Az((zex <-> zey) & (xez <-> yez) -> x=y), which carries, for the language of set hteory, Leibniz's identity of indiscernabiles.

/

Moreover, the consistency of the axiom of extensionality with idenity theory is trivally proven by the trivial model I mentioned:

The domain of discourse is {0 {0}}

'=' for the identity relation on the domain of discourse, i.e., {< 0 0> <{0} {0}>}

'e' for the membership relation on the domain of discourse, i.e., {<0 {0}>}

In that that model, all the axioms of identity theory and the axiom of extensionality are true.

The crank can't properly respond to that, because he doesn't know anything about models and consistency.

Order has nothing to do with this.

An ordering is a certain kind of relation on a set.

The axiom of extensionality pertains no matter what orderings are on a set.

{0 1 } = {1 0}

{<0 1>} is an ordering on {0 1}
and
{<1 0>} is an ordering on {0 1}

{<0 1>} not= {<1 0>}

{<0 0> <1 1>} is a sequence whose range is {0 1}
and
{<0 1> <1 0>} is a sequence whose range is {0 1}

{<0 0> <1 1>} not= {<0 1> <1 0>}

Orders and sequences are rigorous in set theory. And the axiom of extensionality is not inconsistent with them.

The crank is ignorant and confused about identity theory and the axiom of extensionality, so this at least is reference for how they actually work in set theory: — TonesInDeepFreeze

See your ad hominem attacks on other interlocutors from the beginning of your posts? That is not a good manner at all. Please just discuss the philosophy. Have some respect. Don't throw insults to the other interlocutors.

This is exactly how you have started in this thread on many of your previous posts. If you track back your posts, you will see them clearly unless you have edited them out. My statements on the point is proven to be true here.

That is false, since you didn't say that you lied but you did lie.

The plain record of the posts in this thread prove that you lied, as I explicitly linked to the posts. But you skip that. — TonesInDeepFreeze

You don't present any logic in your claims and statements. You just imagine that I didn't say something, and that is the only ground for your false claim. Where is your logic and evidence for your claim?

I have not made many claims quoting hundreds of philosophers. That is just another distortion of the truth with exaggeration.
— Corvus

The quote above, written to Banno, is exaggeration thus distortion.

Banno didn't say that you have made claims by quoting hundreds of philosophers.. — TonesInDeepFreeze

Still speaking on behalf of Banno? Look I am not interested in your ad hominem posts begging for attention.  I am here to read and discuss philosophy.