-
 fdrake
7.2kI hope I'm not the only one who recognizes that you are more interested in this conversation than me. :grin: — Leontiskos
fdrake
7.2kI hope I'm not the only one who recognizes that you are more interested in this conversation than me. :grin: — Leontiskos
Aye.
A circle is a plane figure bounded by one curved line, and such that all straight lines drawn from a certain point within it to the bounding line, are equal. The bounding line is called its circumference and the point, its centre. — Circle | Wikipedia
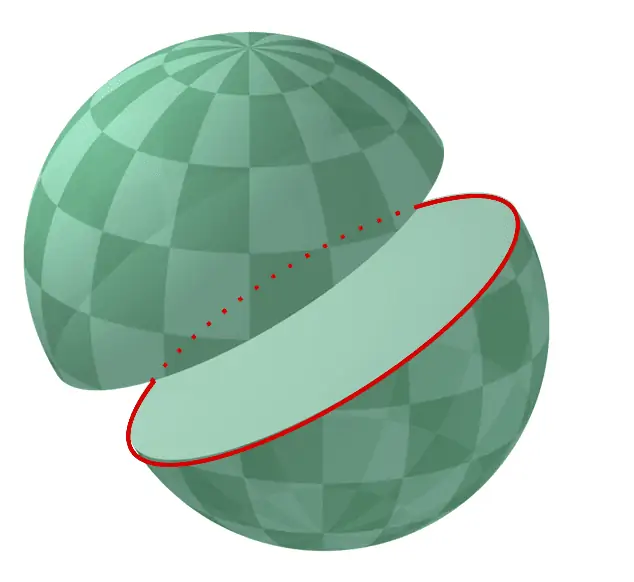
Euclid says: not a circle. The great circle is not a plane figure. -
 Leontiskos
5.3kEuclid says: not a circle. The great circle is not a plane figure. — fdrake
Leontiskos
5.3kEuclid says: not a circle. The great circle is not a plane figure. — fdrake
Why do you think this? And what is "the great circle"? -
 fdrake
7.2kWhy do you think this? — Leontiskos
fdrake
7.2kWhy do you think this? — Leontiskos
Read the definition:
A circle is a plane figure bounded by one curved line, and such that all straight lines drawn from a certain point within it to the bounding line, are equal. The bounding line is called its circumference and the point, its centre. — Circle | Wikipedia
A circle is a plane figure... so something which is not a plane figure cannot be a circle.
And what is "the great circle"? — Leontiskos
The great circle is the circle I've highlighted on the surface of the sphere. Since the circle is confined to the surface of the sphere, and the surface of the sphere is not a plane, it is not a plane figure. -
 Leontiskos
5.3kThe great circle is the circle I've highlighted on the surface of the sphere. Since the circle is confined to the surface of the sphere, it is not a plane figure. — fdrake
Leontiskos
5.3kThe great circle is the circle I've highlighted on the surface of the sphere. Since the circle is confined to the surface of the sphere, it is not a plane figure. — fdrake
The cross-section of a sphere is a circle. A circle is always "confined" by its circumference, but it does not follow that it is not a plane figure. -
 Banno
29k
Banno
29k
@Leontiskos as student Delta:But why accept the counterexample? We proved our conjecture— now it is a theorem. I admit that it clashes with this so-called ‘counterexample’. One of them has to give way. But why should the theorem give way, when it has been proved? It is the ‘criticism’ that should retreat. It is fake criticism. This pair of nested cubes is not a polyhedron at all. It is a monster, a pathological case, not a counterexample. — Lakatos, as quoted in Russell -
 fdrake
7.2k
fdrake
7.2k
Exactly.
The cross-section of a sphere is a circle. — Leontiskos
Well who said anything about cross sections? I was talking about the sphere's surface. You chided me before about extraneous points and operations, and now you've given yourself the liberty of splitting a shape in two, taking an infinitely small cut of it, how exuberant. I just gave you a sphere's surface, not a cross section so...
You'll now need to tell me in what circumstances can you take a cross section of a volume and have it work to produce a circle. Let's assume that you can take any volume and any cross section and that will produce a circle...

Therefore those squares and rectangles are circles. Which is absurd. So your principle must have caveats. What are they, you've got some explaining to do! -
 Leontiskos
5.3kWell who said anything about cross sections? — fdrake
Leontiskos
5.3kWell who said anything about cross sections? — fdrake
You depicted one. I even asked what you were depicting and you weren't very forthcoming.
You'll now need to tell me in what circumstances can you take a cross section of a volume and have it work to produce a circle. Let's assume that you can take any volume and any cross section and that will produce a circle... — fdrake
Just read what I already wrote:
The cross-section of a sphere is a circle. — Leontiskos
Again, you seem to be resorting to sophistry, and I don't see this as a coincidence in the least. In order to try to draw an absurd conclusion you are helping yourself to false premises, such as assuming that planes are bounded, or points can be deleted, or that rectangular prisms are spheres. Why are you doing this sort of thing? -
 fdrake
7.2kWhy are you doing this sort of thing? — Leontiskos
fdrake
7.2kWhy are you doing this sort of thing? — Leontiskos
You do this sort of thing because stipulating a definition and then shit-testing it is standard mathematical practice.
I showed you the great circle on the surface of a sphere because I expected you would see it as a circle - it is - but it does not satisfy Euclid's definition of one verbatim, which you were clearly inspired by. And with maths words, verbatim is all anyone has. That's how you test the boundaries of your definitions and the consequences of ideas.
In picking out the great circle as a circle, you in fact sided with the example over the definition you stipulated. Which is the right thing to do, I think. You could also have ardently insisted that indeed, the great circle was not a great circle because it was not a plane figure. But you did not.
So now that you've abandoned Euclid's verbatim definition of a circle, you've got work to do in telling us what you mean by one.
As for me, I mean a set of points equidistant from a point. And by the by that also makes the great circle a circle. Score one for the thing which includes the taxicab circle over Euclid! -
 Leontiskos
5.3kbut it does not satisfy Euclid's definition of one verbatim — fdrake
Leontiskos
5.3kbut it does not satisfy Euclid's definition of one verbatim — fdrake
I think it does. You've only asserted otherwise, you haven't shown it.
You could also have ardently insisted that indeed, the great circle was not a great circle because it was not a plane figure. — fdrake
It is a plane figure. What do you think a plane figure is? Did you delete the interior of the circle from the plane in the same way you deleted the point from the center of the circle? Deleting points or sections of planes is not possible.
To be clear, the cross-section of a sphere fulfills Euclid's definition of a circle. We could also define a circle as the cross-section of a sphere, but I was only saying that every (planar) cross-section of a sphere will in fact fulfill the definition I already set out. -
 fdrake
7.2kI think it does. You've only asserted otherwise, you haven't shown it. — Leontiskos
fdrake
7.2kI think it does. You've only asserted otherwise, you haven't shown it. — Leontiskos
Well I can tell you what I think a plane figure is.*(the definition below looks to me to be a necessary but not sufficient condition for a plane figure)
A plane figure is closed curve which is inside a subset of . By that definition the great circle is not a plane figure, as it's not inside a subset of - that circle instead would be a closed curve inside a subset of , or with extra precision the surface of the sphere.*(let's not talk about the surface of a sphere being something noneuclidean here)
What do you think a plane figure is? -
 Srap Tasmaner
5.2kbut it does not satisfy Euclid's definition of one verbatim — fdrake
Srap Tasmaner
5.2kbut it does not satisfy Euclid's definition of one verbatim — fdrake
I think it does. You've only asserted otherwise, you haven't shown it. — Leontiskos
He doesn't need to. The sphere is a 2-manifold, and his great circle is a set of points on that manifold. There are no planes here, nothing else, only the points on the surface of the ball.
You are imagining the sphere embedded in the usual 3d Euclidean space. Now, imagine it isn't. There is no point the points on this great circle are equidistant from.
As for me, I mean a set of points equidistant from a point. — fdrake
But don't you need to specify coplanar? If we're in 3d space, you've defined a sphere, in 4th I guess some sort of hypersphere, I don't know, blah blah blah. -
 Leontiskos
5.3kWhat do you think a plane figure is? — fdrake
Leontiskos
5.3kWhat do you think a plane figure is? — fdrake
We took our definition from Euclid, and the term there means a figure that lies entirely on a flat plane.
that circle instead would be a closed curve inside a subset of R3 — fdrake
Do you think the "great circle" (which you have yet to define) lies in three dimensional space rather than two dimensional space? That ambiguity is why I asked you to be more clear about what you were depicting in the first place.
Cutting to the case a bit, it seems that you want to talk about "circles" instead of circles and "plane figures" instead of plane figures, etc. Now if we define "distance" in an idiosyncratic way, then of course there are taxicab circles. If we define "distance" in the commonly accepted way, then there aren't. Are we disagreeing on something more profound than that? -
 Leontiskos
5.3kHe doesn't need to. The sphere is a 2-manifold, and his great circle is a set of points on that manifold. There are no planes here, nothing else, only the points on the surface of the ball.
Leontiskos
5.3kHe doesn't need to. The sphere is a 2-manifold, and his great circle is a set of points on that manifold. There are no planes here, nothing else, only the points on the surface of the ball.
You are imagining the sphere embedded in the usual 3d Euclidean space. Now, imagine it isn't. There is no point the points on this great circle are equidistant from. — Srap Tasmaner
Planes and points cannot be stipulated to exist or not exist. Your word "imagine" is on point given my earlier claim that "This idea of 'deleting' points mixes up reality with imagination." The points in question are coplanar, and therefore the figure they enfold is a plane figure. -
 Srap Tasmaner
5.2kPlanes and points cannot be stipulated to exist or not exist. — Leontiskos
Srap Tasmaner
5.2kPlanes and points cannot be stipulated to exist or not exist. — Leontiskos
I did no such stipulating. Look again.
Your word "imagine" is on point given my earlier claim — Leontiskos
And you are ignoring the fact that I used it twice. -
 Banno
29kI'm enjoying this discussion. I'd like to pause in order to draw attention to the similarities between the insistence here on euclidean space and Leon's previous insistence on Aristotelian logic, or Count Timothy von Icarus' insistence on "material logic'.
Banno
29kI'm enjoying this discussion. I'd like to pause in order to draw attention to the similarities between the insistence here on euclidean space and Leon's previous insistence on Aristotelian logic, or Count Timothy von Icarus' insistence on "material logic'.
There's a pattern... -
 fdrake
7.2kBut don't you need to specify coplanar? If we're in 3d space, you've defined a sphere, in 4th I guess some sort of hypersphere, I don't know, blah blah blah. — Srap Tasmaner
fdrake
7.2kBut don't you need to specify coplanar? If we're in 3d space, you've defined a sphere, in 4th I guess some sort of hypersphere, I don't know, blah blah blah. — Srap Tasmaner
Yeah you're right. Circle, n-sphere, all the same thing in my head. Coplanarity works. A set of coplanar points equidistant from a point in their plane of coplanarity. Thanks!*(could repeat previous definition regarding smoothness and point deletion here)
We took our definition from Euclid, and the term there means a figure that lies entirely on a flat plane. — Leontiskos
Do you think the "great circle" (which you have yet to define) lies in three dimensional space rather than two dimensional space? That ambiguity is why I asked you to be more clear about what you were depicting in the first place. — Leontiskos
Fair enough. There's two things though:
Either you consider the sphere as embedded in 3-space, and the cross section plane isn't "flat" in some sense - it's at an incline. Or you consider the surface as a 2-dimensional object, in which case there's not even a plane to think about. Pick your poison. The latter is the original counter example and is much stronger, the former is easier to remedy.
If we define "distance" in the commonly accepted way, then there aren't. Are we disagreeing on something more profound than that? — Leontiskos
You're behaving like you know what these things are so well you've got them baked into your cerebellum. But clearly that's not true, as the definition you provided doesn't match something you clearly recognised as a circle! So yes, we could insist on your pretheoretical intuition, but it's no longer Euclid's... so I'm wondering what's wrong with it? How will you parry my counterexample?
I'm enjoying this discussion. — Banno
It is a lot like something from Proofs and Refutations. -
 Leontiskos
5.3kAnd you are ignoring the fact that I used it twice. — Srap Tasmaner
Leontiskos
5.3kAnd you are ignoring the fact that I used it twice. — Srap Tasmaner
So? The cross-section of a hollow sphere will be a circle regardless of whether I imagine a point at the center or not. -
 Banno
29kIf it makes you uncomfortable, stop tromping on my bridge.
Banno
29kIf it makes you uncomfortable, stop tromping on my bridge.
I had thought the example, Euler’s formula, a bit obtuse. But perhaps Lakatos chose it so as to minimise the number of auxiliary hypotheses that his students could produce.It is a lot like something from Proofs and Refutations. — fdrake -
 Leontiskos
5.3kEither you consider the sphere as embedded in 3-space, and the cross section plane isn't "flat" in some sense - it's at an incline. Or you consider the surface as a 2-dimensional object, in which case there's not even a plane to think about. Pick your poison. The latter is the original counter example and is much stronger, the former is easier to remedy. — fdrake
Leontiskos
5.3kEither you consider the sphere as embedded in 3-space, and the cross section plane isn't "flat" in some sense - it's at an incline. Or you consider the surface as a 2-dimensional object, in which case there's not even a plane to think about. Pick your poison. The latter is the original counter example and is much stronger, the former is easier to remedy. — fdrake
I still think you're just plain wrong. Namely, a 2-dimensional object lies on a plane. Pretending that there is no plane is a curious move. How do we query whether a plane is present or not? A plane is an abstract object, much like a circle. It makes no more sense to say that the cross-section of a sphere does not lie on a plane than it does to say that one can delete the point in the middle of a circle, at which point it magically becomes a non-circle.
But clearly that's not true, as the definition you provided doesn't match something you clearly recognised as a circle! — fdrake
But it does match it, as I've already noted. Your mere assertions are getting old.
How will you parry my counterexample? — fdrake
I'm waiting for you to present one.
If it makes you uncomfortable, stop tromping on my bridge. — Banno
You artificially inserted an extraneous conversation into your own thread and then invited me here, remember? -
 Leontiskos
5.3kBut it does match it, as I've already noted. Your mere assertions are getting old. — Leontiskos
Leontiskos
5.3kBut it does match it, as I've already noted. Your mere assertions are getting old. — Leontiskos
@fdrake if you like: a circle is the two-dimensional subset of a sphere. A sphere is the set of points equidistant from a point in 3-space and a flat cross-section of a sphere is necessarily a circle, namely a set of points equidistant from a point in 2-space. As I've already said, a cross-section of a sphere conforms to the definition of a circle that I originally gave. -
 Srap Tasmaner
5.2kA set of coplanar points equidistant from a point in the plane of coplanarity. — fdrake
Srap Tasmaner
5.2kA set of coplanar points equidistant from a point in the plane of coplanarity. — fdrake
Does that point need also to be coplanar? Is there a counterexample I'm missing?
The cross-section of a hollow sphere will be a circle regardless of whether I imagine a point at the center or not. — Leontiskos
You realize that on the sphere it's just a straight line, I hope.
―― I don't know why I'm participating in this. -
 Leontiskos
5.3kI don't know why I'm participating in this. — Srap Tasmaner
Leontiskos
5.3kI don't know why I'm participating in this. — Srap Tasmaner
Me neither. Banno's baiting into this thread is itself something I wished to avoid long before he resurrected this thread. If you had created a real thread on logical pragmatism we wouldn't be here. Blame's on you. :razz: -
 fdrake
7.2k―― I don't know why I'm participating in this. — Srap Tasmaner
fdrake
7.2k―― I don't know why I'm participating in this. — Srap Tasmaner
I'm gonna bugger off now too.
Does that point need also to be coplanar? Is there a counterexample I'm missing? — Srap Tasmaner
I was imagining putting the point away from the plane and bending the underlying surface we're trying to draw the circle on. I'm pretty sure we'd end up with some other shapes possible if we inclined the plane, never mind if we corrugated the fucker.
But I suppose that would also apply if we chose the coplanar point far away from the candidate point set... I wish I knew what circles were. -
creativesoul
12.1kThe great circle is the circle I've highlighted on the surface of the sphere. Since the circle is confined to the surface of the sphere, and the surface of the sphere is not a plane, it is not a plane figure. — fdrake
If all circles are plane figures, then the great circle is not a circle.
Hueston, we have a problem. -
 fdrake
7.2kWe could also define a circle as the cross-section of a sphere, but I was only saying that every (planar) cross-section of a sphere will in fact fulfill the definition I already set out. — Leontiskos
fdrake
7.2kWe could also define a circle as the cross-section of a sphere, but I was only saying that every (planar) cross-section of a sphere will in fact fulfill the definition I already set out. — Leontiskos
It would if you give yourself the liberty of hammering the cross section down onto a flat plane. Which is an exercise of the imagination, and not something set out in Euclid's axioms. Is the point. You end up having to mathematise all the stuff you do to make it work. The operative distinction is you're relying on a lot of extra-mathematical intuition and not putting in the work to make it precise. Which is mostly fine, it's just in such imprecision where lots of allegedly undesirable plurality can hide.
Do trust me that the counterexamples work verbatim though! -
 Banno
29k
Banno
29k
No.You artificially inserted an extraneous conversation into your own thread and then invited me here, remember? — Leontiskos
I'm glad you dropped in, at Leon's invitation, I think?―― I don't know why I'm participating in this. — Srap Tasmaner
It wasn't I who engaged in necromancy - that was @frank. And you do not have to be here, if you find it too arduous.
Cheers. I'm glad someone looked at the Russell article.I'm gonna bugger off now too. — fdrake -
 Leontiskos
5.3kIt would if you give yourself the liberty of hammering the cross section down onto a flat plane. — fdrake
Leontiskos
5.3kIt would if you give yourself the liberty of hammering the cross section down onto a flat plane. — fdrake
I take it that a cross-section is flat (i.e. two-dimensional) by definition. But this all goes back to the ambiguity of your figure. If the cross-section you indicated is not two-dimensional then I would of course agree that it is not a circle. -
 fdrake
7.2kI'm glad someone looked at the Russell article. — Banno
fdrake
7.2kI'm glad someone looked at the Russell article. — Banno
I had comments I really wanted to make about the original article but considering that a Proofs and Refutations style chat about square circles was right there it seemed a better opportunity to illustrate the intuitions behind lemma incorporation.
Welcome to The Philosophy Forum!
Get involved in philosophical discussions about knowledge, truth, language, consciousness, science, politics, religion, logic and mathematics, art, history, and lots more. No ads, no clutter, and very little agreement — just fascinating conversations.
Categories
- Guest category
- Phil. Writing Challenge - June 2025
- The Lounge
- General Philosophy
- Metaphysics & Epistemology
- Philosophy of Mind
- Ethics
- Political Philosophy
- Philosophy of Art
- Logic & Philosophy of Mathematics
- Philosophy of Religion
- Philosophy of Science
- Philosophy of Language
- Interesting Stuff
- Politics and Current Affairs
- Humanities and Social Sciences
- Science and Technology
- Non-English Discussion
- German Discussion
- Spanish Discussion
- Learning Centre
- Resources
- Books and Papers
- Reading groups
- Questions
- Guest Speakers
- David Pearce
- Massimo Pigliucci
- Debates
- Debate Proposals
- Debate Discussion
- Feedback
- Article submissions
- About TPF
- Help
More Discussions
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum



