-
 TonesInDeepFreeze
3.8k
TonesInDeepFreeze
3.8k
Maybe you mean by analogy?
For example:
Check
(1) If Churchill was English then Churchill had a stiff upper lip
Churchill had a stiff upper lip
therefore Churchill was English
Compare with:
(2) If DeGaulle was German then DeGaulle was born in Lille
DeGaulle was born in Lille
therefore DeGaulle was German
(2) has true premises and a false conclusion. therefore (2) is invalid. but (2) is analogous in form with (1). so (1) is invalid -
 Count Timothy von Icarus
4.2kSuppose we have:
Count Timothy von Icarus
4.2kSuppose we have:
A → ~A
A
Therefore, B
In a logic with a relevance condition such that not everything follows from a falsehood. And suppose our logic also has removed disjunctive syllogism and disjunction introduction so that it is not explosive.
In this case, wouldn't it be true that:
-A premise is still necessarily false
-B does not follow from the premises by any inference even if both are assumed true?
The point being, the argument would be valid in the sense that it is impossible for the premises to both be true, but even if they were both true they wouldn't entail the conclusion anyhow.
But definitions of validity (e.g. IEP) very often will define it in both terms, i.e. that the conclusion cannot be false while the premises are all true and that the conclusion must follow from the premises or "be contained in them."
Wouldn't this be a case where the two are seperate? -
 Srap Tasmaner
5.2kyour reduction of material implication to set theory. I'm not sure how to understand that, really — Moliere
Srap Tasmaner
5.2kyour reduction of material implication to set theory. I'm not sure how to understand that, really — Moliere
It's not that complicated.
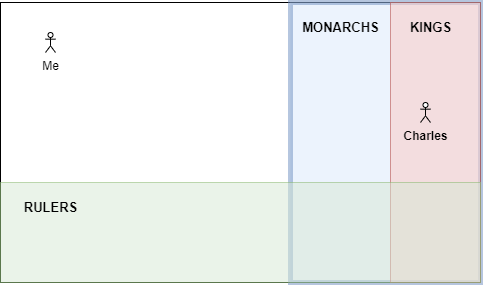
The whole space is people, say. Some are rulers, some monarchs, some kings, some none of those. A lot of monarchs these days are figureheads, so there's only overlap with rulers. All kings are monarchs, but not all monarchs are kings.
There are some things you can say about the probability of a person being whatever, and the ones we're interested in would be like this:
-
Pr(x is a monarch | x is a king) = 1
That is, the probability that x is a monarch, given that x is a king, is 1. The space of "being a king" is entirely contained in the space of "being a monarch".
-
King(x) → Monarch(x)
Similarly we can say
-
Pr(x is not a king | x is not a monarch) = 1
which is the contrapositive.
The complement of Monarchs is contained in the complement of Kings, but the latter also contains Queens and I don't know, Czars and whatnot. Not a king doesn't entail not a monarch, and sure enough Pr(x is a monarch | x is not a king) > 0.
Conceptually, that's it. (There are some complications, one of which we'll get to.)
I find the visualization helpful. We're just doing Venn diagram stuff here.
if the moon is made of green cheese then 2 + 2 = 4. That's the paradox, and we have to accept that the implication is true. How is it that the empirical falsehood, which seems to rely upon probablity rather than deductive inference, is contained in "2 + 2 = 4"? — Moliere
For this example, there's a couple things we could say.
Say you partition a space so that 0.000001% of it represents (G) the moon being made of green cheese, and the complement ― 99.999999% ― is it not (~G). Cool. Little sliver of a possibility over to one side.
2 + 2 = 4 is true for the entire space, both G and ~G. Both are contained in the space in which 2 + 2 = 4, which will keep happening whatever your empirical proposition because it's, you know, a necessary truth.
What's slightly harder to express is something we take to be necessarily false, like 2 + 2 = 5. The space in which that's true is empty, and the empty set is a subset of every single set, including both G and ~G. It could "be" anywhere, everywhere, or nowhere, doing nothing, not taking up any room at all. It doesn't have a specifiable "location" because Pr(2 + 2 = 5 | E) = 0 for any proposition E at all.
Both necessary truths and necessary falsehoods fail to have informative relations with empirical facts. -
 Srap Tasmaner
5.2k
Srap Tasmaner
5.2k -
 Banno
28.9k
Banno
28.9k
Leon and Hanover are more of an inspiration for Tones. They bring forth his best work.Only if you agree to write the preface. — Srap Tasmaner -
 Moliere
6.3kI find the visualization helpful. We're just doing Venn diagram stuff here. — Srap Tasmaner
Moliere
6.3kI find the visualization helpful. We're just doing Venn diagram stuff here. — Srap Tasmaner
Yes that's very helpful, thanks. I was getting lost in the idea of a probability space and how that relates to "contains in", but the visualization makes it quite literal and easy to comprehend.
So going back to
Ask yourself this: would "George will not open tomorrow" be a good inference? And we all know the answer: deductively, no, not at all; inductively, maybe, maybe not. But it's still a good bet, and you'll make more money than you lose if you always bet against George showing up, if you can find anyone to take the other side.
"George shows up" may be a non-empty set, but it is a negligible subset of "George is scheduled to open", so the complement of "George shows up" within "George is scheduled", is nearly coextensive with "George is scheduled". That is, the probability that any given instance of "George is scheduled" falls within "George does not show up" is very high. — Srap Tasmaner
The probability space here is the set of possible outcomes we've thus far observed and, under the assumption that the distribution over that probability space has not changed -- George hasn't converted to the church of punctuality, giving us a reason to believe the probability space has changed -- the good bet is he'll show up late.
EDIT:
Wrapping that back to the OP, now...
A -> ~A
A
Therefore ~A
The (probability) space of A is entirely contained within the (probability) space of not-A.
Well, of course it is. That's almost a restatement of the probability of P v ~P equals 1.
Not sure where I'm going with this, just thinking out loud more than anything. -
 Srap Tasmaner
5.2kThe (probability) space of A is entirely contained within the (probability) space of not-A.
Srap Tasmaner
5.2kThe (probability) space of A is entirely contained within the (probability) space of not-A.
Well, of course it is. That's almost a restatement of the probability of P v ~P equals 1. — Moliere
?
A and its complement ~A are disjoint. If A is contained in ~A, it must be ∅. -
 Moliere
6.3kI flip these values accidentally in my mind all the time. I could just be confusing myself. When stating it in terms of probability space my thought is that we can look at A and its negation as a probability space -- say a quarter that's fair has a 50 percent chance to land heads, and since there is only one other possibility (we could call it "Not-heads") we can deduce that not-heads' probability "is contained within", i.e. determined by, the probability of Heads.
Moliere
6.3kI flip these values accidentally in my mind all the time. I could just be confusing myself. When stating it in terms of probability space my thought is that we can look at A and its negation as a probability space -- say a quarter that's fair has a 50 percent chance to land heads, and since there is only one other possibility (we could call it "Not-heads") we can deduce that not-heads' probability "is contained within", i.e. determined by, the probability of Heads.
Sort of thinking about future events in analogue to the bag of different colored marbles -- George is late has 99 white marbles, George is on time has 15 red marbles, and George doesn't show up is 1 black marble. -
 Srap Tasmaner
5.2k"is contained within", i.e. determined by — Moliere
Srap Tasmaner
5.2k"is contained within", i.e. determined by — Moliere
Oh, not what I was saying at all.
The impetus for talking about this at all was the material conditional, and my suggestion was that you take P → Q as another way of saying that P ⊂ Q.
It helps me understand why false antecedents and true consequents behave the way they do.
Having gone that far, you might as well note that there are sets between ∅ and ⋃, and you can think of logic as a special case of the probability calculus.
That's how it works in my head. YMMV -
 Moliere
6.3kSo "P → Q" can be read as "P is contained within Q", and it makes sense of material implication because P can be empty set, which is a member of every set.
Moliere
6.3kSo "P → Q" can be read as "P is contained within Q", and it makes sense of material implication because P can be empty set, which is a member of every set.
Okay. I was twisting things around with probability because of the example, but they're not related. -
 TonesInDeepFreeze
3.8kP can be empty set, which is a member of every set. — Moliere
TonesInDeepFreeze
3.8kP can be empty set, which is a member of every set. — Moliere
You mean it is a subset of every set (the empty set is not a member of every set).
Not a correction, but a reminder: We prove that the empty set is a subset of every set by using the material conditional:
Show for all S and x, if x is in 0 then x is in S:
It is not the case that x is in 0, so if x is in 0 then x is in S.
I haven't followed all of your conversation, so this might not be pertinent, but if it is, it is good to keep in mind: So, if '->' is construed as in terms of subsets to make sense of the material conditional and vacuous instances, then that tack would be circular, since the empty set being a subset of every set is based on the material conditional. But of course, we could say the notions are compatible, though that is no surprise. -
 Srap Tasmaner
5.2k
Srap Tasmaner
5.2k
Yeah that's a funny thing. Mathematics cannot be reduced to logic, it turns out, but it appears to have an irremediable dependency on logic.
Sometimes it suggests to me that mathematics and logic are both aspects or expressions of some common root.
Anyway, much as I would like for probability to swallow logic, I'm resigned to mostly taking the sort of stuff I've been posting as a kind of heuristic, or maybe even a mathematical model of how logic works. (I have some de Finetti to read soon, so we'll see what he has to say.)
By the way, I understand the main focus for unifying math and logic in recent years has been in category theory, which I haven't touched at all. Is that something you've looked into? -
 Srap Tasmaner
5.2kP can be empty set, which is a member of every set. — Moliere
Srap Tasmaner
5.2kP can be empty set, which is a member of every set. — Moliere
This is a correction ― not a member, but a subset.
A nitpick, for sure, but making exactly that distinction took a long time, and there were questions that remained very confusing until those concepts were clearly separated. -
 Moliere
6.3kOh, nitpick away. I don't pretend to have a mastery here -- just an interested person who doesn't mind being corrected.
Moliere
6.3kOh, nitpick away. I don't pretend to have a mastery here -- just an interested person who doesn't mind being corrected. -
 TonesInDeepFreeze
3.8k
TonesInDeepFreeze
3.8k
Your probability exploration is interesting. I think there's probably (pun intended) been a lot of work on it that you could find.
Indeed, logic and mathematics - chicken and the egg.
I am not up to speed on category theory though I know some of its basics. One problem I've had is finding an axiomatization. However, ZFC+"exists an infinite cardinal" is an axiomatization of category. So, as far as I can tell, category theory does not eschew set theory but rather, and least to the extent of interpretability (different sense of 'interpretation' in this thread) it presupposes it and goes even further. -
 Srap Tasmaner
5.2kYour probability exploration is interesting. I think there's probably (pun intended) been a lot of work on it that you could find. — TonesInDeepFreeze
Srap Tasmaner
5.2kYour probability exploration is interesting. I think there's probably (pun intended) been a lot of work on it that you could find. — TonesInDeepFreeze
Indeed. I'd have to check, but I think Ramsey used to suggest that probability should be considered an extension of logic, "rather" (if that matters) than a branch of mathematics. It's an element of the "personalist" interpretation he pioneered and which de Finetti has probably contributed to the most. I'm still learning.
So, as far as I can tell, category theory does not eschew set theory but rather, and least to the extent of interpretability (different sense of 'interpretation' in this thread) it presupposes it and goes even further. — TonesInDeepFreeze
Yeah not clear to me at all. A glance at the wiki suggests there have been efforts to replace set theory entirely, but I'm a font of ignorance here.
On the other side, it did catch my eye when some years ago Peter Smith added an introduction to category theory to his site, Logic Matters. One of these days I'll have a look. -
 TonesInDeepFreeze
3.8k
TonesInDeepFreeze
3.8k
Then let me nitpick that. You didn't mean 'nitpick' pejoratively, but subset vs member is not a nitpick, and the point about circularity is a good catch. -
 Srap Tasmaner
5.2k
Srap Tasmaner
5.2k
One other tiny point of unity: I always thought it was interesting that for "and" and "or" probability just directly borrows ∩ and ∪ from set theory. These are all the same algebra, in a sense, logic, set theory, probability. -
 Srap Tasmaner
5.2ksubset v member — TonesInDeepFreeze
Srap Tasmaner
5.2ksubset v member — TonesInDeepFreeze
I should also have mentioned that it matters because ∅ has no members but ∅ ⊂ ∅ is still true, in keeping with how the material conditional works. -
 TonesInDeepFreeze
3.8kreplace set theory entirely — Srap Tasmaner
TonesInDeepFreeze
3.8kreplace set theory entirely — Srap Tasmaner
I'm not expert either. But my understanding is that yes, category theory couches mathematics in different terms from set theory, and thus provides a different way of thinking, but category theory is inter-interpretable with ZFC+"exists an inaccessible cardinal"*. And ZFC+"exists an inaccessible cardinal" provides a model of ZFC, so it is a quite strong theory in that sense.
* But I have never been able to find what that really means. I know what interpretability is. And I know what ZFC+"exists and inaccessible cardinal" is. But interpretability is between two theories, but what exactly is the theory category theory that is inter-interpretable with ZFC+"exists and inaccessible cardinal"?
/
Peter Smith offers some nice content. And he used to post at sci.logic, but, if I recall correctly, got disgusted with all the cranks. -
 TonesInDeepFreeze
3.8kOne other tiny point of unity: I always thought it was interesting that for "and" and "or" probability just directly borrows ∩ and ∪ from set theory. These are all the same algebra, in a sense, logic, set theory, probability. — Srap Tasmaner
TonesInDeepFreeze
3.8kOne other tiny point of unity: I always thought it was interesting that for "and" and "or" probability just directly borrows ∩ and ∪ from set theory. These are all the same algebra, in a sense, logic, set theory, probability. — Srap Tasmaner
The duals run all through logic and mathematics. The main result concerning propositional logic is that there is an isomorphism between propositional logic and the Tarski-Lindenbaum algebra (a particular Boolean algebra). Then Tarski also showed an isomorphism between predicate logic and cylindrical algebra (the details of cylindrical algebra are beyond mere) -
 TonesInDeepFreeze
3.8kAnother friendly picking of nits: Some writers use the word 'contained'; it is not wrong. But sometimes I see people being not clear whether it means 'member' or 'subset', so I don't use the word. I just say 'member of' or 'subset of' as suited.
TonesInDeepFreeze
3.8kAnother friendly picking of nits: Some writers use the word 'contained'; it is not wrong. But sometimes I see people being not clear whether it means 'member' or 'subset', so I don't use the word. I just say 'member of' or 'subset of' as suited. -
 Srap Tasmaner
5.2k0 subset of 0 holds by P -> P. — TonesInDeepFreeze
Srap Tasmaner
5.2k0 subset of 0 holds by P -> P. — TonesInDeepFreeze
I've granted that mathematics is dependent upon logic ― but, for the sake of argument, are you sure this is right?
That is, we need logic in place to prove theorems from axioms in set theory, to demonstrate that ∅⊂∅, for instance, but do we want to say it's because of the proof that it is so?
This close to the bone, I'm not sure how much we can meaningfully say, but something about "holds by" ― rather than, "is proved using" ― looks wrong to me.
Am I missing something obvious?
Peter Smith offers some nice content. — TonesInDeepFreeze
I used to enjoy reading his reviews of logic textbooks, because he was very picky about how they presented logic schemas and the process of "translating" natural language into P's and Q's. Unforgiving when authors were too slapdash or handwavy about this, which I thought showed good philosophical sense.
Oh, yes, the duals run all through mathematics. — TonesInDeepFreeze
Just the sort of thing, I understand, that motivates category theory.
#
Honestly, I'm not quite sure why formal logic (mathematical logic) isn't just considered part of mathematics. It would be part of foundations, to be sure, as set theory is, and you need it in place to bootstrap the rest, as you have to have sets (or an equivalent) to do much of anything in the rest of mathematics, but so what? What does mathematics get out of pretending it's importing logic from elsewhere? -
 Srap Tasmaner
5.2kWriters often used the word 'contained'; it is not wrong. But sometimes I see people being not clear whether it means 'member' or 'subset' — TonesInDeepFreeze
Srap Tasmaner
5.2kWriters often used the word 'contained'; it is not wrong. But sometimes I see people being not clear whether it means 'member' or 'subset' — TonesInDeepFreeze
That's a solid point. It felt natural and intuitive when talking about "areas", subspaces of a partitioned probability space, and so on. But it's an awful word, as @Moliere proved. -
 TonesInDeepFreeze
3.8kare you sure this is right? — Srap Tasmaner
TonesInDeepFreeze
3.8kare you sure this is right? — Srap Tasmaner
Absolutely sure.
If x in 0 then x in 0.
That's an instance of P -> P.
do we want to say it's because of the proof that it is so? — Srap Tasmaner
I don't opine as to 'because'.
"holds by" ― rather than, "is proved using" — Srap Tasmaner
I meant 'is proved by'. I find that the word 'holds' is used in at least two senses in mathematics: (1) is true, (2) is proven. But with set theory, 'true' could be taken as 'true in any model of the set theory axioms'. So "0 subset of 0" is proven by deploying "P -> P" and it is also true by deploying "P -> P" (given the soundness theorem in this version: if a sentence is provable from a set of axioms then the sentence is true in any model of the axioms). -
 TonesInDeepFreeze
3.8kUnforgiving when authors were too slapdash or handwavy about this, which I thought showed good philosophical sense. — Srap Tasmaner
TonesInDeepFreeze
3.8kUnforgiving when authors were too slapdash or handwavy about this, which I thought showed good philosophical sense. — Srap Tasmaner
Yet, often censoriously regarded as bad philosophical sense in The Philosophy Forum. -
 TonesInDeepFreeze
3.8kmotivates category theory — Srap Tasmaner
TonesInDeepFreeze
3.8kmotivates category theory — Srap Tasmaner
Category theory centers on arrows, and as involving functions, composition of functions and morphisms and things. -
 fdrake
7.2kWhat does mathematics get out of pretending it's importing logic from elsewhere? — Srap Tasmaner
fdrake
7.2kWhat does mathematics get out of pretending it's importing logic from elsewhere? — Srap Tasmaner
Someplace to start writing without having to explain yourself. I honestly think that's it.
Welcome to The Philosophy Forum!
Get involved in philosophical discussions about knowledge, truth, language, consciousness, science, politics, religion, logic and mathematics, art, history, and lots more. No ads, no clutter, and very little agreement — just fascinating conversations.
Categories
- Guest category
- Phil. Writing Challenge - June 2025
- The Lounge
- General Philosophy
- Metaphysics & Epistemology
- Philosophy of Mind
- Ethics
- Political Philosophy
- Philosophy of Art
- Logic & Philosophy of Mathematics
- Philosophy of Religion
- Philosophy of Science
- Philosophy of Language
- Interesting Stuff
- Politics and Current Affairs
- Humanities and Social Sciences
- Science and Technology
- Non-English Discussion
- German Discussion
- Spanish Discussion
- Learning Centre
- Resources
- Books and Papers
- Reading groups
- Questions
- Guest Speakers
- David Pearce
- Massimo Pigliucci
- Debates
- Debate Proposals
- Debate Discussion
- Feedback
- Article submissions
- About TPF
- Help
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum





