However, I'm not sure about the left drawing. It doesn't show that mammals are a subset of animals. It shows that only a part of mammals are also animals. — Alkis Piskas

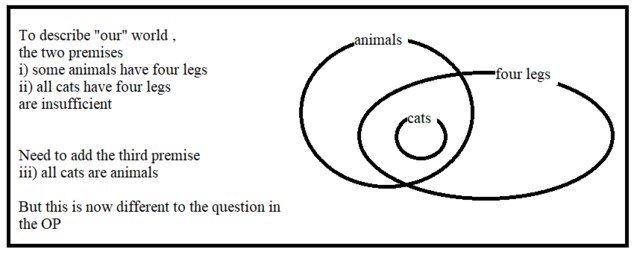
I agree that the left hand drawing is not correct for "our" world, where i) all mammals are animals (all B's are A's) ii) all cats are mammals (all C's are B's)

But the OP is not asking a question about "our" world. The OP is asking a question about a "possible" world, perhaps a fictional world, where i) some animals are mammals (some A's are B's) ii) all cats are mammals (all C's are B's), in which case the left hand drawing is correct.

Certainly. But I have edited my reply and gave you right after I checked the label "possible world" ... Also, I presented a more interesting and realistic scheme ...
See the update at https://thephilosophyforum.com/discussion/comment/646385

So let's see our statemets:
A) Some animals are cats: Unknown
B) Some cats have four legs: False, since we know that "All cats have four legs" (and not only some)
C) No cats are animals: "No cats" is ambiguous - At best, it's Unknown, based on (A)
D) No animals that have not four legs are cats: "No animals" is ambiguous - Assuming that it means "none of the animals" then it is True, since cats have four legs
E) None of the above conclusions can be drawn: False, if we can accept (D) as True, else True.

It all depends on (D). And this also explains the doubt of the OP, who was not sure about (D) or (E). — Alkis Piskas

A) ??
B) ??
C) ??
D) ??
E) ??

The "??" means you need a course in basic logic. And reading. The problem was not about what is or is not true, but what conclusions can be drawn, two different animals.

Some animals have four legs
All cats have four legs — Alkis Piskas

In what the OP said, I have replaced "Ayes" with "animals", "Bees" with "four legs" and "Seas" with "cats". All the rest is the same. The conclusion (D or E) is what the OP also thought (maybe for another reason though).

If you have a dollar in your pants pocket, do you (not) have also 32 cents?
— tim wood
No, you don't. A dollar is a dollar and cents are cents. Also, you cannot use some vending, gambling etc. machines if you don't have the exact amount of cents. — Alkis Piskas

That is ridiculous captiousness. The example is not vitiated by quibbles about the difference between coins and bills. The point of the example is that you can have Some and also All.

Again, I pointed out to you that in the context of basic logic, 'some' means 'at least one' and doesn't mean 'some but not all'.

it is true because its inference is valid — Alkis Piskas

To be clear, a valid inference does not ensure the truth of the conclusion. A valid inference does ensure the truth of the conclusion when either (1) the premises are true or (2) the conclusion is logically true anyway.

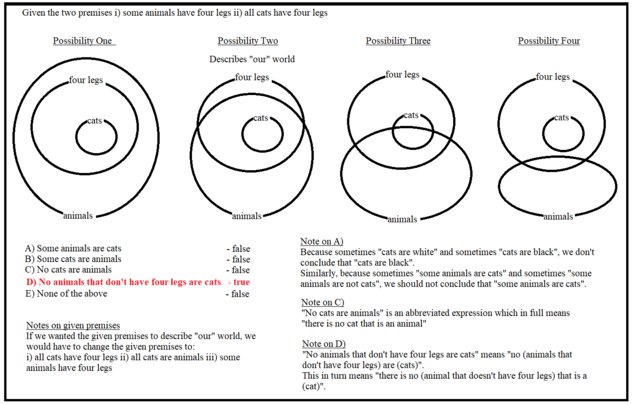
A) Some animals are cats: True, since mammals are animals (based on the first premise) and cats are mammals
— Alkis Piskas
You can infer this adding additional information, but you cannot from the premises given validly conclude it.
— tim wood
You are right that you have to infer it, i.e. we don't know that directly, but it is true because its inference is valid, — Alkis Piskas

No, your inference is not valid. It is true that some animals are cats, but it does not follow from your premises.

Mammals are a subset of animals. Cats are a subset of mammals. That is, cats are a subset of a subset of animals. — Alkis Piskas

Yes, that is valid. But that is different from your original argument.

It is true that some animals are cats. But it is not entailed by your premises.
— TonesInDeepFreeze
If it is true, well, it is True! That's what I said! — Alkis Piskas

No, what you said is that it follows from your premises.

Saying that "some cats are mammals" suggests that there are some cats that are not mammals. — Alkis Piskas

In certain everyday contexts, yes, 'some' may mean or at least suggest 'not all'. But not in the study of basic logic. I'll say it again: In ordinary basic logic [also, in certain other everyday contexts]
'some' means 'at least one' and it doesn't mean 'some but not all'.

(A) is true, but it is not entailed by your premises.
— TonesInDeepFreeze
Yes, you have already said that! — Alkis Piskas

Yet you made the same mistake in a subsequent post!

B) Some cats have four legs: False, since we know that "All cats have four legs" (and not only some) — Alkis Piskas

Wrong. For the fourth time, I'm telling you that in basic logic 'some' means 'at least one' and not 'some but not all'.

"No animals" is ambiguous — Alkis Piskas

I pointed out before that that is wrong. You merely persist in claiming again what has already been explained to you to be incorrect.

In basic logic:

"Some cats are mammals"
means
"There is a thing that is both a cat and a mammal"

"All cats are mammals"
means
"If a thing is a cat then it is a mammal"

"No cats are mammals"
means
"If a thing is a cat then it is not a mammal"
means
"All cats are not mammals"

"Some cats are not mammals"
means
"There is a thing that is a cat and is not a mammal"

There is no ambiguity.

/

Moreover:

"Some cats are mammals" does NOT imply "Some cats are not mammals".

"All cats are mammals" does NOT imply 'Some cats are mammals" (because, if there are no cats, then "All cats are mammals" is vacuously true but "Some cats are mammals" is false). (Though I don't recall what, if anything, Aristotle said about that; and, while the notion of vacuous truth is basic in usual formal logic, it is not ordinarily used in everyday logic.)

Equivalences:

"It is not the case that some cats are mammals"
is equivalent to
'No cats are mammals"

"It is not the case that all cats are mammals"
is equivalent to
"Some cats are not mammals"

"It is not the case that no cats are animals"
is equivalent to
"Some cats are mammals"

"It is not the case that some cats are not mammals"
is equivalent to
"All cats are mammals"

"All cats are mammals" does NOT imply 'Some cats are mammals" (because, if there are no cats, then "All cats are mammals" is vacuously true but "Some cats are mammals" is false). (Though I don't recall what, if anything, Aristotle said about that; and, while the notion of vacuous truth is basic in usual formal logic, it is not ordinarily used in everyday logic.) — TonesInDeepFreeze

This goes to the existential problem, and apparently it has been a problem for at least since A. I find this online: it seems about right.

The presupposition, mentioned above, that all categories contain at least one thing, has been abandoned by most later logicians. Modern logic deals with uninstantiated terms such as “unicorn” and “ether flow” the same as it does other terms such as “apple” and “orangutan”. When dealing with “empty categories”, the relations of being contrary, being subcontrary and of subalternation no longer hold. Consider, e.g., “all unicorns have horns” and “no unicorns have horns.” Within contemporary logic, these are both regarded as true, so strictly speaking, they cannot be contrary, despite the former’s status as an A proposition and the latter’s status as an E proposition. Similarly, “some unicorns have horns” (I) and “some unicorns do not have horns” (O) are both regarded as false, and so they are not subcontrary. Obviously then, the truth of “all unicorns have horns” does not imply the truth of “some unicorns have horns,” and the subalternation relation fails to hold as well. Without the traditional presuppositions of “existential import”, i.e., the supposition that all categories have at least one member, then only the contradictory relation holds. On what is sometimes called the “modern square of opposition” (as opposed to the traditional square of opposition sketched above) the lines for contraries, subcontraries and subalternation are erased, leaving only the diagonal lines for the contradictory relation."

Which goes to my admonition to check house rules before wagering.

This goes to the existential problem — tim wood

Yes,that matter hinges on existential import. But the problem in the first post of this thread does not. Nor does your other concern about undistributed middle.

"The presupposition [...] contradictory relation." — tim wood

All of that quote seems correct to me and it in no way vitiates anything I've said, and it in no way supports your notion that the question of this thread hinges on existential import or undistributed middle.

house rules — tim wood

Of course, discussions about drawing inferences need to be in context of what principles of logic we have in mind. But the question in this thread has been answered according to everyday principles of reasoning, which also are formalized. And those particular principles do not hinge in any way on matters of existence or vacuity. I have explained exactly why that is in this case. I don't know why you continue to ignore it.

You mentioned cutting a knot with a knife. I rebutted that analogy already. But with your fixation that existential import plays a role in the particular question of this thread, you remind me of the saying that if a person has only a hammer then everything looks like a nail.

You do not seem to have grasped that I have yielded the point. Why is that? Not looking for an answer.

Because you never said you gave it up; and your next post seemed to still be trying to connect existential import to what we had been discussing. Granted, it is also reasonable that you were not trying to make the earlier connection, in which case I would grant my previous post would have been beating a dead horse.

I say A. If some ayes are bees, and seas are bees, then some ayes are sees. Unless not all bees are sees, which isn't given.

There are Venn diagrams in this thread, or you can draw your own. Do that and you will see where you're going wrong.

If some ayes are bees, and seas are bees, then some ayes are sees. Unless not all bees are sees, which isn't given. — John McMannis

Yes, "Not all Beas are Seas" is not a premise. But "All Beas are Seas" is also not a premise. So you don't get to use either in the inference.

An inference is not valid when there is an example in which the premises are true and the conclusion is false. Here's an example.

Let the set of Ayes be {Jack}

Let the set of Beas be {Jack, Lucy}

Let the set of Seas be {Lucy}

So:

"Some Ayes are Bees" True
"All Seas are Bees" True

"Some Ayes are Seas" False

So the inference is invalid.

In what the OP said, I have replaced "Ayes" with "animals", "Bees" with "four legs" and "Seas" with "cats". All the rest is the same. The conclusion (D or E) is what the OP also thought (maybe for another reason though). — Alkis Piskas

I have redrawn my Venn Diagram, including @Raymond and @tim wood's suggestions, and using animals, etc rather than Ayes, etc. The solution is still D.

OK, D is the winner! Case closed! :sweat:

D is the winner! — Alkis Piskas

:up:

:pray: + :up:

I believe there's no need to rack our brains on such a simple matter.

1. Some Ayes are Bees.
2. All Seas are Bees.

No conclusion follows.

1. Some colors are white.
2. All snow are white.
Ergo,
???

there's no need to rack our brains on such a simple matter. — Agent Smith

Yes, it is a simple matter that (D) is the correct answer.

1. Some Ayes are Bees.
2. All Seas are Bees.

No conclusion follows. — Agent Smith

No, many conclusions follow. And one of them is:

No Ayes that are not Bees are Seas.

Moreover, it follows from "All Seas are Beas" alone.

And one shouldn't have to rack one's brain to see that, except you still haven't racked your brain enough.

Yes, it is a simple matter that (D) is the correct answer. — TonesInDeepFreeze

Of course. :roll:

:roll: — jgill

I don't know what to make of people who still can't see it after it's been explained six ways to Sunday.

:up: Good that you cleared that up for me.

In my defense though, I wasn't looking at immediate inferences like you are; rather I was trying to see if the two statements could be used to form a classic syllogism; they can't!

I was trying to see if the two statements could be used to form a classic syllogism — Agent Smith

Yes, we went through that with tim wood. Anyway, glad that you see now that (D) is the answer.

Well, to tell you the truth, I don't get it. Anyway, gimme some time...

Good day!

Try it this way:

Some Americans are Brainy.
All Statisticians are Brainy.

We want to prove:

No American that is not Brainy is a Statistician.

But "No American that is not Brainy is a Statistician" means the same as "If something is an American and not Brainy, then it is not a Statistician."

Now, since, all Statisticians are Brainy, it follows that if something is not Brainy then it is not a Statistician. So, perforce, if something is an American and not Brainy, then it is not a Statistician. QED.

But what about the premise "Some Americans are Brainy"? Well, we never used it. We didn't need to. Which is fine. If a statement (such as "All Statisticians are Brainy") proves a conclusion, then that statement plus any other extra unneeded statement (such as "Some Americans are Brainy") still proves the conclusion (this is the principle of Monotonicity of Entailment).

Well, to tell you the truth, I don't get it — Agent Smith

Heh, Heh. :wink:

Heh, Heh — jgill

:smile: It's simply impossible!

It's simply impossible! — Agent Smith

It's impossible for you to understand?

What is the first sentence in my previous post that you don't understand?