-
 Pfhorrest
4.6kIn another thread I began a project to lay out the construction of complicated mathematical objects such as special unitary groups out of bare, empty sets. I set out to do this because that special unitary group is considered by contemporary theories of physics to be the fundamental kind of thing that the most elementary physical objects, quantum fields, are literally made of. Excitations of those quantum fields, which is to say particular states of those special unitary groups, constitute the fundamental particles of physics, which combine to make atoms, molecules, stars, planets, living cells, and organisms, including us.
Pfhorrest
4.6kIn another thread I began a project to lay out the construction of complicated mathematical objects such as special unitary groups out of bare, empty sets. I set out to do this because that special unitary group is considered by contemporary theories of physics to be the fundamental kind of thing that the most elementary physical objects, quantum fields, are literally made of. Excitations of those quantum fields, which is to say particular states of those special unitary groups, constitute the fundamental particles of physics, which combine to make atoms, molecules, stars, planets, living cells, and organisms, including us.
The point of that thread is to illustrate specifically how, in a very distant way, we ourselves can be said to be made of empty sets. (And as all of the truth functions, and so all the set operations, and all the other functions built out of set operations, can be built out of just conegation, and the objects they act upon are built up out of empty sets, everything can in a sense be said to be made out of negations of nothing). In the same way that when we can construct a series of sets that behave exactly like the natural numbers and so are indistinguishable and thus identical to them, so too can we construct complicated mathematical objects that behave indistinguishably from the fundamental constituents of reality and so are, for all intents and purposes, identical to them.
But that thread is looking to be going into much deeper detail on the specific construction of that than I originally intended. (See this post there for my high-level overview of what I expected it to turn out like). So I'd like to start another thread specifically to discuss the philosophical principle underlying that one, mathematicism -- the view that physical stuff is literally made out of mathematical stuff. And I'd like to discuss it as an alternative position on the topic of the existence or reality of abstract objects, opposite of both platonism and nominalism.
It is not a special feature of contemporary physics that says reality is made of mathematical objects; rather, it is a general feature of mathematics that whatever we find things in reality to be doing, we can always invent a mathematical structure that behaves exactly, indistinguishably like that, and so say that the things in reality are identical to that mathematical structure. If we should find tomorrow that our contemporary theories of physics are wrong, it could not possibly prove that those features of reality are not identical to some mathematical structure or another; only that they are not identical to the structures we thought they were identical to, and we need to better figure out which of the infinite possible structures we could come up with it is identical to. We just need to identify the rules that reality is obeying, and then define mathematical objects by their obedience to those same rules. It may be hard to identify what those rules are, but we can never conclusively say that reality simply does not obey rules, only that we have not figured out what rules it obeys, yet.
The mathematics is essentially just describing reality, and whatever reality should be like, we can always come up with some way of describing it. One may be tempted to say that that does not make the description identical to reality itself, as in the adage "the map is not the territory". In general that adage is true, and we should not arrogantly hold our current descriptions of reality to be certainly identical to reality itself. But a perfectly detailed, perfectly accurate map of any territory at 1:1 scale is just an exact replica of that territory, and so is itself a territory in its own right, indistinguishable from the original; and likewise, whatever the perfectly detailed, perfectly accurate mathematical of reality should turn out to be, that mathematical model is a reality: the features of it that are perfectly detailed, perfectly accurate models of people like us would find themselves experiencing it as their reality exactly like we experience our reality. Mathematics "merely models" reality in that we don't know exactly what reality is like and we're trying to make a map of it. But whatever model it is that would perfectly map reality in every detail, that would be identical to reality itself. We just don't know what model that is.
There necessarily must be some rigorous formal (i.e. mathematical) system or another that would be a perfect description of reality. The alternative to reality being describable by a formal language would be either that some phenomenon occurs, and we are somehow unable to even speak about it; or that we can speak about it, but only in vague poetic language using words and grammar that are not well-defined. I struggle to imagine any possible phenomenon that could cause either of those problems. In fact, it seems to me that such a phenomenon is, in principle, literally unimaginable: I cannot picture in my head some definite image of something happening, yet at the same time not be able to describe it, as rigorously as I should feel like, not even by inventing new terminology if I need to. At best, I can just kind of... not really definitely imagine anything in particular.
All of this is building up to me addressing the central question in the philosophy of mathematics, which is about the existence of abstract objects, like numbers and such. There are two main answers to that question, and some positions intermediate to the two, but I want to offer a position that I consider to be off of that spectrum entirely.
One of the usual two positions is Platonism, sometimes called either Platonic realism or Platonic idealism, which holds that abstract objects, or as Plato called them "forms" or "ideas", are real in the same sense that concrete objects, like rocks and trees and tables and chairs, are real; but that they don't exist in our space and time, and instead live in some separate, spaceless, timeless realm, from which they somehow interact with the things in our realm that "partake" of them, in the way that a triangular rock "partakes of the form of the triangle". It is held by Platonists that the existence, in some way, of these abstract objects is necessary in order for mathematical and other abstract statements that seem nominally to be about them to be true: for instance, the Pythagorean theorem which describes the relations of the legs of a right triangle to the length of its hypotenuse is not made true by the existence of any particular triangular objects, but rather by facts about the form of triangles generally, even if no concrete triangular objects existed at all.
I am not very amenable to this position at all, holding it to fall heavily afoul of the principles I've laid out extensively before against the position I call "transcendentalism".
The second of the usual two positions is called nominalism, which holds that abstract objects are merely empty names, that do not refer to real things that exist at all, and are just names for the similar properties of, and collections of, particular concrete objects. I am much more amenable to that position generally, but I think that a kind of existence can nevertheless be applied to abstract objects after all, a kind of existence abstracted away from the more familiar notion of concrete existence.
In the most restricted sense, one could say "only what I am experiencing right here right now exists". Everything else that we talk about existing is some degree of inference and abstraction away from that. There is a position in the philosophy of time, called presentism, that holds that only the present exists, neither the past nor the future. I agree with them to the extent that in a sense only the present exists: only the present presently exists, right now. But a part of what I'm experiencing right now in the present is memory, from which I infer (automatically, intuitively, without thinking about it) the existence of other times, having an experience of moving between different times, from those remembered past times and toward projected future times, and there is a perfectly serviceable sense in which I can say that those other times "exist" in a timeless sense of the word: they don't exist now, presently, for sure, but they still exist at other times.
And in that "movie", so to speak, of my past, present, and future experiences that I have now inferred, I have the experience of seeming to move around different places, so I further infer that other places exist too, besides just the here that I am experiencing now. Like with presentism, only the place I am at exists here, but those other places can still reasonably be said to exist elsewhere.
In this way, a spatiotemporal kind of existence is already abstracted away from the more primitive kind of existence relevant to my local, present experiences. But beyond that, some philosophers such as David Lewis hold, and I agree, that other possible worlds, like the kind that we use to make sense of talk of alethic modalities like necessity and possibility, really exist, and aren't just useful fictions, even though they don't actually exist, because "actual" is an indexical term like "present" or "local": it refers to things relative to the person using the word. Just as other times don't presently exist but are still real in a more abstract sense, so too, on this account, other possible words don't actually exist, because "actually" means "in the possible world I am a part of", but they are nevertheless still real in a still more abstract sense.
Likewise, to finally get on to my point about the existence of mathematical objects, since we can in principle equate our concrete universe with some mathematical structure or another, and that mathematical structure definitely concretely exists (because it just is the concrete universe), we can say that other mathematical structures, i.e. abstract objects, don't concretely exist — because "concretely" is indexical, like "actually", it means "as a part of the mathematical structure that is our universe" — but they can nevertheless be reasonably called "real" in some even broader sense, the most abstract sense possible: they abstractly exist. This position is held by physicist Max Tegmark, and he calls it the "ultimate ensemble"; it is more broadly called the mathematical universe hypothesis, or mathematicism, and it has precursors tracing back to the Pythagorean philosophers of ancient Greece.
This kind of existence for abstract objects does not run afoul of my position against transcendentalism the way that Platonism does, because the abstract objects don't exist in some wholly different kind of way separate from the kind of concrete objects that we can empirically observe. They are just the loosest part of the broader framework of explanation for our empirical observations. We cannot directly observe other times or places, only the local present, but postulating the existence of other times and places helps to explain the patterns in our local, present experiences. Those other times and places aren't held to be discontinuous or of a completely different nature than the local present, they are just postulated extensions of the here and now. Likewise, I hold, with postulating other possible worlds, continuous with the one we find ourselves in and of same nature as it; and also likewise with other abstract objects besides whichever one is identical with the concrete universe, continuous with it and of the same nature as it.
But still, that last step into abstract rather than concrete existence is a significant one. This view of the relation between the concrete and abstract bears a similarity to what Immanuel Kant called the phenomenal and the noumenal, where on his account we cannot ever have direct experiential contact with noumena, but instead only project our ideas about them behind the world of phenomena that we experience, much like how on my account the truly abstract has no direct influence on the concrete world we experience, and we can only project our ideas of abstract objects behind that concrete world in an attempt to understand and explain it.
In relation to platonism and nominalism, we might say that while the platonist affirms the reality of two kinds of existence, and the nominalist denies the reality of one of those kinds, mathematicism like mine outline here instead denies that there are two wholly separate kinds at all, holding the latter kind (the kind the nominalist affirms) to be merely a subset of the former kind (the kind the nominalist denies). Rather than there being no abstract objects, or both abstract objects and concrete objects, on this account all concrete objects are but parts of a single abstract object -- the abstract object of which we are a part, namely the concrete universe. -
 bongo fury
1.8kA visual map can be encoded in binary on a computer, and a human could read off those ones and zeros, even if they didn’t understand what they were reading. All the information in the picture would be retained in the sound of the human voice.
bongo fury
1.8kA visual map can be encoded in binary on a computer, and a human could read off those ones and zeros, even if they didn’t understand what they were reading. All the information in the picture would be retained in the sound of the human voice.
— Pfhorrest
So this seems to me an excellent example of the obvious differences to be found between an object (whatever it was, a still life?) and its representation or description (the vocalised bit map). The map is certainly not the territory.
If that picture were to be perfectly detailed down to the subatomic level, it would have to be animated
— Pfhorrest
If you mean represent temporally successive states, gradients etc. then, sure. If you mean represent them by a temporal succession of symbols, then surely not? Why? (I know the bit map is vocalised as a succession, but thus far that aspect was irrelevant to what it described, and could continue to be so, I would have assumed.)
or at least include temporal information in it like momentum, and all of the structural details that give a complete picture of its function,
— Pfhorrest
Sure, why not. We're on a flight of fancy as regards the level of precision achieved by the description, but that's ok. Bolt on another hard drive (or immortal chanter) to store the whole bit-map.
and contain within it all the exact information that the physical thing the “picture” it is of does.
— Pfhorrest
(Interesting syntax... reminds me of "no head injury is too trivial to be ignored" ;) )
Do you mean, "the physical thing that the picture is (a picture) of: the thing it depicts; the bowl of fruit?
Ok, the picture/bit-map/description must be as complex as the physics of a bowl of fruit; but was this paragraph meant to show how the bit-map must become a replica of the bowl of fruit? That's what I'm not getting. — bongo fury
Talking about a literal map of a city is probably a clearer illustration. — Pfhorrest
Evidently not. How or why must the ever more elaborate vocal performance which is the bit-map become more and more like the bowl of fruit? -
 Tristan L
187That’s really an interesting idea. When I first learned of the Pythagorean saying All is Rimetale (Number) as a child, I was spellbound by it at once. Even before that, I was sure of the soothness (realness) of numbers and other mathematical things. Of course, my philosophy has greatly evolved since then.
Tristan L
187That’s really an interesting idea. When I first learned of the Pythagorean saying All is Rimetale (Number) as a child, I was spellbound by it at once. Even before that, I was sure of the soothness (realness) of numbers and other mathematical things. Of course, my philosophy has greatly evolved since then.
I like your basic idea, as you can likely see from what I said in the discussion about the not-inventableness of ideas, such as:
I think that things stand as follows: All things are abstract and therefore eche and uncreatable, but information can be created (though not destroyed). — Tristan L
and maybe this comment of mine.
However, I do find issues with some of your points:
The second of the usual two positions is called nominalism, which holds that [...]. I am much more amenable to that position generally — Pfhorrest
I, on the other hand, am strongly against nominalism and find it self-refuting, for the proposition that there are no Shapes (Forms, Ideas, wideas) means that Shapehood has no instances, for which at least the Shape of Shapehood itself is needed.
What’s the problem with beyondness? Both, the Shape of Mindhood, as well as each and every mind, belongs to the beyondly abstract realm. Moreover, the mind can directly “see” many of the abstract things directly with “the mind’s eye”, giving it true knowledge of the abstract, as opposed to mere opinions about the concrete. I, for one, can “see” the abstract widea of mindhood, my own mind, and the rimetale 4, but I cannot directly see any concrete ‘entity’. Thoughtcasters (telepaths) can even directly see other minds, but I doubt that there are any true thoughtcasters in our world.I am not very amenable to this position [platonism] at all, holding it to fall heavily afoul of the principles I've laid out extensively before against the position I call "transcendentalism". — Pfhorrest
Also, the upspring (orspring, origin) of beon itself (see this comment of mine to resolve the problem of the two meanings of “being”), not-beon itself, as well as all beondes, must itself be above both beon and not-beon. This is quite simple to see, which is why I don’t understand why the debate between the (in my opinion) equally meaningless positions of theism and atheism is still raging. To back up my claim that it is simple to see:
- Only a few hundred years after the birth of Western philosophy, Plato already realised than Goodness-Oneness-Fairness(Beauty) is beyond being.
- When I was not even eleven-and-a-half years old, I reasoned that since God made everything, he made existence itself and not-existence itself, so God can neither exist nor not exist, but must stand above both. Also, God cannot be a being. God is simply God. That was long before I knew anything of Plato's thoughts on the matter.
There necessarily must be some rigorous formal (i.e. mathematical) system or another that would be a perfect description of reality. — Pfhorrest
Gödel’s Incompleteness Theorems show that not even the reality of the natural numbers can be fully described by a formal theory, let alone the whole of reality.
Oh, but we can have direct hygely (noetic) knowledge of the abstract world. In fact, I’m mentally looking at the very Shape of Abstractness right now. On the other hand, I cannot directly see your thoughts or the text written in this forum.This view of the relation between the concrete and abstract bears a similarity to what Immanuel Kant called the phenomenal and the noumenal, where on his account we cannot ever have direct experiential contact with noumena, but instead only project our ideas about them behind the world of phenomena that we experience — Pfhorrest
But the abstract does have a direct bearing on the concrete. The very thoughts you’re having right now owe their being in part to the widea of thoughthood, without which they could not exist, and the very meaningfulness of the discussion of platonism against nominalism against mathematicism needs the Shape of Shapehood. See also the second-last paragraph of this comment of mine about the cynodonts.on my account the truly abstract has no direct influence on the concrete world we experience, and we can only project our ideas of abstract objects behind that concrete world in an attempt to understand and explain it. — Pfhorrest
the platonist affirms the reality of two kinds of existence — Pfhorrest
I do that, too, but I hold that all things have abstract beon, and that only information, which is another kind of beonde, can have concrete beon. Pieces of info belonging to eternally true propositions, and only those pieces of info, have both abstract and concrete being. -
 jgill
4kThe point of that thread is to illustrate specifically how, in a very distant way, we ourselves can be said to be made of empty sets. — Pfhorrest
jgill
4kThe point of that thread is to illustrate specifically how, in a very distant way, we ourselves can be said to be made of empty sets. — Pfhorrest
Some time back on another thread I mentioned being curious about the process of going from the empty set to the fundamental theorem of calculus, step by step. I had done this sort of thing sixty years ago when a professor had us reach the exponential function this way. Your proposal is much more challenging. :smile: -
 Kenosha Kid
3.2k
Kenosha Kid
3.2k
It's difficult if not impossible to conceive of a Universe that isn't mathematical. It would have to be one in which quantity was meaningless, that had no regularity, no symmetry, no fixed number of dimensions... just sheer randomness or nothingness. Obviously intelligent life could not evolve in such a universe: we can only find that mathematics describes the universe perfectly in a perfectly mathematical universe.
I like your thread on building a human, maybe even culture, from axiomatic maths, but ZFC I think is linguistic, not physical: it is the least we can insist upon to describe the most. There is, for instance, so much charge in the universe and such facts as the countability of charges is more fundamental to the mathematical nature of the universe than the fact that we can represent zero as an empty set and build the natural numbers and integers and rationals, etc., by adding small numbers of axioms as we go.
There are ambiguities in mathematics and that is likely one. There are other axiomatic maths, there may be others in future. There are also ambiguities in how we apply mathematics to physics. Is it waves or matrices? They are physically equivalent but mathematically distinct. There is also a lot of mathematics that is not useful in physics or, if useful, is so for humans to approximate or iterate solutions to physical equations and unlikely to be part of nature's toolkit. If correspondence to physical law is an argument for mathematical realism, what to make of the rest of mathematics? What of the infinity of laws we could write down but have no apparent reality? -
 Pfhorrest
4.6kIf correspondence to physical law is an argument for mathematical realism, what to make of the rest of mathematics? What of the infinity of laws we could write down but have no apparent reality? — Kenosha Kid
Pfhorrest
4.6kIf correspondence to physical law is an argument for mathematical realism, what to make of the rest of mathematics? What of the infinity of laws we could write down but have no apparent reality? — Kenosha Kid
In this view that I'm laying out in this thread, our concrete universe is just one of the infinitely many abstract mathematical structures. (We don't know for sure which; that's what physics is to investigate, which is all I have to say in response to the bulk of your post.) All of those other mathematical structures that are not this one, nor parts of this one, still "exist" abstractly on this account, but not concretely, since "exists concretely" just means "is part of the same abstract structure that we are a part of", on this account.
Maybe for illustration, imagine a nested set of simulated universes, each full of simulated people who built the next simulation down. The deepest simulation seems to be a physical world to the people in it, but is actually an informational structure embedded in what seems to be physical stuff to the people in the next level out, which in turn is actually an informational structure embedded in what seems to be physical stuff to the the people in the next level out, and so on, until we get to actual physical reality. Why cannot we just treat actual physical reality as merely the deepest of those informational structures -- and so an abstract object -- one merely not embedded in anything else? And once we've granted the existence of that one abstract object, and subsumed all concrete objects within it, why not equally grant the existence of other abstract objects of which we are not parts? -
 Kenosha Kid
3.2kAll of those other mathematical structures that are not this one, nor parts of this one, still "exist" abstractly on this account, but not concretely, since "exists concretely" just means "is part of the same abstract structure that we are a part of", on this account. — Pfhorrest
Kenosha Kid
3.2kAll of those other mathematical structures that are not this one, nor parts of this one, still "exist" abstractly on this account, but not concretely, since "exists concretely" just means "is part of the same abstract structure that we are a part of", on this account. — Pfhorrest
Fair enough, a sort of abstract multiverse (itself a theory of abstraction). But why stop at mathematical universe's? Why not consider a much larger infinity of amathematical universes we cannot comprehend, of which a subset us regular and describable, of which one is ours? Does it have to be turtles all the way down?
Maybe for illustration, imagine a nested set of simulated universes, each full of simulated people who built the next simulation down... — Pfhorrest
Simulations are an inefficient way of building universes. On a classical computer, for instance, the amount of matter required to encode the wavefunction of a single electron is many orders of magnitude larger than an electron. Future computers will do better, but the easiest way to represent any system is always going to be to build it, not to describe it. Even if we discover that, say, the holographic principle means that all information about the universe can be encoded on its 2D boundary, it would be more efficient to build a 2D surface to house a real universe than to simulate one. And this holds for any 'The stars are illusions' arguments.
I didn't have this in mind, but it's a push for me in the direction of physicalism. Mathematics is symbolic and symbolism is not as efficient as simply being. -
 Kenosha Kid
3.2kBtw obvs the OP is comparing Platonism, nominalism and mathematician, not physicalism. I agree with the OP that mathematician makes more sense. The fact that axiomatic mathematics is possible multiple ways ought to make the notion of essential triangles, say, less attractive.
Kenosha Kid
3.2kBtw obvs the OP is comparing Platonism, nominalism and mathematician, not physicalism. I agree with the OP that mathematician makes more sense. The fact that axiomatic mathematics is possible multiple ways ought to make the notion of essential triangles, say, less attractive. -
 Pfhorrest
4.6kUsually a physicalist, asked to choose between platonism and nominalism, would choose nominalism, because platonism is obviously anti-physicalist.
Pfhorrest
4.6kUsually a physicalist, asked to choose between platonism and nominalism, would choose nominalism, because platonism is obviously anti-physicalist.
OTOH I consider my kind of mathematicism to be a kind of physicalism, because it agrees that all concrete stuff is physical, inasmuch as that means empirical— the kind of stuff we can do physical sciences too — and then says that abstract stuff is the same kind of stuff as the concrete universe our physical sciences investigate, differing only in that we are not a part of those structures while we are a part of this one. To any observers in other structures, they will find them as physical as we find ours.
But why stop at mathematical universe's? Why not consider a much larger infinity of amathematical universes we cannot comprehend, of which a subset us regular and describable, of which one is ours? — Kenosha Kid
Because it’s not clear that such things are possible. See the passage from the OP:
There necessarily must be some rigorous formal (i.e. mathematical) system or another that would be a perfect description of reality. The alternative to reality being describable by a formal language would be either that some phenomenon occurs, and we are somehow unable to even speak about it; or that we can speak about it, but only in vague poetic language using words and grammar that are not well-defined. I struggle to imagine any possible phenomenon that could cause either of those problems. In fact, it seems to me that such a phenomenon is, in principle, literally unimaginable: I cannot picture in my head some definite image of something happening, yet at the same time not be able to describe it, as rigorously as I should feel like, not even by inventing new terminology if I need to. At best, I can just kind of... not really definitely imagine anything in particular. — Pfhorrest -
 Kenosha Kid
3.2kBecause it’s not clear that such things are possible. — Pfhorrest
Kenosha Kid
3.2kBecause it’s not clear that such things are possible. — Pfhorrest
Indeed. As I said myself:
It's difficult if not impossible to conceive of a Universe that isn't mathematical. — Kenosha Kid
But that only seems to me to put a tick in the physicalism box.
That said, consider a universe in which the dimensions are infinite in scope (and can be spatial, temporal or other) and random in number at any one time, the lengths of which are random at any one time, and contained fields of infinite kinds whose numbers are random at any one time and whose excitations are random at any one time. One could not describe that universe in particular mathematically; one could at best model an infinity of them stochastically.
My feeling is that no such universes exist, likely through physical constraints (the above would not conserve any quantity: information, energy, any kind of charge, any kind of momentum... everything would be discontinuous). Those physical constraints correspond to groups: mathematical objects with decorative physical constants, but there are many more such groups possible in maths than are realised in our universe.
Four possibilities:
1) This is the only possible universe: This would seem to me to give primacy to physics. Our language for describing physics is more ambiguous than physics itself, and that ambiguity is collapsed on the basis of what is physically possible. (A variation: there are many such universes and they are different, but each realises a physically possible mathematics.)
2) All possible universes are real: In a multiverse theory, all such groups might be realised in one universe or another, in which case the distinction between ideal and concrete vanishes.
3) There are infinitely many possible universes, but only this one is realised: This would seem the best fit for a mathematicism, where what is physical is contingent on what is possible, not the other way around. (A variation: there are finite many universes.)
4) Simulcra theory*: Like simulation theory, but there's no original to copy, just an infinite regression of mathematical models. There is no concrete, just the appearance of physical law arises from mathematical axioms. The question this raises now is why only this maths, given that mathematics is much more ambiguous than physics. Why should this be so limited at all?
*I coined it. It's mine. -
 Pfhorrest
4.6k2) All possible universes are real: In a multiverse theory, all such groups might be realised in one universe or another, in which case the distinction between ideal and concrete vanishes. — Kenosha Kid
Pfhorrest
4.6k2) All possible universes are real: In a multiverse theory, all such groups might be realised in one universe or another, in which case the distinction between ideal and concrete vanishes. — Kenosha Kid
This is essentially what I am proposing. But...
There is no concrete, just the appearance of physical law arises from mathematical axioms. — Kenosha Kid
This sounds like the same thing to me. There is nothing more to being a “real thing” than being an abstract possibility, except for “concreteness” which is just being a part of the same abstract possible structure as we are. -
 Kenosha Kid
3.2kThis sounds like the same thing to me. There is nothing more to being a “real thing” than being an abstract possibility, except for “concreteness” which is just being a part of the same abstract possible structure as we are. — Pfhorrest
Kenosha Kid
3.2kThis sounds like the same thing to me. There is nothing more to being a “real thing” than being an abstract possibility, except for “concreteness” which is just being a part of the same abstract possible structure as we are. — Pfhorrest
Whereas I'm saying the converse, that if all possible mathematical worlds were real, there would be nothing abstract at all. For any mathematical possibility, there would be a real instance of it. That's why I think (2) describes mathematicism less well than (3) wherein there are many possible worlds that are not realised and therefore not constrained to be real. -
 Pfhorrest
4.6kI'm having trouble following you on this. I took your mention of how "the distinction between ideal and concrete vanishes" to be talking about the same thing as I've been calling abstract and concrete, so in your scenario 2, the distinction between abstract and concrete vanishes. With that distinction gone, then "There is no concrete, just the appearance of physical law arises from mathematical axioms" seems to trivially follow, if I take that use of "concrete" there to be the usual sense that is distinct from "abstract" as used by nominalists and platonists.
Pfhorrest
4.6kI'm having trouble following you on this. I took your mention of how "the distinction between ideal and concrete vanishes" to be talking about the same thing as I've been calling abstract and concrete, so in your scenario 2, the distinction between abstract and concrete vanishes. With that distinction gone, then "There is no concrete, just the appearance of physical law arises from mathematical axioms" seems to trivially follow, if I take that use of "concrete" there to be the usual sense that is distinct from "abstract" as used by nominalists and platonists.
For an analogy, when talking about ordinary possible worlds, not necessarily mathematical-objects-as-worlds like we are, the usual modal anti-realist takes other possible worlds to be an ontologically different kind of thing than the actual world. On their account, only the actual world is real and other possible worlds are ontologically different kind of things than that actual reality. Modal realists like David Lewis, on the other hand, say there is only one kind of thing when it comes to types of worlds: possible worlds. The actual world is just one instance of that type of thing, not different or special except inasmuch as it is the one that we are in. Other possible worlds are also actual, to the people who are in them.
I'm saying basically the same thing about concrete vs abstract as Lewis says about actual vs possible. I'm not saying it's the same distinction, but the relationship between the two sides of each distinction is the same -- it's analogous:
- Lewis says the actual world is just a possible world like any other possible world, and there is no special ontological status of "actuality", just the relationship this possible world has to us, namely that we're in it. Actuality is indexical, so any possible world is actual to anyone who's in it, and merely possible to anyone else who is not.
- 'm saying, analogously, that the concrete universe is just an abstract object like any other abstract object, and there is no special ontological status of "concreteness", just the relationship this abstract object has to us, namely that we're part of it. Concreteness is indexical, so any abstract object is concrete to anyone who is part of it, and merely abstract to anyone who is not.
I drew a picture to help:
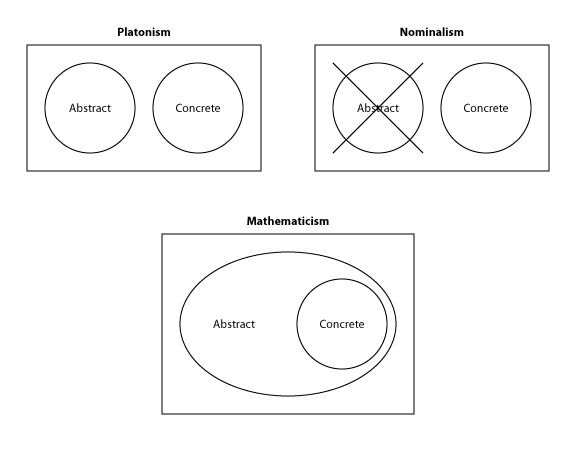
-
 Saphsin
385Can you explain what you mean by indexical and how it works that way?
Saphsin
385Can you explain what you mean by indexical and how it works that way?
I mean I looked up the word and saw your chart above, but I feel clueless about how you overcame the massive burden of proof for such a description of reality, it looks arbitrary to me. -
 Pfhorrest
4.6kIndexicals are words like “now” and “here”. There isn’t anything ontologically special about “here” compared to other places, it’s just the place where I am. Likewise, “now” is not an ontologically special time, it’s just the time where I am.
Pfhorrest
4.6kIndexicals are words like “now” and “here”. There isn’t anything ontologically special about “here” compared to other places, it’s just the place where I am. Likewise, “now” is not an ontologically special time, it’s just the time where I am.
David Lewis has proposed that “actual” is a word like that, that there’s not anything ontologically special about the actual world compared to other possible worlds, they are all ontologically the same kind of thing, equally real, and the actual world is just the one of them where we are.
I am proposing that there is nothing ontologically special about the concrete universe, that it is just the same kind of thing as any abstract object, with the only thing making it “concrete” to us being that it’s the abstract object that we are a part of.
Welcome to The Philosophy Forum!
Get involved in philosophical discussions about knowledge, truth, language, consciousness, science, politics, religion, logic and mathematics, art, history, and lots more. No ads, no clutter, and very little agreement — just fascinating conversations.
Categories
- Guest category
- Phil. Writing Challenge - June 2025
- The Lounge
- General Philosophy
- Metaphysics & Epistemology
- Philosophy of Mind
- Ethics
- Political Philosophy
- Philosophy of Art
- Logic & Philosophy of Mathematics
- Philosophy of Religion
- Philosophy of Science
- Philosophy of Language
- Interesting Stuff
- Politics and Current Affairs
- Humanities and Social Sciences
- Science and Technology
- Non-English Discussion
- German Discussion
- Spanish Discussion
- Learning Centre
- Resources
- Books and Papers
- Reading groups
- Questions
- Guest Speakers
- David Pearce
- Massimo Pigliucci
- Debates
- Debate Proposals
- Debate Discussion
- Feedback
- Article submissions
- About TPF
- Help
More Discussions
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum






