-
 TonesInDeepFreeze
3.8kas I argued with TIDF earlier in the thread. There are many ways to determine a quantity without referencing an ascending order. — Metaphysician Undercover
TonesInDeepFreeze
3.8kas I argued with TIDF earlier in the thread. There are many ways to determine a quantity without referencing an ascending order. — Metaphysician Undercover
I don't know what specifically MU has in mind that I said, but I have not said anything that could be correctly paraphrased as "There are not many ways to determine a quantity without referencing an ascending order". -
 fishfry
3.4kThanks for the clarification fishfry, but here's a couple more things still to clear up.
fishfry
3.4kThanks for the clarification fishfry, but here's a couple more things still to clear up.
To me, the following statements contradict each other.
But the set of natural number may nonetheless be ordered in many alternative ways.
— fishfry
But no set has order. That's the axiom of extensionality. Will you kindly engage with this point?
— fishfry
Which is the case, no set has order, or a set may be ordered in many different ways. Do you apprehend the contradiction? Which is it, ordered in different ways, or not ordered? — Metaphysician Undercover
The concepts are built up in layers, like a burrito.
At the bottom is the concept of set. A set is informally a collection of objects. Formally, set is an undefined term, just as point and line are undefined terms in Euclidean geometry. We all "know" what the intended meaning is, but when reasoning formally, we can only use their properties as stated by the axioms. Likewise with sets.
By the axiom of extensionality, a set is entirely characterized by its elements, without regard to order. So the set {a,b,c} is the exact same set as {b,c,a} or {c,b,a}.
That's at the lowest layer. Now we want to layer on the concept of order. To do that, we define a binary relation, which I'll call <, and we list or designate all the true pairs x < y in our set. So for example to designate the order relation a,b,c, we would take the base set {a,b,c}, and pair it with the set of ordered pairs {a < b, a < c, b < c}. Then the ordered set is designated as the PAIR ({a,b,c,}, {a < b, a < c, b < c}). I hope this is clear.
For example we have the unordered set consisting of all the natural numbers 0, 1, 2, ... Then we layer on top of it the usual order <, so that the ordered sets is now . In other words a set is an unordered collection. An ordered set is a PAIR consisting of an unordered set, along with an order relation.
In the case of the usual order relation on the natural numbers, the order relation < is actually the set of all the true order statements: {0 < 1, 0 < 2, 0 < 3, ..., 1 < 2, 1 < 3, 1 < 4, ..., 2 < 3, 2 < 4, ...}.
In order to remove the apparent ambiguity of using the symbol < as both the relation and as specific instances of it, formally a relation is a set of ordered pairs; so the usual < relation on the natural numbers is actually the set {(0,1), (0,2), (0, 3), ..., (1,2), (1,3), ...}. Again I hope this is clear, it's basic stuff for a math major but is definitely a little formalism-heavy if you haven't seen it before.
The basic takeaway is that a set has no inherent order. We impose an order on a set by PAIRING the set with an order relation. That's why earlier I noted that we can start with the set and then form two distinct ordered sets and , where and are distinct order relations.
I'll mention in passing that this is a very common pattern in math. We start with a set , which has no inherent structure at all. Then we let be a topology on , and we call the pair a topological space. Or we have an unstructured set and pair it with an operation , subject to some rules for how behaves, and we call the pair an Abelian group.
Pretty much everything in math is defined as some set, along with some other structures that impose whatever attributes on the set that we're interested in.
Finally, there's always some notational ambiguity, because when we say, for example, , we very often mean the set of natural numbers along with its usual order. The meaning is always clear from context. If we were being precise we would always write for the set with its usual order; and we would write for the natural numbers with their usual order and standard arithmetic operations of addition and multiplication.
You don't have to care about the details. What is important is that any "structured set" actually consists, formally, of an unstructured set combined with whatever additional structure we care about: an order relation, a topology, arithmetic operations, and the like. I truly hope this is clear, and if not please ask.
Let me go back to my question from the last post. What exactly constitutes "the set"? Is it the description, or is it the elements which are the members of the set. — Metaphysician Undercover
Of course the description is just a representation, as 5 is a representation of the abstract number 5 (whatever that is!) and "snow" is a representation of snow.
A set is entirely characterized by its elements; but a set is more than just its elements. It's the elements along with the collecting of the objects into a set. Maybe that's a bit philosophical, I'm not sure if I can really explain it any better than that. A set is a collection of elements, regarded as an individual thing, a set. So in Peano arithmetic we have numbers 0, 1, 2, 3, 4, ... But the axiom of infinity is much stronger. It says that there is a SET that contains all the numbers. It's the difference between 0, 1, 2, 3, ... and {0, 1, 2, 3, ...}. I hope that's clear, but if you think there's a lot of philosophical mystery that I haven't adequately explained, I'd be inclined to agree. Perhaps it's the distinction between a bunch of athletes and a team, or a collection of birds and a flock. I'm sure some philosophers have found ways to describe this. A set is a collection of elements, along with the concept of their set-hood. That's the best I can do!
If it is the description, or definition, then order is excluded by the definition. — Metaphysician Undercover
Agreed. The order is imposed by PAIRING the set with a separate order relation. Hope I made that clear in my longwinded exposition a moment ago.
But if the set is the actual participants, then as I explained already they cannot exist without having an order. If the supposed participants have no existence then they cannot constitute the set. — Metaphysician Undercover
The order relation is technically a separate set, consisting of the collection of ordered pairs that define the order. Hope I made that clear already.
That's why I ask, which is it? Can a set be ordered, or is it inherently without order? Surely it cannot be both. — Metaphysician Undercover
A set is inherently without order and without any kind of structure. We impose order and other structures (topology, arithmetic, etc.) on a set by pairing the set with additional relations, which are themselves formally defined as other sets.
And as I've mentioned, we often CASUALLY say "the ordered set X," or "the topological space X", when we REALLY mean the pair (X, <) or the pair (X, . That's perhaps the source of some confusion, as I can sometimes mean X the unstructured set, or X the ordered set, or X the topological space, because I'm implicitly leaving out the addition structure with which X is paired.
But formally, every set is inherently unstructured and unordered. We impose structure, order, arithmetic operations, etc., after the fact, by associating the set with other sets that represent order relations, topologies, arithmetic operations, and so forth.
Let's look at "concept" as a noun, as if a concept is a thing. Do you agree that a concept is the product, or result of conception, which is a mental activity? There's different mental activity involved, understanding, judgement, conclusion, and effort to remember. Would you agree that the effort to remember is what maintains the concept as a static thing, So if a "concept" is used as a noun, and is said to be a thing, it is in the same sense that a memory is said to be a thing. Would you agree that if a mathematical concept is "a thing", it is a thing in the same sense that a memory is a thing? — Metaphysician Undercover
You lost me a bit here. The original question is that you object to my use of the phrase mathematical object, and I'm just asking what to call it instead. If you want me to call numbers, topological spaces, groups, rings, and fields "mathematical concepts" instead of mathematical objects, I'll do that if it makes you happy, but really, they're mathematical objects and universally recognized as such by people trained in math. -
 Metaphysician Undercover
14.3kYou assume that numbers are objects but argue that numbers are not objects? Sounds about right given your confusion. — Luke
Metaphysician Undercover
14.3kYou assume that numbers are objects but argue that numbers are not objects? Sounds about right given your confusion. — Luke
Do you understand the meaning of the word "if"? I don't think it's me who's the confused one.
By the axiom of extensionality, a set is entirely characterized by its elements, without regard to order. So the set {a,b,c} is the exact same set as {b,c,a} or {c,b,a}. — fishfry
That "elements" may exist without an order is the falsity I've explained to you already. And if we say that "element" indicates an abstraction, then it is a universal, not a particular, and to assume that an abstraction is a particular is a category mistake.
Let's assume a special type of "element", created, or imagined specifically for set theory. This type of element can exist in a multitude without that multitude having any order. Each of these elements would have no spatial or temporal relation to any other element, or else there would be an order, according to that relation. We could say that they are like points, but without a spatial reference, so that we cannot draw lines between them etc., because there is no order to them. But if they were like points, without spatial relations constituting order, there would be no way to distinguish one from another
Unlike points though, there is something which distinguishes one element from another, so that in the set of (a,b,c,), "a" does not represent the same thing as "b" does. Can I conclude, that the distinct elements are separated from one another, and distinguished one from another, by something other than space? To make them distinct and individual, they must have separation, but the separation cannot be spatial or else they would have an order, by that spatial relation.
Do you see, that from this premise alone, we cannot give any order to any set? To give a set an order would be a violation of the fundamental meaning of "element" which allows that elements can exist as particulars without any spatial temporal; relations. To be able to talk about an order within a set, would require that we transform the elements into something other than "elements", something which could have spatial or temporal relations and therefore an order. Remember, even quantity requires spatial-temporal separation between one and the other, to distinguish separate individuals.
Now we want to layer on the concept of order. To do that, we define a binary relation, which I'll call <, and we list or designate all the true pairs x < y in our set. So for example to designate the order relation a,b,c, we would take the base set {a,b,c}, and pair it with the set of ordered pairs {a < b, a < c, b < c}. Then the ordered set is designated as the PAIR ({a,b,c,}, {a < b, a < c, b < c}). I hope this is clear. — fishfry
No, sorry, it's not clear at all. You have imagined distinct "elements" which exist without any spatial or temporal relations, thereby having no order, though they are somehow distinct individuals. Now you want to add order. You have already defined order out of the set, to add it in, is blatant contradiction.
The basic takeaway is that a set has no inherent order. We impose an order on a set by PAIRING the set with an order relation. — fishfry
What I need, is a clear explanation of what an "order relation" is. What type of relation are you attempting to give to these elements, which gives them an order, when you've already stipulated the premise that they have no order?
The point is, that to give them existence without order requires a special conceptualization which I described above. Now if we want to proceed with that conceptualization, and now bring in principles of order, we must do so in a consistent way. So, we need to describe what separates one element from another, since it's clearly not space, and what makes it distinct as an element, in terms which do not give it a relationship to the others, to allow that the multitude of them do not already have any order, Then we need a principle by which order can be initiated within this non-ordered type of separation.
A set is a collection of elements, regarded as an individual thing, a set. — fishfry
Clearly, for a group of things to be regarded as an individual thing, "unity" is implied. And, it is quite clear that for a group of parts to form a unity, it is necessary that the parts exist with some sort of order. So this statement directly contradicts you assertion that a set has no inherent order.
Perhaps it's the distinction between a bunch of athletes and a team, or a collection of birds and a flock. I'm sure some philosophers have found ways to describe this. A set is a collection of elements, along with the concept of their set-hood. That's the best I can do! — fishfry
Do you see the contradiction? You describe a "set" as a thing, a unity, like a team, but then you say that there is no inherent order to this unified thing. Do you see how ridiculous this is, to say that there exists a unified thing, composed of parts, but there is no order to the parts? How in the world are we supposed to conceive of a unity of parts which have no order? To say that they are a unity is to say that they have order.
A set is inherently without order and without any kind of structure. — fishfry
Take a look again. You are proposing a type of unity, a "set", without any structure. By what principle do you say that it is a collection?
A set is entirely characterized by its elements; but a set is more than just its elements. It's the elements along with the collecting of the objects into a set. — fishfry
What is this act which you call "the collecting of the objects into a set"? Wouldn't such an act necessarily create an order, if only just a temporal order according to which ones are collected first? I'm trying to figure out how you get around the need for the elements to have an order. I mean, it's one thing to assert, "I've got this collection of elements and they have no inherent order" (to which I'd say you're lying), and another thing to demonstrate how you've collected a group of elements into a unified whole, without them having any order. -
 Luke
2.7kDo you understand the meaning of the word "if"? — Metaphysician Undercover
Luke
2.7kDo you understand the meaning of the word "if"? — Metaphysician Undercover
As in, if you were arguing that numbers are not objects? But you already told us that you were. You also told us that you assume numbers are objects.
For someone so rabid about logic, you seem quite content with your contradiction. -
 TonesInDeepFreeze
3.8kFor sets with cardinality greater than 1:
TonesInDeepFreeze
3.8kFor sets with cardinality greater than 1:
It's not that sets don't have orderings. It's that sets have many orderings (though in some cases we need a choice axiom or an axiom weaker than choice but still implying linear ordering). So the point is that there is no single ordering that is "the ordering".
/
Typically, in an informal sense, the notion of 'set' is taken as undefined. But just a technical note: In set theory and in class theory, we can formally define 'set' from the primitive 'element'. -
 Metaphysician Undercover
14.3kYou also told us that you assume numbers are objects. — Luke
Metaphysician Undercover
14.3kYou also told us that you assume numbers are objects. — Luke
Notice, the quoted passage says numbers are assumed when "you" count. And, it's your count that I argue is false. .
You are back to your pathetic strawman misinterpretation, for the sake of ridicule.
It's not that sets don't have orderings. It's that sets have many orderings (though in some cases we need a choice axiom or an axiom weaker than choice but still implying linear ordering). So the point is that there is no single ordering that is "the ordering". — TonesInDeepFreeze
If we assume that a set necessarily has an ordering, but it could be one of many possible orderings, by what principle can we say that each of these many possible orderings constitutes the same set? What type of entity is an "element", such that the identity of a unity of numerous elements is based solely in the identity of its parts with complete disregard for the relations between those parts? Isn't this a sort of fallacy of composition? -
 Luke
2.7kNotice, the quoted passage says numbers are assumed when "you" count. And, it's your count that I argue is false. . — Metaphysician Undercover
Luke
2.7kNotice, the quoted passage says numbers are assumed when "you" count. And, it's your count that I argue is false. . — Metaphysician Undercover
I'm sure you meant the impersonal pronoun. This is more obvious with the preceding sentence provided for context:
Your "ascending order" is based on quantity, therefore your supposed "count" of ascending order means nothing unless it is determining a quantity. This is why "numbers" as objects are assumed, so that when you count up to ten you have counted ten objects, (numbers). — Metaphysician Undercover
You assert your stipulation/argument that "ascending order" is based on quantity, and then "This is why "numbers" as objects are assumed" by you. It's your argument and your assumption.
Otherwise, if you were arguing that "my" count is false, then it would also be false that "when you count up to ten you have counted ten objects, (numbers)." This would be odd, since it's the antithesis of your argument in earlier posts. But you are no stranger to contradiction, since you said in the very same post:
And, if numbers are not true objects, as I argue is the case, then this is not a true act of counting at all. — Metaphysician Undercover
Keep blowing smoke trying to hide your contradiction. You clearly cannot account for it. -
 TonesInDeepFreeze
3.8kIf we assume that a set necessarily has an ordering — Metaphysician Undercover
TonesInDeepFreeze
3.8kIf we assume that a set necessarily has an ordering — Metaphysician Undercover
We prove from axioms.
by what principle can we say that each of these many possible orderings constitutes the same set? — Metaphysician Undercover
"constitutes" is your word.
What type of entity is an "element", such that the identity of a unity of numerous elements is based solely in the identity of its parts with complete disregard for the relations between those parts? — Metaphysician Undercover
An element is an x such that there exists a y such that xey. In set theory, every x is an element of some y.
Isn't this a sort of fallacy of composition? — Metaphysician Undercover
No.
Your questions reflect your complete ignorance of set theory. You could remedy your ignorance by getting a book and reading it. -
 Metaphysician Undercover
14.3kWe prove from axioms. — TonesInDeepFreeze
Metaphysician Undercover
14.3kWe prove from axioms. — TonesInDeepFreeze
If an axiom is false then the proof is unsound. -
 jgill
4kIf an axiom is false then the proof is unsound — Metaphysician Undercover
jgill
4kIf an axiom is false then the proof is unsound — Metaphysician Undercover
Is it possible for an axiom to be false? Please explain. Don't refer to inconsistency. :roll: -
 TonesInDeepFreeze
3.8kIf an axiom is false then the proof is unsound. — Metaphysician Undercover
TonesInDeepFreeze
3.8kIf an axiom is false then the proof is unsound. — Metaphysician Undercover
Which axioms of finite set theory do you think are false?
Sorry, that was a stupid question. You don't know any axioms. -
 fishfry
3.4kThat "elements" may exist without an order is the falsity I've explained to you already. And if we say that "element" indicates an abstraction, then it is a universal, not a particular, and to assume that an abstraction is a particular is a category mistake. — Metaphysician Undercover
fishfry
3.4kThat "elements" may exist without an order is the falsity I've explained to you already. And if we say that "element" indicates an abstraction, then it is a universal, not a particular, and to assume that an abstraction is a particular is a category mistake. — Metaphysician Undercover
I think you are just not cut out for mathematical abstraction and should pick another major.
Let's assume a special type of "element", created, or imagined specifically for set theory. This type of element can exist in a multitude without that multitude having any order. Each of these elements would have no spatial or temporal relation to any other element, or else there would be an order, according to that relation. We could say that they are like points, but without a spatial reference, so that we cannot draw lines between them etc., because there is no order to them. But if they were like points, without spatial relations constituting order, there would be no way to distinguish one from another — Metaphysician Undercover
Ditto.
Unlike points though, there is something which distinguishes one element from another, so that in the set of (a,b,c,), "a" does not represent the same thing as "b" does. Can I conclude, that the distinct elements are separated from one another, and distinguished one from another, by something other than space? To make them distinct and individual, they must have separation, but the separation cannot be spatial or else they would have an order, by that spatial relation.
Do you see, that from this premise alone, we cannot give any order to any set? To give a set an order would be a violation of the fundamental meaning of "element" which allows that elements can exist as particulars without any spatial temporal; relations. To be able to talk about an order within a set, would require that we transform the elements into something other than "elements", something which could have spatial or temporal relations and therefore an order. Remember, even quantity requires spatial-temporal separation between one and the other, to distinguish separate individuals. — Metaphysician Undercover
Ditto. I'm not going to bother. You are obfuscatory in the extreme. The only thing I can't figure out is why someone with zero aptitude for mathematical abstraction is so interested in it, yet so utterly unwilling to engage with it.
No, sorry, it's not clear at all. You have imagined distinct "elements" which exist without any spatial or temporal relations, thereby having no order, though they are somehow distinct individuals. — Metaphysician Undercover
Yes exactly.
Now you want to add order. You have already defined order out of the set, to add it in, is blatant contradiction. — Metaphysician Undercover
Enough. You win. You wore me out.
What I need, is a clear explanation of what an "order relation" is. — Metaphysician Undercover
I have explained this many times. I linked you to the Wiki page on mathematical order theory. An order is a binary relation; that is, a function that outputs True or False for every pair of elements in a set; that has certain properties as I've mentioned several times already.
Here is the page. Come back when you've made a sincere effort to understand the material.
https://en.wikipedia.org/wiki/Order_theory
What type of relation are you attempting to give to these elements, which gives them an order, when you've already stipulated the premise that they have no order? — Metaphysician Undercover
I explained that it's a process of abstraction, where we start from no assumptions and layer on the structure we want. If you don't get it, you don't get it.
The point is, that to give them existence without order requires a special conceptualization which I described above. Now if we want to proceed with that conceptualization, and now bring in principles of order, we must do so in a consistent way. So, we need to describe what separates one element from another, since it's clearly not space, and what makes it distinct as an element, in terms which do not give it a relationship to the others, to allow that the multitude of them do not already have any order, Then we need a principle by which order can be initiated within this non-ordered type of separation. — Metaphysician Undercover
Pick another major.
Skipping the rest. I've done what I can. I do recommend that you read the page on order theory.
I do get that you reject the mathematical concept of set. Not much anyone can do about that. It's like saying you want to learn physics and then arguing with the concepts of space, time, force, motion, energy, and temperature. You may well have a philosophical point to make, but you are preventing yourself from learning the subject. And it's learning the subject that would allow you to make more substantive rather than naive and obfuscatory objections.
I have explained that set is an undefined term entirely characterized by its behavior under the axioms. You insist on imposing your own incorrect conceptions. So there's no conversation to be had. -
TheMadFool
13.8kGiven that physicists rely heavily on math and knowing that the current foundation of math is Set Theory and taking into consideration The Axiom Of Infinity, it becomes impossible for ALL physicists not to believe in an actual infinity, the set of Natural numbers {1, 2, 3,...}. -
 Metaphysician Undercover
14.3kIs it possible for an axiom to be false? Please explain. Don't refer to inconsistency. :roll: — jgill
Metaphysician Undercover
14.3kIs it possible for an axiom to be false? Please explain. Don't refer to inconsistency. :roll: — jgill
For sure it's possible, the difficulty would be to demonstrate falsity, and this would require reference to some sort of inconsistency. What else could demonstrate falsity other than a reference to some form of inconsistency?.
An axiom is expressed as a bunch of symbols, so it must be interpreted. Interpretation requires that it be related to something else, and here we can have inconsistency and contradiction. So the author of an axiom will intentionally avoid internal inconsistency, or contradiction, but to understand, or employ the axiom it must be related to something external to it. If in interpretation, there is a contradiction with another principle then one or both must be false. If the other is a principle one holds to be true, then the axiom must be viewed as false.
Take the axiom of extensionality for example. Here's how Wikipedia states it:
".Given any set A and any set B, if for every set X, X is a member of A if and only if X is a member of B, then A is equal to B.
(It is not really essential that X here be a set — but in ZF, everything is. See Ur-elements below for when this is violated.)"
Further, Wikipedia says it is interpreted like this:
" To understand this axiom, note that the clause in parentheses in the symbolic statement above simply states that A and B have precisely the same members. Thus, what the axiom is really saying is that two sets are equal if and only if they have precisely the same members."
Notice there is an exchange of "equal" and "same". As I've argued in other threads, if we adhere to the law of identity, this is a false use of "same". To resolve this issue, one might deny the law of identity, or insist on a faulty interpretation of that law. I think that approach is futile, so we must look directly at the axiom of extensionality and see what "equal" means in that context. If we can interpret in a way which does not employ "same" we might avoid the falsity.
Which axioms of finite set theory do you think are false? — TonesInDeepFreeze
Read above.
I think you are just not cut out for mathematical abstraction and should pick another major. — fishfry
I found that out at about tenth grade, despite living in an extremely mathematically inclined family. Prior to that though, I had difficulty even in grade school, when the teachers insisted on distinguishing numbers from numerals. Where are these "numbers" that the teacher kept trying to tell us about, I thought. All I could see is the numerals, and the quantity of objects referred to by the numeral. But the teacher insisted no, the numeral is not the number. So it took me very long to figure out that the numeral was not the "number" which the teacher was talking about, and that the number was just some fictitious thing existing in the teacher's mind, so I shouldn't even bother looking for it because I have to make up that fiction in my own mind, for there to be a number for me to "see". Of course, I chose philosophy as a major instead, because philosophy provides a solid, grounded understanding of abstraction, rather than simply insisting on the existence of fictitious "numbers" existing in people's minds, and trying to convince people to create those fictitious things in their minds.
Enough. You win. You wore me out. — fishfry
Simply put, I'm right and you're wrong. Nah, nah, let's go back to grade school. You should have chosen philosophy instead of math, if you wanted to learn the truth about abstraction.
Pick another major. — fishfry
I have picked another major, philosophy. That's why I'm discussing this in a philosophy forum. Care to join me? Or will you simply assert that mathematics is far superior to philosophy, then run off and hide under some numbers somewhere when the unintelligibility of your principles is demonstrated to you?
You may well have a philosophical point to make, but you are preventing yourself from learning the subject. And it's learning the subject that would allow you to make more substantive rather than naive and obfuscatory objections. — fishfry
You seem to have a very naive outlook. How do you propose that one proceed toward "learning the subject", when the most basic principles in that subject do not make any sense to the person? To me, the distinction between a numeral and a number is fundamentally unintelligible, as a falsity, because it requires producing a fictitious thing in my mind, and then talking about that fictitious thing as if it is a truth. Therefore proceeding into "learning the subject" requires an initial step of dishonesty, self-deception, then deceiving others in talking about this issue I have deceived myself about. I am not prepared to make that step of dishonesty. Making that initial step of self-deception is the first step toward misunderstanding, not toward understanding. -
 TonesInDeepFreeze
3.8kWhat else could demonstrate falsity other than a reference to some form of inconsistency?. — Metaphysician Undercover
TonesInDeepFreeze
3.8kWhat else could demonstrate falsity other than a reference to some form of inconsistency?. — Metaphysician Undercover
Falsity is semantic; inconsistency is syntactical.
Given a model M of a theory T, a sentence may be false in M but not inconsistent with T.
An axiom is expressed as a bunch of symbols, so it must be interpreted. — Metaphysician Undercover
Formulas don't have to be interpreted, though usually they are when they are substantively motivated.
If in interpretation, there is a contradiction with another principle then one or both must be false. — Metaphysician Undercover
It might not be a matter of principles but of framework. Frameworks don't have to be evaluated as true or false, but may be regarded by their uselfulness in providing a conceptual context or their productivity in other ways.
Notice there is an exchange of "equal" and "same" — Metaphysician Undercover
Even though there is nothing wrong with taking 'equal' to mean 'same', the axiom of extensionality doesn't require such mention.
Az(zex <-> zey) -> x=y.
"=' is mentioned, but not "same".
As I've argued in other threads, if we adhere to the law of identity, this is a false use of "same". — Metaphysician Undercover
As you argued unsuccessfully, ignorantly and incoherently.
I had difficulty even in grade school — Metaphysician Undercover
The education system let you down. They should have given you proper cognitve tests to investigate your learning disability.
will you simply assert that mathematics is far superior to philosophy — Metaphysician Undercover
We don't have to assert such a thing. But understanding mathematics is prerquisite to philosophizing about it.
How do you propose that one proceed toward "learning the subject", when the most basic principles in that subject do not make any sense to the person? — Metaphysician Undercover
By the person at least reading the first chapters in a textbook on the subject. If the person cannot comprehend those first basics, then we might have to admit that the person is simply ineducable. -
 fishfry
3.4kYou seem to have a very naive outlook. How do you propose that one proceed toward "learning the subject", when the most basic principles in that subject do not make any sense to the person? — Metaphysician Undercover
fishfry
3.4kYou seem to have a very naive outlook. How do you propose that one proceed toward "learning the subject", when the most basic principles in that subject do not make any sense to the person? — Metaphysician Undercover
Well in fact that is exactly how one learns math! Once you get past calculus, the early upper division math classes make no sense to students. Abstract algebra and real analysis typically baffle students, until at some point they either get it or give up. It's like saying that learning to play a musical instrument is tremendously difficult at first so people should just give up. On the contrary, you do your scales over and over and over and one day you find that you can play music passably well. It's called learning.
It's true of virtually EVERYTHING that at first, the subject makes no sense. You just do as you're told, do the exercises, do the homework, do the problem sets without comprehension, till one day you wake up and realize you've learned something. It must be that you've learned nothing at all in your life, having given up the moment something doesn't make immediate sense to you.
If you truly wish to criticize the foundations of math, wouldn't it make sense for you to temporarily put aside your objections, and learn the material on its own terms? Especially as you've found someone willing to explain it to you. Then after you have grasped the basic methodologies, such as abstraction and layering properties on top of formless sets -- THEN you are in a better position to make substantive criticisms rather than childish ones.
When you learned to play chess, or any game -- bridge, poker, whist -- do you say, "Oh this is nonsense, no knight REALLY moves this way," and quit? Why can't you learn a formal game on its own terms? If for no other reason than to be able to criticize it from a base of knowledge rather than ignorance? If you've never seen a baseball game, it makes no sense. As you watch, especially if you are lucky enough to have a companion who is willing to teach you the fine points of the game, you develop appreciation. Is that not the human activity called LEARNING? Why are you morally opposed to it?
Finally, even your basic objection to unordered sets is wrong. Imagine a bunch (infinitely many, even) of points randomly distributed on the plane or in 3-space. Can't you see that there is no inherent order? Then you come by and say, "Order them left to right, top to bottom." Or, "Order them by distance from the origin, and break ties by flipping a coin." Or, "Call this one 1, call this one 2, etc."
Where is the inherent order in an otherwise random assemblage of points?
I had difficulty even in grade school, when the teachers insisted on distinguishing numbers from numerals. Where are these "numbers" that the teacher kept trying to tell us about, I thought. All I could see is the numerals, and the quantity of objects referred to by the numeral. But the teacher insisted no, the numeral is not the number. So it took me very long to figure out that the numeral was not the "number" which the teacher was talking about, and that the number was just some fictitious thing existing in the teacher's mind, so I shouldn't even bother looking for it because I have to make up that fiction in my own mind, for there to be a number for me to "see". — Metaphysician Undercover
I feel for you. LOL. But you see, you WERE capable of getting it. Or you could just take the formalist perspective and say that the entire thing is a fictional game made up of marks on paper. In which case you couldn't object to math any more than you can object to chess.
To me, the distinction between a numeral and a number is fundamentally unintelligible, as a falsity, because it requires producing a fictitious thing in my mind, and then talking about that fictitious thing as if it is a truth. — Metaphysician Undercover
So just adopt the formalist perspective. There are only numerals and the rules for manipulating them. It's a game. What on earth is your objection? Were you like this when you learned to play chess? "There is no knight!" "The Queen has her hands full with Harry and that witch Meghan!" etc. Surely you're not like this all the time, are you?
Or will you simply assert that mathematics is far superior to philosophy, — Metaphysician Undercover
If you can find any instance of my ever asserting such a thing on this forum, then show it. Otherwise you have lied, claiming I said something I never said nor implied in any way.
then run off and hide under some numbers somewhere when the unintelligibility of your principles is demonstrated to you? — Metaphysician Undercover
On the contrary. I'm doing you the service of attempting to explain to you how modern abstract mathematics is set up. And I'm giving up, since it's clear that you'd rather cling to your naive and incorrect beliefs about the subject rather than learn anything. -
 fishfry
3.4k@Metaphysician Undercover, What is the inherent order of the points in this set? Can you see that the points are inherently disordered or unordered, and that we may impose order on them arbitrarily in many different ways? Pick one and call it the first. Pick another and call it the second. Etc. What's wrong with that?
fishfry
3.4k@Metaphysician Undercover, What is the inherent order of the points in this set? Can you see that the points are inherently disordered or unordered, and that we may impose order on them arbitrarily in many different ways? Pick one and call it the first. Pick another and call it the second. Etc. What's wrong with that?
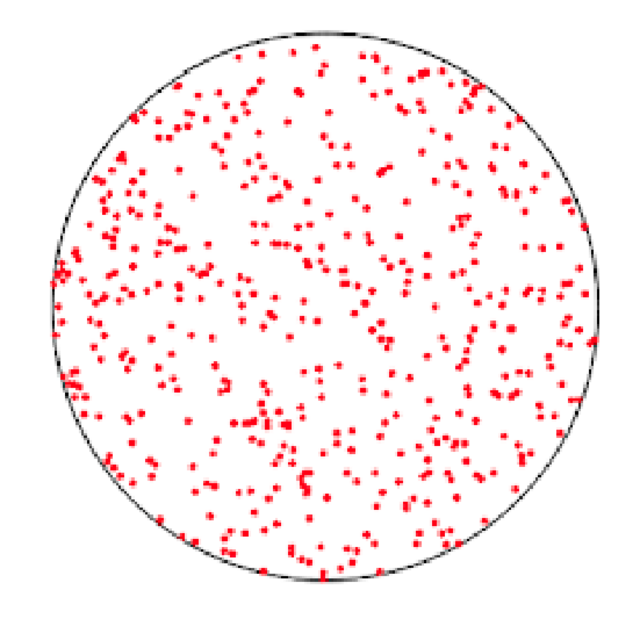
-
 TonesInDeepFreeze
3.8kat first, the subject makes no sense — fishfry
TonesInDeepFreeze
3.8kat first, the subject makes no sense — fishfry
That the subject at first makes little sense is probably usually true. And it's true for me for many different subjects. But symbolic logic is one subject that made perfect sense to me immediately. Then, after learning the predicate calculus I found that there is a mathematical analysis of it and theorems not just in the predicate calculus but about the predicate calculus. I was blown away with admiration of the human intelligence that would devise such a calculus but also move on to prove its consistency and completeness.
But when I was a child, I was not interested in having to learn arithmetic by rote stipulations. But I was intrigued by some of the other math I was introduced to when I was 10 years old, including sets, Venn diagrams, base number systems and things like that. Those ideas - the abstraction involved - immediately impressed me as like pure cool water of abstract invention. That's what I most admire about mathematics - that it combines full rigor with free exploration of abstract imagination. Later, I was not interested in high school algebra - again just a bunch of stipulations. But in the back of my mind, I wondered what kinds of problems have algorithms (though I didn't know the word 'algrorithm' then) for solving, even if only in principle. Again, years later when I discovered mathematical logic, I found that this question had been investigated thoroughly, and is still being investigated.
Mathematics is not at all one of my intellectual strengths; I'm really not very good at it. But I love it.
The other area that I understood immediately is jazz. The very first time I happend to put on a jazz record to give it real attention, I loved it, understand it, and embraced it for a lifetime. -
 fishfry
3.4k
fishfry
3.4k -
 Metaphysician Undercover
14.3k
Metaphysician Undercover
14.3k
Sorry Tones, but we're so far apart on these principles of truth and falsity, that I see no place to start, or any point to it. I look at truth as corresponding with reality.
It's like saying that learning to play a musical instrument is tremendously difficult at first so people should just give up. — fishfry
I don't think the analogy is good. I learned to play a musical instrument, and it always made sense to me, right from the start. I learned philosophy and it always made sense to me. The point is that I proceeded because it made sense to. If mathematics requires self-deception, then this does not make sense to me, and so I will not proceed. Many people would not become athletes because there is much physical pain involved in the practice. This might be similar to my refusal to learn math because physical pain, and self-deception may both be viewed as harmful. Some people though will put up with the physical pain because they see a greater good in being an athlete. Maybe you put up with the self-deception of mathematics because you apprehend a greater good.
It's true of virtually EVERYTHING that at first, the subject makes no sense. You just do as you're told, do the exercises, do the homework, do the problem sets without comprehension, till one day you wake up and realize you've learned something. It must be that you've learned nothing at all in your life, having given up the moment something doesn't make immediate sense to you. — fishfry
Again I don't agree with this. Many things I've learned made sense to me right from the start. Even learning the numerals, how to count, and simple arithmetic, addition, subtraction multiplication, division made sense to me right from the start. It was only later, when they started insisting that there existed a number, distinct from the numeral, that things started not making sense.
I had a similar experience later with physics. We learned basic physics, then we learned about waves, and got to experiment in wave tanks. We learned that waves were an activity within a medium and we were shown through diagrams how the particles of the medium moved to formulate such an activity. All of this made very much sense to me. Then we were shown empirical proof that light existed as waves, and we were told that light waves had no medium. Of course this made no sense to me.
When you learned to play chess, or any game -- bridge, poker, whist -- do you say, "Oh this is nonsense, no knight REALLY moves this way," and quit? Why can't you learn a formal game on its own terms? If for no other reason than to be able to criticize it from a base of knowledge rather than ignorance? If you've never seen a baseball game, it makes no sense. As you watch, especially if you are lucky enough to have a companion who is willing to teach you the fine points of the game, you develop appreciation. Is that not the human activity called LEARNING? Why are you morally opposed to it? — fishfry
When I learn a game, I must learn the rules before I play. If the rules are such that I have no desire to play the game, I do not play. It's not a question of whether the game makes sense or not, so the analogy is not a good one.
Finally, even your basic objection to unordered sets is wrong. Imagine a bunch (infinitely many, even) of points randomly distributed on the plane or in 3-space. Can't you see that there is no inherent order? Then you come by and say, "Order them left to right, top to bottom." Or, "Order them by distance from the origin, and break ties by flipping a coin." Or, "Call this one 1, call this one 2, etc." — fishfry
Finally, you decided to address the issue. If there are points distributed on a plane, or 3d space, the positioning of those points relative to each other is describable, therefore there is an inherent order to them. If there was no order their positioning relative to each other could not be described..
You say that they are "randomly distributed", to create the illusion that there is no order. But the fact is that they must have been distributed by some activity, and their positioning posterior to that activity is a reflection of that activity, therefore their positioning is necessarily ordered, by that activity.
So just adopt the formalist perspective. There are only numerals and the rules for manipulating them. It's a game. What on earth is your objection? Were you like this when you learned to play chess? "There is no knight!" "The Queen has her hands full with Harry and that witch Meghan!" etc. Surely you're not like this all the time, are you? — fishfry
If you think you can interpret the rules as we go, then I'd advise you not to play any games with me.
What is the inherent order of the points in this set? Can you see that the points are inherently disordered or unordered, and that we may impose order on them arbitrarily in many different ways? Pick one and call it the first. Pick another and call it the second. Etc. What's wrong with that? — fishfry
The inherent order is the exact spatial positioning shown in the diagram. If any point changes location, then the order is broken. Is that so difficult to understand? A spatial ordering is not a matter of first and second, that is a temporal ordering. -
 Metaphysician Undercover
14.3k
Metaphysician Undercover
14.3k
That about sums it up. Math is like religion, a whole bunch of bullshit which we are told to accept on faith. -
 TonesInDeepFreeze
3.8kI look at truth as corresponding with reality. — Metaphysician Undercover
TonesInDeepFreeze
3.8kI look at truth as corresponding with reality. — Metaphysician Undercover
Whatever your personal meaning of "reality", or lack of meaning, might be.
Meanwhile, you're not even familiar with the distinction between semantics and syntax and the notion of model theoretic truth. So you don't know anything about the mathematics you disdain.
If mathematics requires self-deception — Metaphysician Undercover
It doesn't.
how to count, and simple arithmetic, addition, subtraction multiplication, division made sense to me right from the start. It was only later, when they started insisting that there existed a number, distinct from the numeral, that things started not making sense. — Metaphysician Undercover
Exactly, you could perform calculations by rote adherence, but as soon as you confronted actual abstract thought, you couldn't handle it and dealt with it by reviling it.
If you think you can interpret the rules as we go — Metaphysician Undercover
He didn't suggest anything like that. You're back to one of your favorite tactics again: the strawman argument.
The inherent order is the exact spatial positioning shown in the diagram. — Metaphysician Undercover
No one but you uses the word "order' that way. But it does allow you to evade the challenge in the example. -
 fishfry
3.4kThat about sums it up. Math is like religion, a whole bunch of bullshit which we are told to accept on faith — Metaphysician Undercover
fishfry
3.4kThat about sums it up. Math is like religion, a whole bunch of bullshit which we are told to accept on faith — Metaphysician Undercover
I was thinking you'd respond to that with a little self-aware sense of humor.
I found that out at about tenth grade, despite living in an extremely mathematically inclined family. — Metaphysician Undercover
Could there be some unresolved psychological dynamics at play?
In any event, can you please respond to my point about chess? Surely if you learned to play chess, or any other artificial game -- monopoly, bridge, checkers, baseball -- you were willing to simply accept the rules as given, without objecting that they don't have proper referents in the real world or that they make unwarranted philosophical assumptions. If you could see math that way, even temporarily, for sake of discussion, you might learn a little about it. And then your criticisms would have more punch, because they'd be based on knowledge. I wonder if you can respond to this point. Why can't you just treat math like chess? Take it on its own terms and shelve your philosophical objections in favor of the pleasure of the game. -
 TonesInDeepFreeze
3.8kMath is like religion — Metaphysician Undercover
TonesInDeepFreeze
3.8kMath is like religion — Metaphysician Undercover
Mathematics is the opposite of religion. In another post I completely demolished the comparison. -
 fishfry
3.4kAgain I don't agree with this. Many things I've learned made sense to me right from the start. Even learning the numerals, how to count, and simple arithmetic, addition, subtraction multiplication, division made sense to me right from the start. It was only later, when they started insisting that there existed a number, distinct from the numeral, that things started not making sense. — Metaphysician Undercover
fishfry
3.4kAgain I don't agree with this. Many things I've learned made sense to me right from the start. Even learning the numerals, how to count, and simple arithmetic, addition, subtraction multiplication, division made sense to me right from the start. It was only later, when they started insisting that there existed a number, distinct from the numeral, that things started not making sense. — Metaphysician Undercover
Ok. I can understand that. But I'm offering you a way out. Just take the position of formalism. There are no numbers, only numerals and the rules for manipulating them. Then you can enjoy the game without reifying it. Like chess. Could that be a philosophical viewpoint that would allow you to get past your current impasse?
I had a similar experience later with physics. We learned basic physics, then we learned about waves, and got to experiment in wave tanks. We learned that waves were an activity within a medium and we were shown through diagrams how the particles of the medium moved to formulate such an activity. All of this made very much sense to me. Then we were shown empirical proof that light existed as waves, and we were told that light waves had no medium. Of course this made no sense to me. — Metaphysician Undercover
It makes no sense to anyone else either. This is well known. Especially in terms of quantum fields being "probability waves." That makes no sense to me. Physics has perhaps lost its way. Many argue so. You and I might well be in agreement on this.
When I learn a game, I must learn the rules before I play. If the rules are such that I have no desire to play the game, I do not play. It's not a question of whether the game makes sense or not, so the analogy is not a good one. — Metaphysician Undercover
Ok. I get that. And I've asked you this many times. You don't want to play the game of math. So then why the energetic objection to it? After all if someone invites me to play Parcheesi and I prefer not to, I don't then go on an anti-Parcheesi crusade to convince the enthusiasts of the game that they are mis-allocating their time on a philosophically wrong pursuit. So there must be more to it than that. With respect to a perfectly harmless pastime like Parcheesi or modern math, one can be for, against, or indifferent. You have explained why you are indifferent; but NOT why you are so vehemently against.
Finally, you decided to address the issue. If there are points distributed on a plane, or 3d space, the positioning of those points relative to each other is describable, therefore there is an inherent order to them. If there was no order their positioning relative to each other could not be described.. — Metaphysician Undercover
Makes no sense. It's perfectly clear that you can order a random assemblage of disordered points any way you like, and that no one order is to be preferred over any other.
You say that they are "randomly distributed", to create the illusion that there is no order. — Metaphysician Undercover
Yes I did say that, and for that reason. We understand each other on this point. The points are placed randomly, so there is no inherent order to their position.
But the fact is that they must have been distributed by some activity, and their positioning posterior to that activity is a reflection of that activity, therefore their positioning is necessarily ordered, by that activity. — Metaphysician Undercover
Well yes, the random number generator I used was actually determined at the moment of the big bang, if one believes in determinism. But you're making a point about randomness, not about the order of the points. You are not persuading me with your claim that a completely random collection of points has an inherent order.
If you think you can interpret the rules as we go, then I'd advise you not to play any games with me. — Metaphysician Undercover
You're flailing.
The inherent order is the exact spatial positioning shown in the diagram. If any point changes location, then the order is broken. Is that so difficult to understand? A spatial ordering is not a matter of first and second, that is a temporal ordering. — Metaphysician Undercover
Ok. Perhaps we can put this one to bed now and meet again some other time. You don't want to read the Wiki piece on order theory. You don't want to learn any math, even for the sake of sharpening your own arguments against it. I do believe we've gone as far as we can here. My conscience is clear as to my having made a good faith effort to inform you as to how mathematicians regard the subject of order. -
 TonesInDeepFreeze
3.8kI learned to play a musical instrument, and it always made sense to me, right from the start. — Metaphysician Undercover
TonesInDeepFreeze
3.8kI learned to play a musical instrument, and it always made sense to me, right from the start. — Metaphysician Undercover
Actually it doesn't make initial sense. Moving from one letter to the next is always a whole step, except from B to C and from E to F. And then double flats move you down a letter except from C to Cbb and from F to Fbb, and double sharps move you up a step except from B to B## and from E to E##.
And on some instruments, when you play at note it's called one particular letter, but on another instrument it's called a different letter, and usually with a flat sign too.
And a 7th chord is not actually the 7th of the scale of the key, but rather it is the minor 7th. But we don't call it a 'minor 7th chord' because that's yet another different chord.
And some intervals in the scale that are not minor nor diminished are called 'major' but others are called 'perfect'.
And a crank (such as you are crank in logic and math) when first confronted with musical notation could come up with nonsense like "a minor second is supposed to be the most dissonant interval, but it's actually two notes that are the closest! It makes no sense!" And the fact that it's enharmonic with an augmented unison. The crank may say, "augmented unison? it's an oxymoron, like empty set!".
And a crank can say, "The major 6th chord is an inversion of a minor 7th chord, so it's two different things! Can't be both major and minor! Doesn't "correspond to reality"! So I'm not going to learn music - it requires that I decieve myself!"
Etc. -
 fishfry
3.4kThat the subject at first makes little sense is probably usually true. And it's true for me for many different subjects. But symbolic logic is one subject that made perfect sense to me immediately. — TonesInDeepFreeze
fishfry
3.4kThat the subject at first makes little sense is probably usually true. And it's true for me for many different subjects. But symbolic logic is one subject that made perfect sense to me immediately. — TonesInDeepFreeze
Me too. But I well remember my experience in abstract algebra. At first it made no sense whatsoever, and it was that way several weeks into the course, until the textbook mentioned a particular example that related to something I was already familiar with. I said, "Ah, this stuff is actually ABOUT something!" That was a great revelation. -
 TonesInDeepFreeze
3.8kIf mathematics requires self-deception, then this does not make sense to me, and so I will not proceed. — Metaphysician Undercover
TonesInDeepFreeze
3.8kIf mathematics requires self-deception, then this does not make sense to me, and so I will not proceed. — Metaphysician Undercover
And we are so lucky that people who did actually go on to learn mathematics were not arrested in development as you are. You wouldn't be typing on your computer or enjoying all the other comforts of science and technology if all the mathematicians dropped out of the subject at the mere suggestion that there is a difference between numbers and numerals. -
 Luke
2.7kWhere are these "numbers" that the teacher kept trying to tell us about, I thought. All I could see is the numerals, and the quantity of objects referred to by the numeral. But the teacher insisted no, the numeral is not the number. So it took me very long to figure out that the numeral was not the "number" which the teacher was talking about, and that the number was just some fictitious thing existing in the teacher's mind, so I shouldn't even bother looking for it because I have to make up that fiction in my own mind, for there to be a number for me to "see". — Metaphysician Undercover
Luke
2.7kWhere are these "numbers" that the teacher kept trying to tell us about, I thought. All I could see is the numerals, and the quantity of objects referred to by the numeral. But the teacher insisted no, the numeral is not the number. So it took me very long to figure out that the numeral was not the "number" which the teacher was talking about, and that the number was just some fictitious thing existing in the teacher's mind, so I shouldn't even bother looking for it because I have to make up that fiction in my own mind, for there to be a number for me to "see". — Metaphysician Undercover
You could see the quantity of objects but not the number of objects?
Even learning the numerals, how to count, and simple arithmetic, addition, subtraction multiplication, division made sense to me right from the start. It was only later, when they started insisting that there existed a number, distinct from the numeral, that things started not making sense. — Metaphysician Undercover
You must have already understood that the number is not the numeral in order to do simple arithmetic. Otherwise, the addition of any two numbers (i.e. numerals) would always equal 2 (numerals).
Welcome to The Philosophy Forum!
Get involved in philosophical discussions about knowledge, truth, language, consciousness, science, politics, religion, logic and mathematics, art, history, and lots more. No ads, no clutter, and very little agreement — just fascinating conversations.
Categories
- Guest category
- Phil. Writing Challenge - June 2025
- The Lounge
- General Philosophy
- Metaphysics & Epistemology
- Philosophy of Mind
- Ethics
- Political Philosophy
- Philosophy of Art
- Logic & Philosophy of Mathematics
- Philosophy of Religion
- Philosophy of Science
- Philosophy of Language
- Interesting Stuff
- Politics and Current Affairs
- Humanities and Social Sciences
- Science and Technology
- Non-English Discussion
- German Discussion
- Spanish Discussion
- Learning Centre
- Resources
- Books and Papers
- Reading groups
- Questions
- Guest Speakers
- David Pearce
- Massimo Pigliucci
- Debates
- Debate Proposals
- Debate Discussion
- Feedback
- Article submissions
- About TPF
- Help
More Discussions
- Other sites we like
- Social media
- Terms of Service
- Sign In
- Created with PlushForums
- © 2025 The Philosophy Forum






